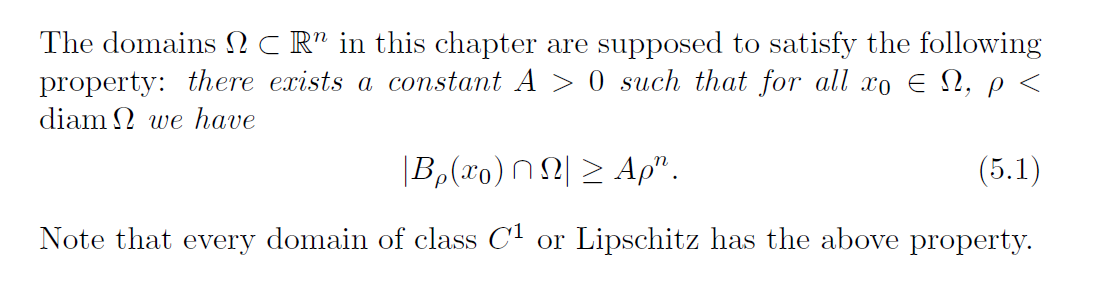In this article and in the book of Ladyzhenskaya et al (1968) - Linear and Quasilinear Elliptic Equations we have the following definition of what is a domain of type (A):
There is no example of a large class that fits into this definition. By $|B_\rho|$ we mean the Lebesgue $N$-dimensional measure of $B_\rho$
My question is the following: A uniform Lipschitz bounded domain (connected, open) domain is a domain of type (A)?
P.S. In the book of Mariano Giaquinta - An introduction to the regularity theory for ellitic systems, harmonic maps and minimal graphs (2012) we have the following information:
Clearly it's not the same of (A) domains but maybe it helps...