In lemma 3.1.5 of this paper I read, there is a fiber sequence of the underlying spaces of simplicial commutative rings $A\stackrel{f}{\longrightarrow} A\rightarrow A/\!\!/f$. Here we define the "derived quotient' $A/\!\!/f$ by the following homotopy pushout diagram: ($f$ is a point in $A$)
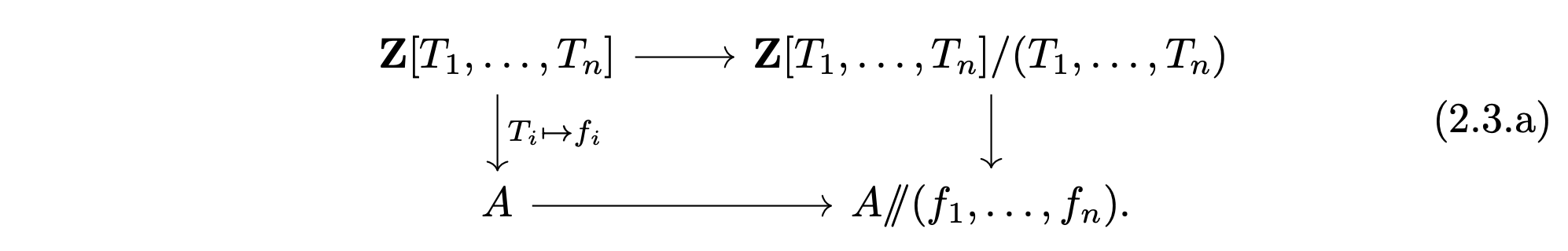 which we can also denote as $A\otimes^{L}_{Z[T] } Z[T]/(T)$.
which we can also denote as $A\otimes^{L}_{Z[T] } Z[T]/(T)$.
I am wondering how we deduce the fiber sequence from the above definition. Here are my thoughts on this:
By the above definition, consider the exact sequence $Z[T]\stackrel{\cdot T}{\longrightarrow} Z[T] \rightarrow Z[T]/(T)$ ( which also is a fiber sequence), after the fully faithful embedding into the category of simplicial ring, which is a right adjoint to $\pi_0$ functor, is again a fiber sequence because right adjoint preserves pullbacks.
if the $\textbf{derived tensor product commutes with fiber sequence in SCRing}$, then from the above sequence we have $A\otimes_{Z[T]}^{L}Z[T]=A\stackrel{\cdot f}{\longrightarrow} A\otimes_{Z[T]}^{L}Z[T]=A\rightarrow A\otimes_{Z[T]}^{L}Z[T]/(T)=A/\!\!/(f)$, which is a fiber sequence in $SCRing$, and geometric realization preserve pullbacks, thus we get the fiber sequences of the underlying spaces.
Here I just take the model category structure on $SCRing=Fun(\triangle^{op},CRing)$ and work with the (homotopy) (co)limits, I understand in many cases we are dealing with the infinity category structure on $SCRing$ as discussed in this post, probably using the infinity structure will be more convenient for the proof of this fiber sequence, any thoughts or comments on this question are very welcome.
Remark: I believe this problem boils down to the commutativity of homotopy limits and colimits, more precisely, pullback of push out is again pullback, sadly I can’t use Mather’s first cubic here because it requires somehow two pullback diagrams.
