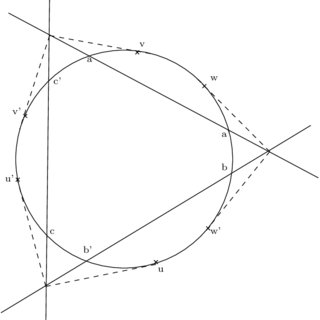
Consider the complex projective plane $P^2$. A rational curve in $P^2$ of degree $\leq 3$ is either a line, a smooth conic, a nodal cubic, or a cuspidal cubic. I am looking for some "nontrivial" configuration of rational curves of degree $\leq 3$ in $P^2$. Here nontrivial means that the existence is not trivial (this is not a mathematically rigorous statement), i.e., we need some calculations to verify whether the configuration really exists.
Here is an example. The figure below is a configuration of four lines and a nodal cubic. The existence can be verified as follows: Let $C:y^2=x^3+x^2$ and $L_1:y=ax$. If $a\neq \pm1$, ±1, then $L_1$ passes through $C$ at a point $p_1$ different from the origin. For $i = 2, 3, 4$, recursively define $L_i$ as the tangent line of $C$ at $p_{i-1}$, and $p_i$ as the another intersection point of $L_i$ and $C$. Then for some suitably chosen $a$, we have $p_1=p_4$, $p_1\neq p_2\neq p_3\neq p_1$. (Explicit calculation is done in https://www.ams.org/journals/proc/2012-140-06/S0002-9939-2011-11038-4/S0002-9939-2011-11038-4.pdf, Section 5.)
P.S. I'm not sure if it makes sense to include enumerative geometry in the tag.