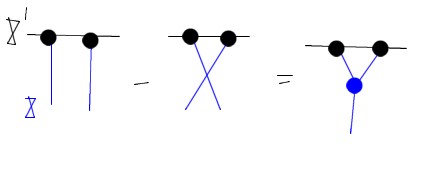0
$\begingroup$

Jacobi

Assume a triad involving the adjoint has multiplicity, e.g. $8\bigotimes 8=1+8+8'+\dots$ in the Lie algebra $SU_3$. How can I handle this in a graphic formalism? Is both $8$ and $8'$ the adjoint, so I just place multiplicity labels $888,888'$ on the dots in the image? Or do I also need a different "color" for $8$ and $8'$ since they are actually different irreps? Or are there even two kind of multiplicities that must be treated differently? (I've heard of "inner" and "outer".)

Improve this question
$\endgroup$
2
  • $\begingroup$ What is the source for the graphical notation you use? The notation may vary significantly between books. $\endgroup$
    – Vladimir Lysikov
    Commented Jul 25 at 8:19
  • $\begingroup$ @VladimirLysikov: My own, although using colored edges for irreps and colored vertices for multiplicity seems natural. (Point is, I've seen the first one over and over, but never the second.) $\endgroup$
    – Hauke Reddmann
    Commented Jul 27 at 16:27

0

Reset to default

You must log in to answer this question.

Browse other questions tagged .