1
$\begingroup$

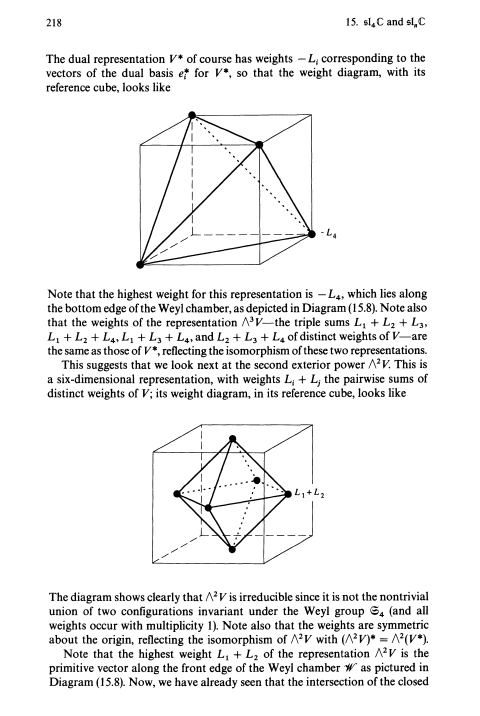
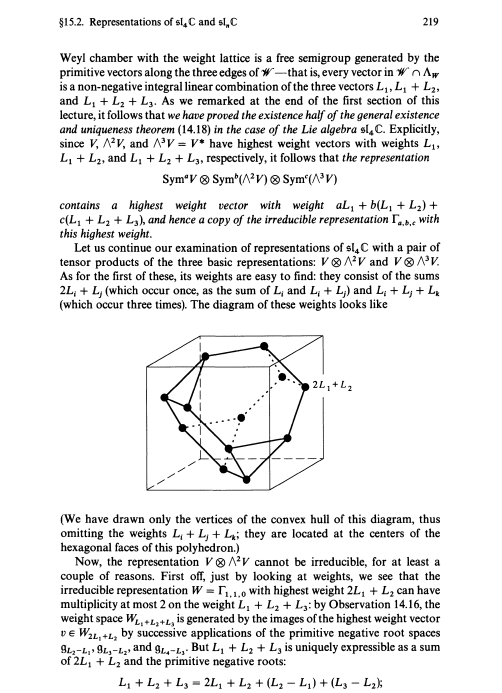
I'm a student studying undergraduate abstract algebra and doing a summer research project in the mathematics department at my school. I'm barely familiar with the rudiments of representation theory; I major in physics and electrical engineering, so my mathematical background is lacking compared to a typical mathematics student. In "Representation theory" by Fulton and Harris, I noticed that Section 15.2 begins with representations of $\mathfrak {sl}_4$($\mathbb{C}$).

enter image description here enter image description here enter image description here enter image description here

I noticed that the weight diagrams of the standard representation of $\mathfrak {sl}_4$($\mathbb{C}$) on $V=\mathbb{C}^4$, the representation $\bigwedge^2V$, and the tensor product representations $V \otimes \bigwedge^2V$ and $V \otimes \bigwedge^3V$ appear to form integral convex polytopes. I want to know if its possible to "embed" these polytopes in an integer lattice such as $\mathbb{Z}^3\subset\mathbb{R}^3$, and consider the Ehrhart polynomials of such polytopes.

How should I establish a coordinate system to even consider convex integral polytopes with the weights acting as vertices in a way that makes sense?

Improve this question
$\endgroup$
2
  • 7
    $\begingroup$ Do you have an advisor/mentor for your research project? They should really be who you are addressing these questions to. (But briefly: there is a lattice here, namely the weight lattice; and considering the Ehrhart polynomial of a weight polytope is an interesting idea, but it is not directly relevant to the representation theory because the weights have multiplicity so e.g. the dimension is not just given by counting lattice points.) $\endgroup$
    – Sam Hopkins
    Commented Jul 9 at 1:33
  • $\begingroup$ @SamHopkins Thank you for this piece of input. I will also ask my advisor. $\endgroup$
    – Caleb Williams
    Commented Jul 9 at 18:31

0

Reset to default

You must log in to answer this question.