Domino equation derivation

Question

Can the functional form of $G$ in the expression $\frac{V}{\sqrt{gH}} = G\left(\frac{d}{H}\right)$ be rigorously derived from first principles, where $V$ is the limiting wave speed of a line of falling dominoes, $H$ is the height of the dominoes, $d$ is the gap between adjacent dominoes, and $g$ is the acceleration due to gravity?

Answer 1

This is a question which my colleague Hans van Leeuwen investigated in much detail in The Domino Effect. There is no closed form solution, and the answer depends on whether or not you want to include the effect of friction. One limit where a simple answer is possible is the limit of infinitely thin dominos without friction, where $G(x)$ starts at $\sqrt{3}=1.7321$ for $x\rightarrow 0$ and decays to $\frac{\sqrt{3}}{2 F\left(\left.\frac{\pi }{4}\right|-2\right)}=1.2641$ as $x\rightarrow 1$. (The function $F$ is the elliptic integral of the first kind.)
The decay is slow, see the plot from Van Leeuwen's paper.

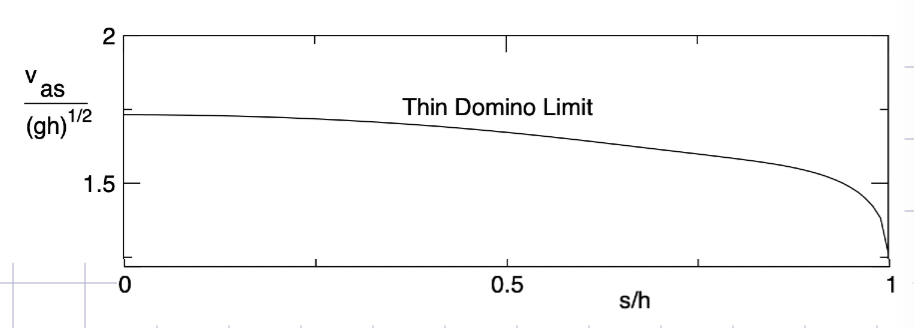
_{Notation: $d$ is the thickness of the domino's, $s+d$ is their separation, and $h$ their height. The plot is for $d\rightarrow 0$.}