Consider the Bolza surface, a compact Riemann surface of genus 2.
It is an octagon in the Poincaire disk model with opposite sides identified.
I would like to write down some analytic expressions for simple smooth functions on the Bolza surface, using coordinates on the Poincaire disk. An example is the constant function $1$, but I would like to have access to non-constant functions. They don't have to be exhaustive (i.e. span the $L^2$ space), but I just want some subset which is easy to manipulate with.
An example of some smooth functions on the Bolza surface are the eigenfunctions of the Laplacian, e.g.:
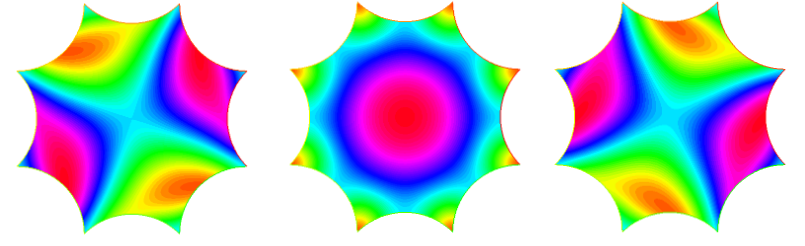but these were found numerically.
I know asking for an analytic expression for these eigenfunctions is probably impossible so that's not what I am going for; but I just want some easy-to-use explicit parameterisation of some set of smooth functions. Thanks!
