2
$\begingroup$

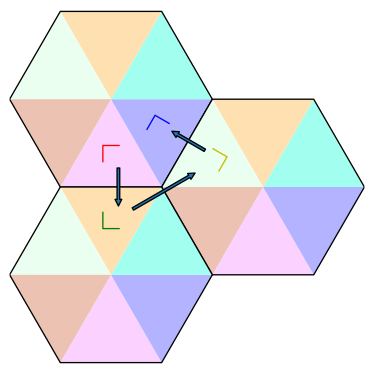
Let $H$ be a regular hexagonal room centered at the origin. Let $W$ be the group generated by reflections about the six sides of $H$. It's well known that $W$ is the affine Weyl group of type $\widetilde{A}_2$. Moreover, $H$ tiles the entire plane under the action of $W$ (though 6 group elements in $W$ can map $H$ to a same room).

Let $H'$ be any regular hexagon room in the tiling, $p$ be a point in $H$, and $q$ be a point in $H'$, then the line segment $pq$ gives an element $w\in W$ such that $wH=H'$. This is because, suppose the line segment $pq$ crosses sequentially through the walls $l_1,\dotsc,l_m$ in the tiling, then we can fold $H'$ back to $H$ by successively reflecting $H'$ about $l_m$, then $l_{m-1}$, and so on. In summary, a line segment joining two rooms gives a word $w\in W$ such that $w$ maps one room to the other.

However, not every $w$ such that $wH=H'$ can correspond to a line segment joining $H$ and $H'$ in the way decribed above. For example, if we reflect $H$ around one of its corners three times, we will get a word $w\in W$ that maps $H$ to itself. But such $w$ certainly does not correspond to a segment that joins $H$ to itself.

Illustration of three such reflections

My question is, what is the necessary and sufficient condition for $w \in W$ to correspond to a line segment connecting $H$ and $wH$?

Improve this question
$\endgroup$
5
  • $\begingroup$ In your picture, is there a reason that one of the three arrows goes through a vertex, rather than the midpoint of a wall? $\endgroup$
    – LSpice
    Commented Jun 11 at 2:20
  • 1
    $\begingroup$ The positioning of the arrows is determined by the following rule: I put a rotated "L" mark at the center of those triangles, and draw an arrow from a mark to another, if the former is reflected to the latter. So the arrows are not meant to pass a vertex or the middle points of the edges. they just point from marks to marks. $\endgroup$
    – zemora
    Commented Jun 11 at 6:13
  • 2
    $\begingroup$ A related question that has a easier answer and is very Coxeter-theoretically natural: Instead tile the plane with equilateral triangles. The set of reflections in your tiling is the same. Every group element is obtained from a segment, recording which triangle edges are crossed rather that which hexagon edges. That is because elements in the group correspond in a natural way to the triangles. (Declare one triangle $T$ to correspond to the identity element. Every other triangle is $wT$ for a unique element of the group.) It may be that the triangles are relevant to your question. $\endgroup$
    – Nathan Reading
    Commented Jun 11 at 13:07
  • $\begingroup$ Yes, the situation with the triangular grid issimpler. Furthermore, any line segment connecting two given rooms gives a reduced representation of $w$, because it is a line segment can only cross any hyperplane once. $\endgroup$
    – zemora
    Commented Jun 12 at 2:23
  • $\begingroup$ Precisely how is "a line segment corresponding to a word" (in the generators of the group W) defined? $\endgroup$
    – Daniel Asimov
    Commented Jun 12 at 17:31

0

Reset to default

You must log in to answer this question.