Is the real and imaginary part of the Dirichlet eta function invertible when viewed as single variable function?

Question

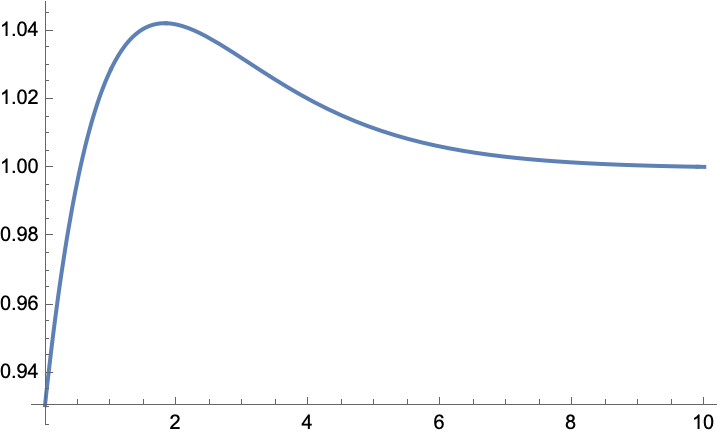
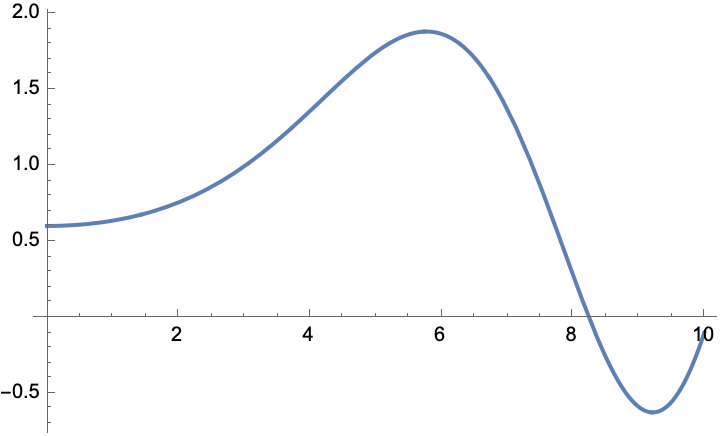
If we examine $\Re(\eta(\alpha + \beta i))$ as a function of $\alpha$ or only $\beta$ is $\eta$ invertible? That is, if we define that map $J:\mathbb{R}\rightarrow \mathbb{R}$ as

\begin{equation}\label{Eq3} J(\alpha)= \sum_{n=1}^{\infty}{\dfrac{(-1)^{n-1}}{n^{\alpha}}\cos(\beta\ln(n))} \end{equation}

for any $\beta\in \mathbb{R}$ is $J$ invertible? Similarly, if we define that map $I:\mathbb{R}\rightarrow \mathbb{R}$ as

\begin{equation}\label{Eq4} I(\beta)= \sum_{n=1}^{\infty}{\dfrac{(-1)^{n-1}}{n^{\alpha}}\cos(\beta\ln(n))} \end{equation}

for any $\alpha\in (0,1)$ is $I$ invertible? My thought was maybe we can invert it but not as a multivariable function and examine each piece separately.

Answer 1

Here are plots of $J(\alpha)$ for $\beta=3$ (left plot) and of $I(\beta)$ for $\alpha=1/2$ (right plot), as you can see these are not invertible functions.