2
$\begingroup$

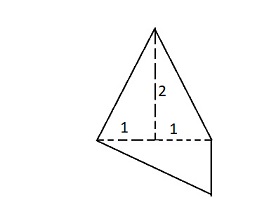
Observations: any thin isosceles triangle has exactly 1 partition into 2 congruent pieces - only 1 line, bisector of its apex, does it.

By attaching a right triangle with base 1 and altitude 2 to an isosceles triangle of base 2 and altitude 2 as below,

enter image description here

we can form a quadrilateral that can be cut into 3 convex congruent pieces as shown. The quad seems to have no other set of such cutting segments that is either disjoint totally to this set of cut segments or has a partial intersection with that set.

  • For which values of n, the number of pieces, greater than 2, can one form convex polygonal regions which can be cut into n convex and mutually congruent pieces by exactly one set of cut lines? There should be no other set of cut lines that is disjoint to or has even a partial intersection with the one set.
Improve this question
$\endgroup$
5
  • $\begingroup$ Is there any way to cut a triangle into four convex and mutually congruent pieces, other than by cuts connecting midpoints of the sides? There is another way, if the triangle is a right triangle, but in other cases? $\endgroup$
    – Gerry Myerson
    Commented May 24 at 2:37
  • $\begingroup$ i can't readily see how there are two ways to cut a right triangle into 4 pieces in two ways. i suspect your comment belongs better with the post: mathoverflow.net/questions/471894/… . there i had mistakenly written that there are 3 obvious ways to cut any triangle into any perfect square number of pieces (m=perfect square, n =3) and that has been edited out now; i see only n =1. thanks. $\endgroup$
    – Nandakumar R
    Commented May 25 at 9:42
  • $\begingroup$ What I had in mind with the right triangle, if you drop perpendiculars to the midpoints of the legs from the midpoint of the hypotenuse, you get two congruent triangles, and a rectangle that can be cut into two further triangles by using either diagonal. But on closer reading of your requirements, I see the two sets of cut lines have a partial intersection with each other, so my construction doesn't count. $\endgroup$
    – Gerry Myerson
    Commented May 25 at 14:39
  • 1
    $\begingroup$ I think a 30-60-90 triangle can be cut into three congruent pieces by cutting along the bisector of the $60$-dgree angle all the way to the opposite side, and then cutting the resulting $120$-degree angle along its bisector all the way to the hypotenuse of the original triangle. I doubt there's any other way to cut that triangle into three congruent pieces. $\endgroup$
    – Gerry Myerson
    Commented May 25 at 14:53
  • 1
    $\begingroup$ That seems one more example - a simpler n nicer one than given in question - of a convex polygon that can be cut into 3 congruent pieces only in one way. Thanks. Further, for an arbitrary triangle, there might be only one partition into any perfect square number of congruent pieces; number of pieces =5 could be trickier. $\endgroup$
    – Nandakumar R
    Commented May 25 at 17:05

0

Reset to default

You must log in to answer this question.