Let $(\mathscr V, \otimes, 1, \sigma)$ be a symmetric strict monoidal category whose unit is terminal. Suppose that every object $A$ is equipped with the structure of a cocommutative comonoid $1 \xleftarrow ! A \xrightarrow{\delta_A} A \otimes A$ for which every morphism is a homomorphism.
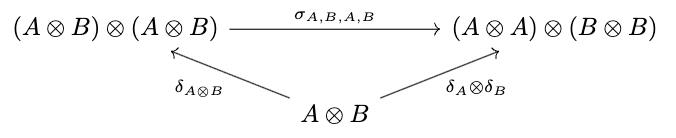
If the following diagram commutes for each pair of objects $A$ and $B$, then $\mathscr V$ is a cartesian monoidal category; this is a variant of Fox's theorem.
Is this condition necessary? In other words, is it possible to find such a $\mathscr V$ for which the diagram above does not commute for all pairs of objects? Or is this condition redundant?
(This is motivated by this question. It is essentially a variant in which we assume much stronger conditions.)