3
$\begingroup$

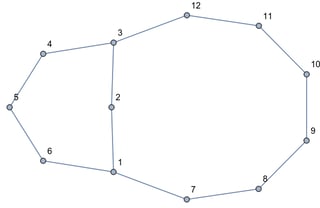
Consider a graph composed of two overlapping cycles: one cycle of length $\ell$ and one cycle of length $m$ where the two cycles share $e$ edges. See picture as an example:

enter image description here

The eigenvalues and eigenvectors are well known for the cases graphs composed of disjoint cycles, as their adjacency matrix reduces to a circulant matrix. 1. Are the eigenvalues known for graphs like the example above?

  1. Consider the matrix resolvent $R(z)$ of the adjacency matrix $A$ defined as $R(z)=(z-A)^{-1}$ for $z\in \mathbb{C}$ with $\rm{Im}(z)\neq 0$. Is the resolvent known for such graphs?
Improve this question
$\endgroup$
3
  • 3
    $\begingroup$ These are "theta graphs" and searching on that gives some eigenvalue information. I didn't find the answer to your question but maybe you will. $\endgroup$
    – Brendan McKay
    Commented May 6 at 9:58
  • 1
    $\begingroup$ I could not find the answer either. I think that for arbitrary graphs, the eigenvalues might be difficult to find. However, obtaining the resolvent should not be too hard. (I am trying to look at generalisations of the Kesten-McKay law, so imagine my wonderment when I read your comment!) $\endgroup$
    – papad
    Commented May 8 at 0:57
  • 1
    $\begingroup$ match.pmf.kg.ac.rs/electronic_versions/Match62/n3/… suggests that the general problem is open. Another source with similar scope: math.ipm.ac.ir/~tayfeh-r/papersandpreprints/thetarevised.pdf $\endgroup$
    – Georg Essl
    Commented May 11 at 0:02

0

Reset to default

You must log in to answer this question.

Browse other questions tagged .