I am currently trying to find some field $F$ which includes $\mathbb{R}$ (or $\mathbb{C}$) and in which series $x^* = \sum_{i\in\mathbb{N}} x_i$ converge to some element of the field. (i.e. $x^* \in F$ whenever all $x_i \in F$) The hyper-real numbers seem like a promising candidate.
I am ok with a non-standard definition of $x^*$ just so long as it coincides with the standard definition when all $x_i \in \mathbb{R}$ and the series is convergent in the standard sense on the real numbers.
The answer is strongly negative.
Arbitrary extensions. The first thing to say is that whenever one extends $\newcommand\R{\mathbb{R}}\R$ to a larger ordered field $F$, one has immediately destroyed (except in trivial cases) the convergence of every convergent series and sequence in $\R$. In this sense, your final requirement is impossible.
The reason is that if $F$ properly extends $\R$, then $F$ will be nonarchimedean, and consequently there will be infinitesimals in $F$. But if we have a nontrivial convergent sequence $x_n\to x$ in $\R$, then none of the $x_n$ will penetrate into the infinitesimal neighborhood of $x$ in $F$ and so the sequence is no longer convergent there. Similarly a convergent series like $\sum_n \frac1{2^n}=2$ in $\R$ will not converge in $F$, since the finite partial sums do not get inside the infinitesimal neighborhood of $2$.
Basically, whenever you add a new infinitesimal neighborhood of $0$, you will destroy the convergence of all sequences and series in the prior field.
The hyperreals. Secondly, you mention the hyperreals, but with the hyperreal field the situation is much worse, for there are no nontrivial convergent sequences or series at all.
Usually when people refer to the hyperreals, they intend a certain kind of ordered field that relates to the real field in a certain way. They want the hyperreal field to be nonarchimedean, of course, but more than this, they want it to be what is called $\aleph_1$-saturated. For the order to be saturated in this way means that whenever you have two countable sets of numbers $A,B$, with $A<B$ in the sense that every member of $A$ is smaller than every member of $B$, then there is a member $x$ of the field lying strictly between $$A<x<B.$$ This property holds, for example, if you construct your hyperreal field as an ultrapower of the real field by a nonprincipal ultrafilter on the natural numbers, which is perhaps the most common construction.
But the thing to observe about any $\aleph_1$-saturated ordered field is that: there are no nontrivial convergent sequences or series at all. The main reason is that every countable set of positive numbers is bounded away from zero.
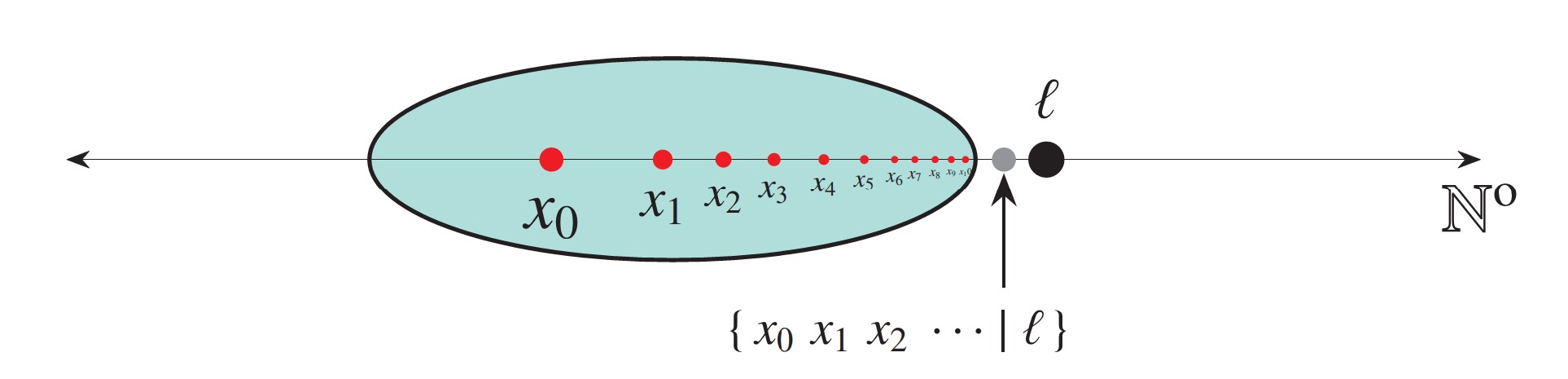
An increasing sequence of field elements $x_n$ for $n\in\mathbb{N}$, for example, cannot converge to a putative limit $\ell$, since the distances from the $x_n$ to $\ell$ will be bounded away from zero, and so there will be field elements closer to to $\ell$ than any $x_n$, which prevents convergence. A similar analysis applies to any nontrivial sequence or series. (The image is from my essay on the Surreal numbers.)
Ultimately, it is precisely the saturation property of the hyperreal field that makes it fundamentally discontinuous, and indeed, an ordered field is $\aleph_1$-saturated if and only if it has no nontrivial convergent sequences, since the latter property implies that every gap is filled. Being merely nonarchimedean is insufficient, since there are nonarchimedean fields with countable cofinality, and in this case there will be many nontrivial convergent sequences.
Nonstandard series. Meanwhile, in a hyperreal field you will have an abundance of nontrivial nonstandard convergent series, where you use a sequence not of type $\mathbb{N}$ but of the nonstandard type $\mathbb{N}^*$. This will be true by the transfer principle, which transfers the truths of such convergence properties from the real field and the standard series to their nonstandard analogues.
For example, although as I have explained the series $\sum_{n\in\mathbb{N}}1/2^n$ does not converge in $\mathbb{R}^*$, nevertheless the nonstandard continuation of it does converge in $\R^*$. $$\sum_{n\in\mathbb{N}^*}\frac1{2^n}=2$$ This nonstandard series has terms for every nonstandard natural number. This is not a series in the usual sense, since it has many more terms beyond $\mathbb{N}$, but it is a nonstandard series, and such series behave in the nonstandard realm very much as the standard series behave in the standard realm.
One way of understanding what has happened is that the standard series does not penetrate the infinitesimal neighborhood of $2$ in $\R^*$, but the nonstandard continuation of the series does make the extra infinitesimal leaps to get inside that neighborhood and thereby converge to $2$.
