15
$\begingroup$

The paper Dividing a polygon into two similar polygons proves that there are only three families of polygons that are irrep-2-tiles (can be subdivided into similar copies of the original).

  • Right angled triangles
  • $1:\sqrt{2}$ parallelograms
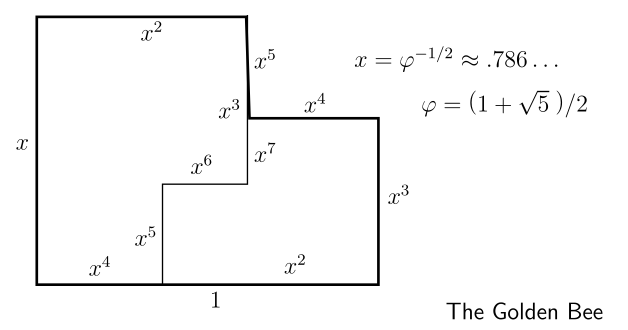
  • The Golden Bee

I wish to find examples of polyhedra that are irrep-2-tiles. The only example I have been able to find is:

  • The $1:2^\frac{1}{3}:2^\frac{2}{3}$ parallelopipeds.

Are there further examples?

This question originates on MathStackExchange.

Diagram of the irregular solution in two dimensions from the linked paper: An irrep-2-tile

I am not sure if this question is difficult or just really, really niche. I've added a bounty which I'll award for any information relevant to the question.

Improve this question
$\endgroup$
0

1 Answer 1

Reset to default
13
+300
$\begingroup$

Definitely not tetrahedra, see this paper. The proof generalizes to other families of polytopes, actually. For example, by Sydler's theorem these cannot be polytopes with nonzero Dehn invariant ($\Leftrightarrow$ not scissor congruent to a cube), see Theorem 16.2 in my book. None of this contradicts some 3-dim analogue of "The Golden Bee", of course.

Improve this answer
$\endgroup$
1
  • 1
    $\begingroup$ Thank you! I'll upvote this answer as soon as I get back the reputation needed to do so. Your book looks like a wonderful resource. I was aware that no tetrahedra is an $n$-reptile for $n\le 7$, but using this together with Dehn invariant is neat. $\endgroup$
    – Kepler's Triangle
    Commented May 8 at 18:20

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .