We define a sequence of graphs $\{B_0, B_1, \dots B_k \dots \}$ as follows:
Consider a seed graph $B$ with a vertex set $V(B) = \{v_1, v_2, \dots v_n\}$ and an edge set $E(B)$. The sequence is constructed iteratively as follows:
- Define the graph $B_0 = (V(B_0), E(B_0))$ where $V(B_0) = \{v_{0,1}, v_{0,2}, \dots v_{0,n}\}$, and $E(B_0) = \{(v_{0,i}, v_{0,j}): (v_i, v_j) \in E(B)\}$.
- For $k = 1, 2 \dots$ define the graph $B_k = (V(B_k), E(B_k))$, such that
\begin{equation}
\begin{split}
& V(B_k) = V(B_{k - 1}) \cup \{v_{k,1}, v_{k,2}, \dots v_{k,n}\}; ~\text{and}\\
& E(B_k) = E(B_{k - 1}) \cup \{(v_{k,i}, v_{k,j}): \text{if}~ (v_i, v_j) \in E(B)\} \cup \{(v_{0,i}, v_{k,i}): i = 1, 2, \dots n\}.
\end{split}
\end{equation}
If the seed graph is $P_2$ we present the first few members of this sequence in the below figure:
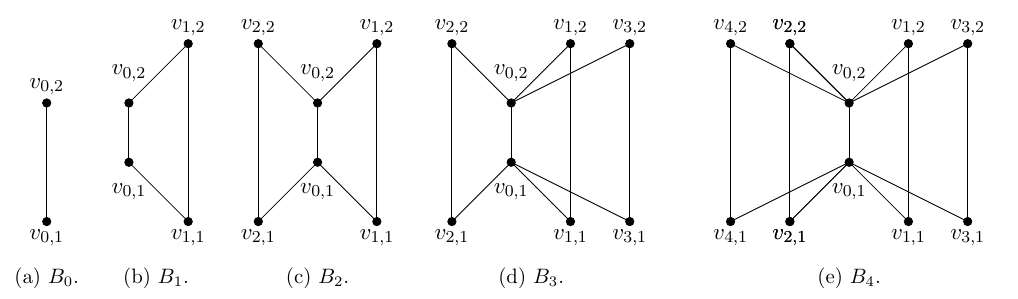
Now I have the following questions:
- Is this graph sequence discused in the literature of graph theory?
- If it is already discussed, what is its standard name?
- If it is already discussed please provide some references.
Thank you.
