Simple proof that certain walks in the plane don't intersect

Question

Suppose that $n$ hamsters are at the points $(1,n)$, $(2,n),\dots, (n,n)$ in the plane. They walk independently one step east with probability $p$ or one step south with probability $1-p$, until reaching the $x$-axis (a point $(k,0)$). It can be shown using symmetric function theory that the probability that their paths don't intersect is $\left(\frac{1-p}{1+p}\right)^{n\choose 2}$. Is there a simple proof? For further information see here.

Answer 1

The non-trivial part of the following argument is due to Fedor Ushakov (for that he gets A at my symmetric functions course).

The hamster from $(i,n)$ goes to south to $(i,i)$ for sure, otherwise their paths intersect. This already has probability $(1-p)^{{n\choose 2}}$.

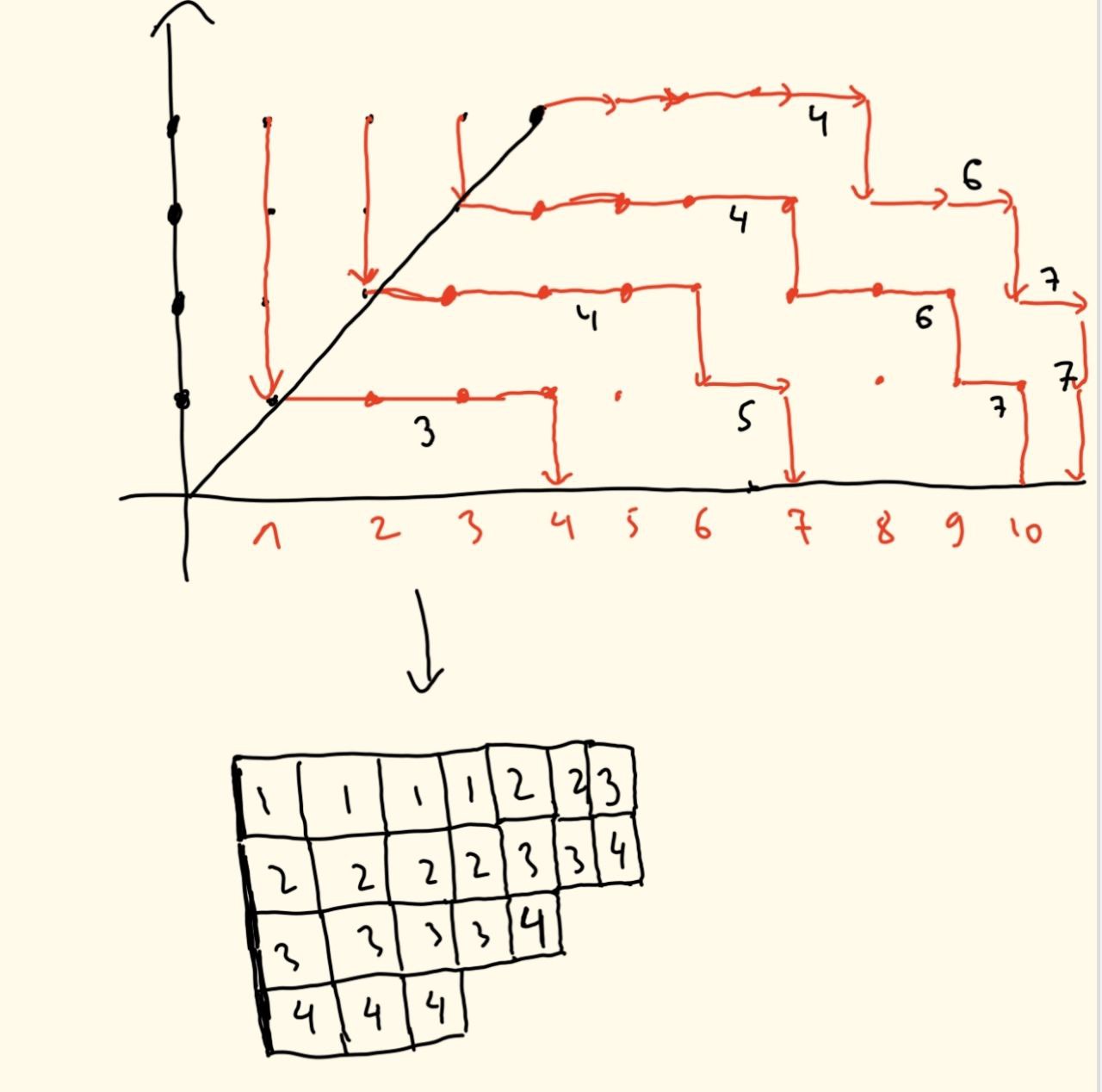
After that we make a semistandard Young tableaux $T$ with at most $n$ rows and entries between 1 and $n$ (denote by $\lambda(T)$ the shape of $T$) from our collection of paths as follows: the hamster who is now at $(i,i)$ is responsible for the $(n+1-i)$-th row (which may be empty): when he makes a move to east being in the horizontal line $(\cdot,j)$, he adds a box with the number $n+1-j$ to this row, see an example.

The hamsters make $|\lambda(T)|$ moves to east and $1+2+\ldots+n=n+{n\choose 2}$ moves to south, thus the probability of this equals $p^{|\lambda(T)|}(1-p)^{n+{n\choose 2}}$. We want to prove that the sum over all Young tableaux $T$ with at most $n$ rows and entries not exceeding $n$ equals $(1+p)^{-{n\choose 2}}$. This is equivalent to the formula $$ \sum_T p^{|\lambda(T)|}=(1-p)^{-n}(1-p^2)^{-{n\choose 2}}. \tag{$\clubsuit$} $$

Note that RHS of $(\clubsuit)$ is the generating function of the sum of elements of symmetric $n\times n$ matrices $A$ with non-negative integer elements: $$ (1-p)^{-n}(1-p^2)^{-{n\choose 2}}=\sum_{A=(a_{ij})}p^{\sum a_{ij}} $$ (each diagonal element corresponds to $(1-p)^{-1}$, each pair of symmetric off-diagonal elements to $(1-p^2)^{-1}$). The bijection from matrices $A$ to semistandard Young tableaux $T$ is provided by RSK.