Consequences of imposing conditions on the restricted Yoneda embedding of a functor

Question

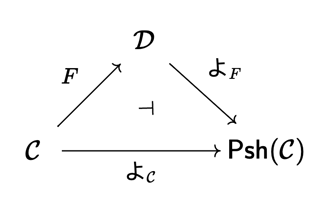
$\newcommand{\op}{\mathsf{op}}\newcommand{\yo}{よ}$Given a functor $F\colon\mathcal{C}\to\mathcal{D}$, the composition $$\mathcal{D}\overset{\yo}{\hookrightarrow}\mathsf{PSh}(\mathcal{D})\xrightarrow{F^*}\mathsf{PSh}(\mathcal{C})$$ is called the restricted Yoneda embedding associated to $F$. Write $\yo_F$ for it.

I'm interested in understanding what commonly imposed conditions on functors, such as essential injectivity, fullness, faithfulness, etc., would imply for $F$ when imposed on $\yo_F$, as well as characterisations of functors $F$ such that $\yo_F$ satisfies a certain condition.

For example, we have the following result for fully faithfulness:

Proposition. The restricted Yoneda embedding $\yo_F$ is fully faithful precisely when $F$ is dense.

Qiaochu Yuan's blog post here has the following result for faithfulness when $F$ is a full subcategory inclusion:

Proposition. The following conditions are equivalent:

1. The restricted Yoneda embedding $\yo_F\colon\mathcal{D}\to\mathsf{PSh}(\mathcal{C})$ is faithful.
2. The functors $\mathrm{Hom}_{\mathcal{C}}(F(A),-)$ are jointly faithful.
3. (If $\mathcal{C}$ has coproducts) For each $D\in\mathrm{Obj}(\mathcal{D})$, the map $$\coprod_{\substack{A\in\mathrm{Obj}(\mathcal{C})\\f\colon F(A)\to D}}F(A)\to D$$ is an epimorphism.

Are there characterisations for when $\yo_F$ is:

1. Faithful;
2. Full;
3. Faithful on isomorphisms;
4. Full on isomorphisms;
5. Pseudomonic;
6. Conservative;
7. Essentially injective?

Moreover, when $\yo_F$ satisfies one of these conditions, what can we deduce for $F$, even if the implication only goes in one direction?

Answer 1

This is not an answer, but some intuition that is too long for a comment.

The defining property of the restricted Yoneda embedding (a.k.a. nerve) is that it is a relative adjoint: specifically, $F : \mathcal C \to \mathcal D$ is left adjoint to $よ_F : \mathcal D \to \mathsf{Psh}(\mathcal C)$ relative to the Yoneda embedding $よ_{\mathcal C} : \mathcal C \to \mathsf{Psh}(\mathcal C)$.

Why is this helpful? Well, very often properties of a left adjoint imply properties of a right adjoint, and vice versa (see here for a list of some such properties). This is also true of relative adjoints (particularly when their roots are dense and fully faithful, which is true of $よ_{\mathcal C}$). For instance:

• Let $J$ be a dense functor. A left $J$-relative adjoint is dense if and only if its right $J$-relative adjoint is fully faithful.

In particular, this recovers the characterisation of full faithfulness of the restricted Yoneda embedding. I suspect any characterisations you find of conditions of the restricted Yoneda embedding in terms of $F$ will apply to relative adjunctions in general. Therefore, you might find it more helpful to start by considering properties of adjunctions, and then seeing to what extent they can be generalised to relative adjunctions.