I have recently watched a seminar about Variational Methods from Mónica Clapp and she gave a very interesting motivation of why the Lusternik–Schnirelmann category (click on the link for the definition of category) arises when one is trying to find critical points of a functional.
The motivation is:
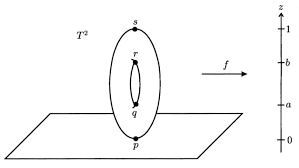
Let $T^2$ be the rotational torus in the position given by the figure and $f:T^2\to\mathbb{R}$ the height function.
Consider $\sigma(x,t)$ be the flux of the ODE $\sigma^\prime=-\nabla f(\sigma)$ with $\sigma(x,0)=x$. Now consider $A_i$, $i=1,2,3,4$, to be open neighborhood ,homeomorphic to discs, of the critical points of $f$ (for example $A_1$ can be a disc that contains the point $s$ on the figure, $A_2$ a disc that contains $r$ and so on) and consider $$B_i:=\left\{x\in T^2: \sigma(x,t_x)\in A_i,\text{for some $t_x$}\right\},$$ Then each $B_i$ is an open, contractible set (I won't prove this) and $T^2=\cup B_i$. This just pictures the fact tha the flow takes any point of the torus and lead to one of the four critical points of $f$.
This then gives us a clue that counting the least number $k$ of open contractible sets $U_i$ such that $T^2=\cup_{i=i}^{k}U_i$, can gives us a hint of the amount of critical points that a function $f$ must have when defined on $T^2$. For example if for a compact 2-manifold $M$ and a function $g: M\to \mathbb{R}$ such a number is $3$ (the category of $M$ is 3), then the function $g$ must have at least $3$ critical points, because if for example $g$ has only 2 critical points, then by defining the sets $B_i$ as we did in the example of the torus we would get two of them, $B_1$ and $B_2$ such that $M=\cup_{i=1}^2 B_{i}$, which contraditcs the fact that $3$ is the least number of open contractibe sets that covers $M$.
So my question is;
- Is there any natural motivation for the Krasnoselskii genus (the genus is defined below)as we just saw for the case of the category? I mean, I would like a motivation directly associated to finding critical points of a function, that arises in a "natural way" (the word natural is subjective here)
Let us define the Krasnoselskii genus:
Let $X\subset \mathbb{R}^N\backslash\left\{0\right\}$ be a symmetric set, i.e. $-x\in X$ for all $x\in X$. We say that a function $h$ is odd if $h(-x)=-h(x)$ ,for all $x$. Then the Krasnoselskii genus of $X$, denoted by $\gamma (X)$ is defined as $$\gamma(X):=\inf\left\{k\in\mathbb{N}\cup\left\{0\right\}:\exists h:X\to\mathbb{R}^k\backslash\left\{0\right\},\text{odd and continuous}\right\}.$$
For the ones that are familiar with variational methods, the genus $\gamma$ is extremely important for finding critical points of an even functional defined a symmetric set. And more than that, it is a well known result that under certain conditions (I will not enter in detail here) we have a very interesting relation with the genus $\gamma$ and the category of a set, namely, denoting $\overline{A}=A/(-x\sim x)$ then the category of $\overline{A}$ equals $\gamma(A)$ (again, I will not enter in detail here, I just want to let you know that I'm aware of such a connection). Moreover I want a motivation for the genus that does not relate to the category in the first moment, as such a mentioned result says.