2
$\begingroup$

Let $M \subset \mathbb{R}^d$ be a manifold with boundary/corners. For example, a piece of curve with endpoints or a $2d$ unit square in $\{ z = 0 \}$. I am interested in introducing local coordinates in the $\varepsilon$-tube defined as $\{ x \colon d ( x, M ) < \varepsilon \}$ around $M$. The tubular neighbourhood theorem gives a way to do so in the vicinity of each inner point of $M$, however the points of $\partial M$ are not covered by this construction.

What I've looked at so far: I've seen the notion of a collar of a manifold, but I don't understand how to use it together with the tubular neighbourhood coordinates. I've also heard of tubular neighbourhood theorem for stratified manifolds, but I couldn't find a good reference. Another idea is to take the tubular neighborhood of some smooth extension of $M$, but I haven't seen any results on such extensions.

So, my question is this: what is the "standard" way of introducing local coordinates on the tubes around manifolds with boundaries/corners?

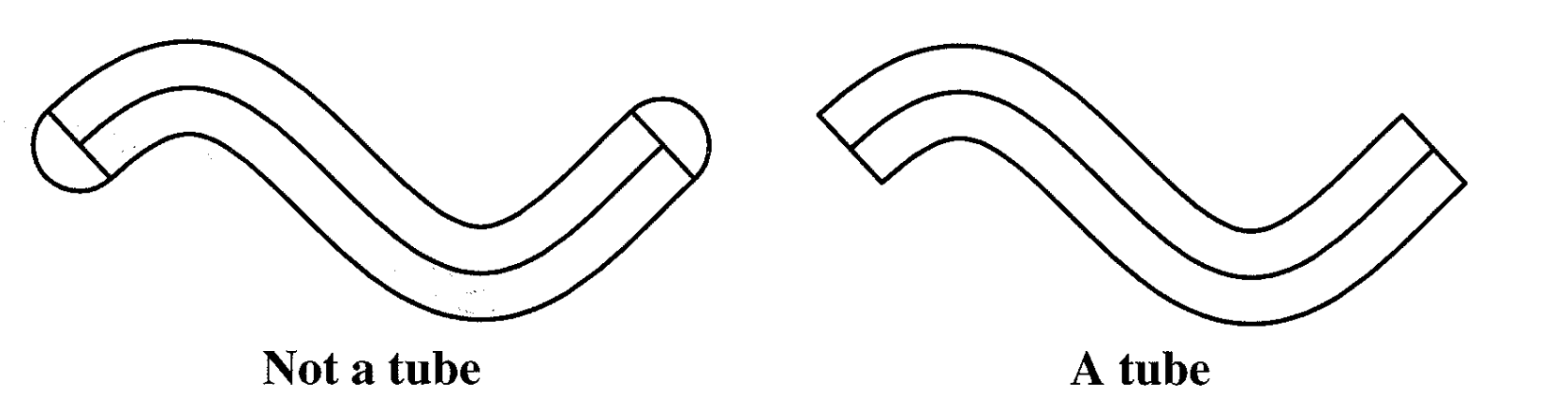
Here's a picture from Alfred Gray's book "Tubes". I am looking for some way to chart the caps (left side of the picture). Tubular neighbourhood theorem gives me a nice fibre bundle structure for the neighbourhood on the right.

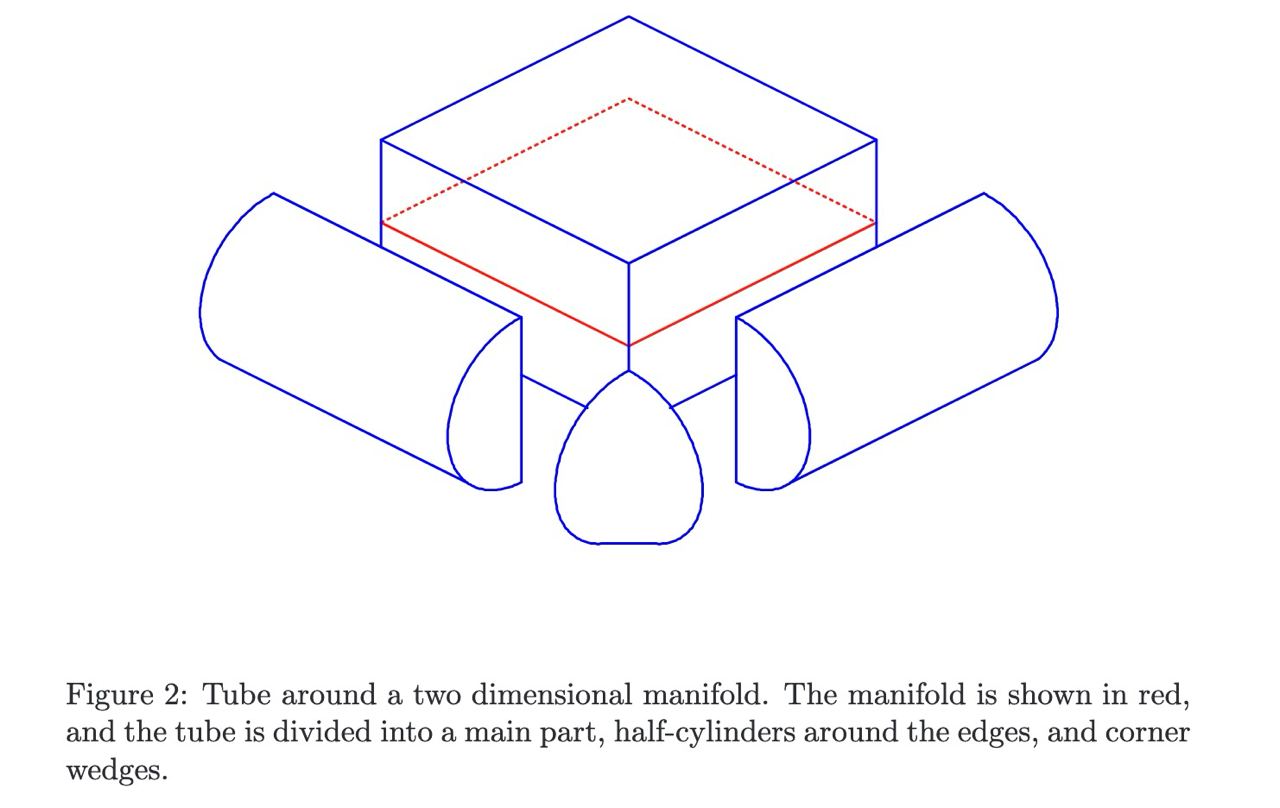
enter image description here

Here's another picture from this paper:

enter image description here

Improve this question
$\endgroup$
15
  • 1
    $\begingroup$ I guess you are aware of the fact that there is no chance for the tubular neighborhood construction working for manifolds with corners in the classical sense for the situation you describe. THe reason is that your manifold is in the thickening not a "neat" submanifold. The situation is discussed in Michors book manifolds of differentiable mappings (available online here: mat.univie.ac.at/~michor/…). The definition can be found in 2.5 and the tubular neighborhood theorem is then following later in the book. $\endgroup$
    – Alexander Schmeding
    Commented Feb 11 at 12:47
  • $\begingroup$ A question I have: What do you mean by "what is the "standard" way of introducing local coordinates on manifolds with boundaries"? Your question is asking for the \epsilon thickening of the manifold and this is something extrinsic after you have a given embedding. $\endgroup$
    – Alexander Schmeding
    Commented Feb 11 at 12:49
  • $\begingroup$ @AlexanderSchmeding, thanks for the reference! Yes, I know that tubular neighbourhood theorem only works for neat submanifolds. I was vague with the use of word "standard", what I mean is: imagine you have a manifold $M$ already embedded into $\mathbb{R}^d$. Then its $\varepsilon$-thickening is well-defined. Then, under some assumptions, there are ways of making this $M_\varepsilon$ into a manifold. If $M$ is neat, then this is done by tubular neighbourhood theorem. Is it possible to do something similar if it's not? For example, a piece of smooth line $\{ x = f(t), \ t \in [0, 1] \}$. $\endgroup$
    – tsnao
    Commented Feb 11 at 13:02
  • $\begingroup$ I think you have something different in mind when writing about the $\varepsilon$ thickening as a manifold. Since this will always be an open subset of euclidean space it has a trivial manifold structure, but arguably that is not the one you are after? $\endgroup$
    – Alexander Schmeding
    Commented Feb 11 at 13:05
  • $\begingroup$ @AlexanderSchmeding, yes, I want a manifold structure compatible with that of $M$. I want my local coordinates on $M_\varepsilon$ to be the local coordinates in $M$ + the normal coordinates. Near the boundary I want to somehow extend the local coordinates of $M$. $\endgroup$
    – tsnao
    Commented Feb 11 at 13:07

1 Answer 1

Reset to default
3
$\begingroup$

From the comments I think the theorem you are looking for is this. I'll be a little fast and loose just to make it easier to state.

Let $M$ be a manifold with corners and $N$ a submanifold, potentially not properly embedded. So $[0,1]^2$ as a subset of $\mathbb R^2$ would qualify, for example, and similarly $[0,1]^2 \times \mathbb R$ as a subset of $\mathbb R^3$ or $[0,1] \times \mathbb R^2$. I suppose this should be called something like semi-properly embedded. We do demand that each connected stratum of $N$ is mapped to just one connected stratum of $M$, as in the above listed examples.

Then there exists an essentially unique regular neighbourhood of $N$ in $M$ and it has a description similar to a stratified space, all definable in terms of the normal bundle of $N$ in $M$, and the stratifications of $M$ and $N$ respectively.

The `top' stratum is the normal bundle of $N$ in $M$.

The co-dimension $1$ stratum consists of the restriction of the normal bundle to $M$ in $N$ to $\partial_1 M \setminus \partial_1 N$, but then you have to take a direct sum with $[0,\infty)$. Here $\partial_1 M$ means the co-dimension one boundary stratum of $M$. Similarly $\partial_2 M$ means the co-dimension two strata, i.e. the traditional `corners', for example $\partial_2 [0,\infty)^2 = \{(0,0)\}$.

The co-dimension 2 stratum of the regular neighbourhood consists of the normal bundle to $M$ in $N$ restricted to $\partial_2 M$, but then you have to take a direct sum with $[0,\infty)^k$ depending on which stratum of $M$ you are in. $k=2$ for the interior stratum, $k=1$ for the co-dimension one stratum and $k=0$ for the co-dimension two stratum.

etc.

These spaces you glue together in the natural way, and you have a corresponding embedding from this total space to the ambient manifold $M$.

I could revise this to be more precise but let me know if that makes sense. It corresponds to your images, but my response deals with some cases you did not include in your images.

Improve this answer
$\endgroup$
4
  • $\begingroup$ This is actually very promising! Do you have a reference for this construction? Somewhere where it's fully described (along with some existence theorem)? $\endgroup$
    – tsnao
    Commented Feb 11 at 22:43
  • $\begingroup$ If you do a little searching you'll find several references for manifolds with cubical corners on this forum. I am not certain if anyone has written down a regular neighbourhood theorem in this level of detail. But the above is the theorem. It's proof would basically be the same as the proof of the tubular neighbourhood theorem, but you'd have to adapt to manifolds with cubical corners. Unfortunately manifolds with cubical corners is one of those topics that gets covered without much fanfare, fairly quietly in non-obvious papers. $\endgroup$
    – Ryan Budney
    Commented Feb 12 at 3:43
  • $\begingroup$ Here are a few threads: mathoverflow.net/questions/387304/… mathoverflow.net/questions/199021/manifold-with-corners mathoverflow.net/questions/103785/… mathoverflow.net/questions/452553/… $\endgroup$
    – Ryan Budney
    Commented Feb 12 at 4:03
  • $\begingroup$ They might have the reference you need. If you don't find it there, you might need to write up the proof yourself. $\endgroup$
    – Ryan Budney
    Commented Feb 12 at 4:15

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .