Has the function $F_s(x)=\sum_{k=0}^{\infty}\frac{x^k}{\Gamma(k+1)^s}$ been studied before?

Question

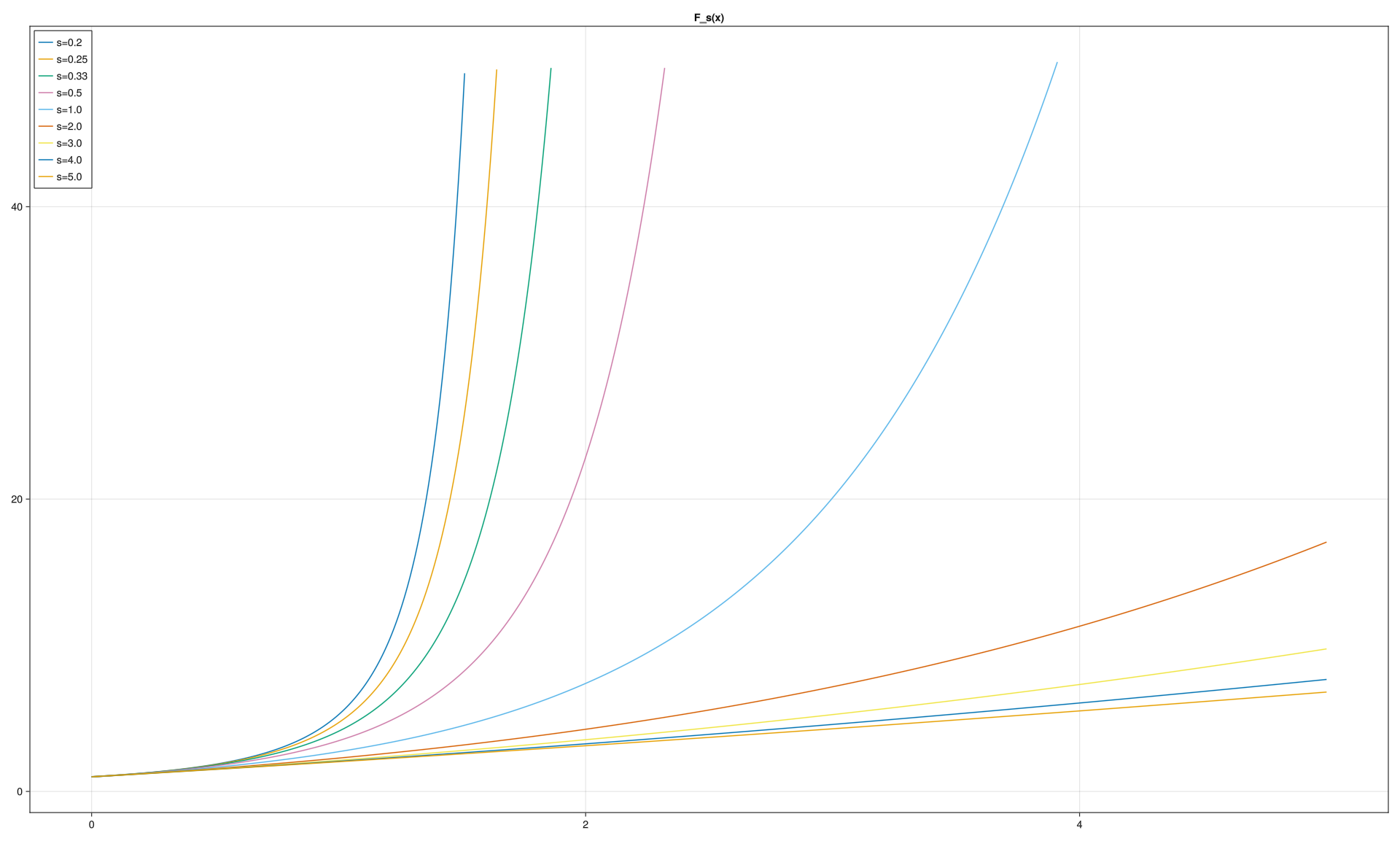
While studying an application of Grönwall's inequality I found that the function $$ F_s(x)=\sum_{k=0}^{\infty}\frac{x^k}{\Gamma(k+1)^s} $$ for $s\geq0$ in some cases provides a sharper bound. I had a quick look at the DLMF but couldn't find anything related.

It is clear that $F_1(x)=e^x$ and for $n\in\mathbb{N}$ it seems that $F_n(x) = {}_{0}F_{n-1}(;1,\ldots,1;x)$ is a generalized hypergeometric function. However, I'm more interested in the case $s\in[0,1]$.

Is this function (and its properties) already known?

Answer 1

Carlo identified it as the normalization constant of the Conway-Maxwell-Poisson distribution (see e.g. Wikipedia.

The first to study this function seems to be

• É. Le Roy: Valeurs asymptotiques de certaines séries procédant suivant les puissances entières et positives d’une variable réelle. (1900)

I also found some more references related to the generalization $$ F_{\alpha,\beta}^{(\gamma)}(z) = \sum_{k=0}^{\infty} \frac{z^k}{\Gamma(\alpha k+\beta)^\gamma}, \qquad z\in\mathbb{C}, \quad \alpha,\beta,\gamma\in\mathbb{C} $$

• S. Gerhold: Asymptotics for a variant of the Mittag–Leffler function. (2012)

  improves Le Roy's result to provide asymptotics for $F_{\alpha,\beta}^{(\gamma)}$

• R. Garra, F. Polito: On some operators involving Hadamard derivatives. (2013)

  they call it the $\alpha$-Mittag-Leffler function and $\alpha L$-exponential function (for $\alpha=\beta=1$) and provide connections with the fractional calculus

• R. Gorenflo, A. A. Kilbas, F. Mainardi, S. Rogosin: Mittag-Leffler functions, Related Topics and Applications. 2nd ed. (2020)

  see Chapter 5.3, they call it Le Roy type function