When is $\Re(\zeta(s)) - \Im(\zeta(s)) = 0 $ for $0<\Re(s)<1$. Here $\zeta$ denotes the Reimann zeta function. Does the solution live on a vertical line? Or is this another coincidence when both $\Re(\zeta(s)) = 0$ and $\Im(\zeta(s))= 0$.
-
5$\begingroup$ The answers to the kinds of question you are asking can be found in Chapter XI Distribution of Values of $\zeta(s)$ of Titchmarsh's Theory of the Riemann Zeta Function. PS not Reimann. $\endgroup$– StoppleCommented Jan 21 at 1:24
-
$\begingroup$ negative ding because i point out the obvious $\endgroup$– MrPieCommented Jan 21 at 1:25
-
6$\begingroup$ Read the whole chapter, including the notes at the end. @Conrad already alluded to this material in his comment on your previous question. $\endgroup$– StoppleCommented Jan 21 at 1:42
-
2$\begingroup$ See comment at the other post but essentially the real and imaginary part are equal very often since the argument is a real continuous function with jumps at zeta zeroes that oscillates a lot so passes often through $\pi/4+2k\pi$ or $3\pi/4+2k\pi$ I would look up Selberg limit theorem and its simpler analogs on $1/2<\sigma<1$ - there is a book by Laurincikas called Limit Theorems for the RZ function which is post Titchmarsh and discusses these things - a lot is known about the distribution of the values of zeta on vertical lines in the critical strip and that book gives a good account $\endgroup$– ConradCommented Jan 21 at 2:36
-
3$\begingroup$ @free_lancer Your formulas for $\Re(\zeta(s))$ and $\Im(\zeta(s))$ are actually formulas for $\Re(\eta(s))$ and $\Im(\eta(s))$ since they don't account for the $\frac{1}{1-2^{1-s}} $ factor in $$\zeta(s)=\frac{1}{1-2^{1-s}} \eta(s)=\frac{1}{1-2^{1-s}} \sum\limits_{n=1}^\infty \frac{(-1)^{n+1}}{n^s}\,,\quad\Re(s)>0.$$ $\endgroup$– Steven ClarkCommented Jan 21 at 19:53
2 Answers
Let me add another illustration (cw): lines are $\Re(\zeta)=\Im(\zeta)$, the critical strip is in gray, the critical line in red, the zeroes in cyan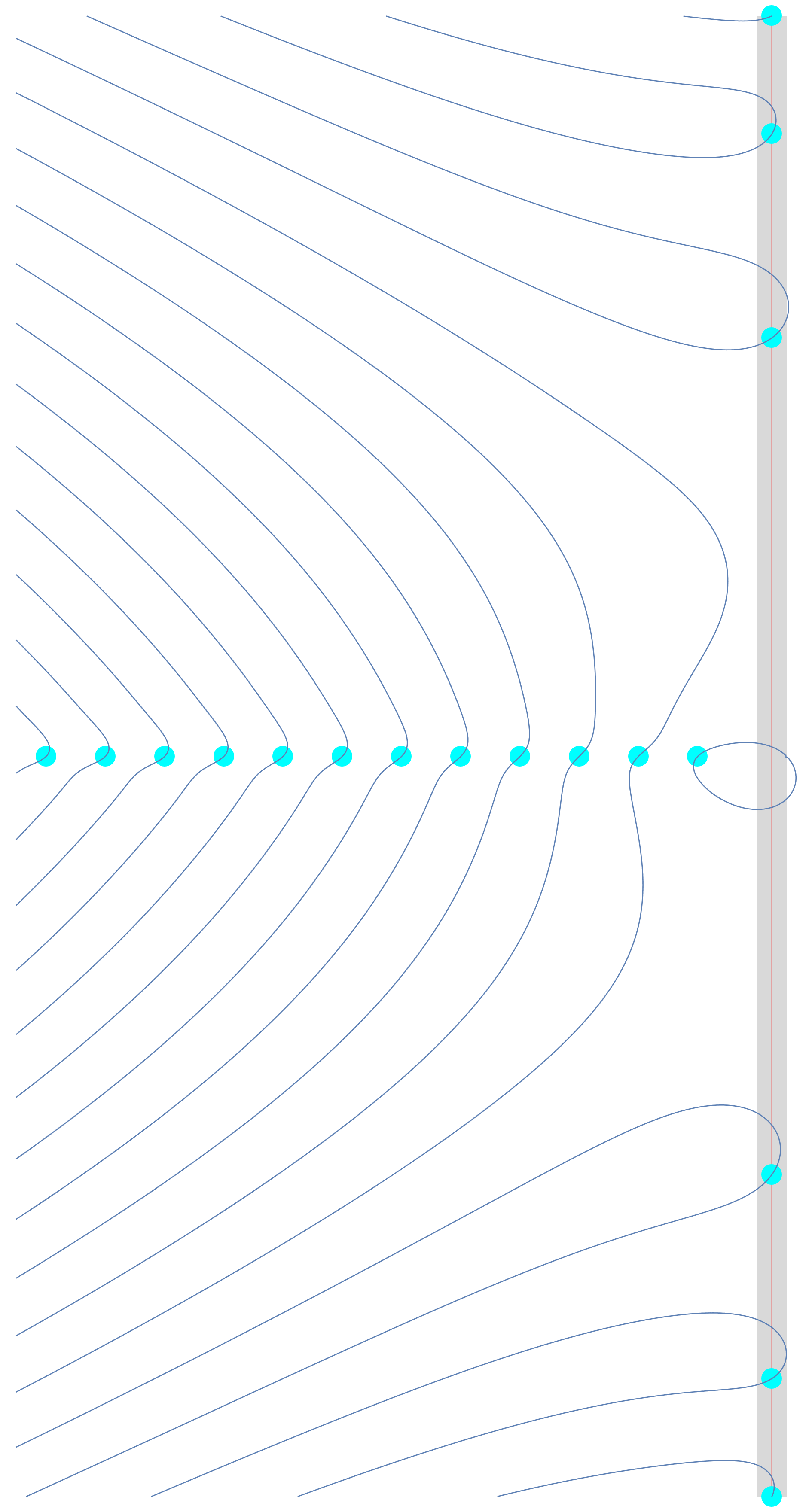
-
-
$\begingroup$ Trivial zeroes at $-2k$, $k\in\mathbb N$, yes. $\endgroup$ Commented Jan 21 at 3:38
-
$\begingroup$ okay sorry i coudlnt see the domain wasnt quite sure. So the zeroes of real and imaginary part are both on the line $1/2$? or at least what it indicates $\endgroup$– MrPieCommented Jan 21 at 3:40
-
$\begingroup$ Simultaneous zeroes of $\Re$ and $\Im$ are exactly zeroes of $\zeta$ if this is what you are asking $\endgroup$ Commented Jan 21 at 3:41
-
$\begingroup$ yes, they both seem to be on the line $1/2$ if I am reading them right. The real and imaginary parts are like a translation of one another. $\endgroup$– MrPieCommented Jan 21 at 3:42
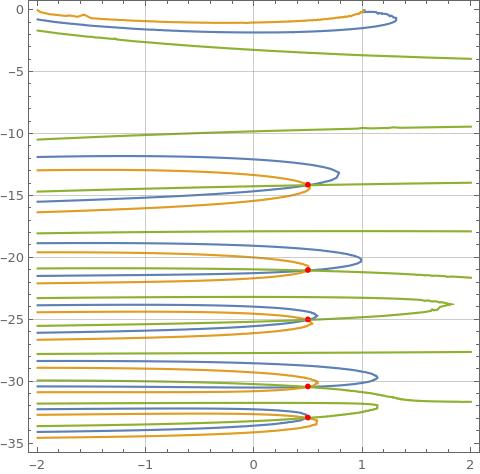
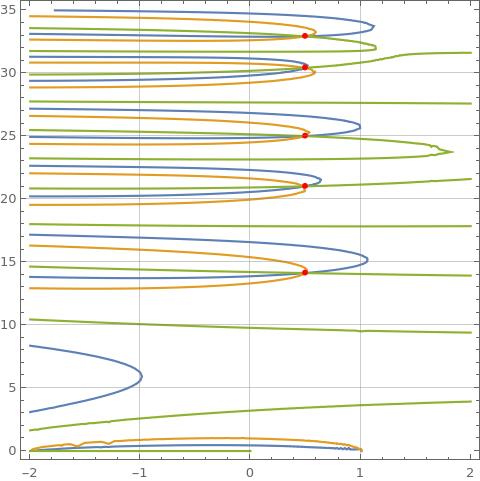
Here are a couple of contour plots of
$$\Re(\zeta(\alpha+i \beta))=\Im(\zeta(\alpha+i \beta))\quad\tag{blue curve}$$
$$\Re(\zeta(\alpha+i \beta))=0\quad\tag{orange curve}$$
$$\Im(\zeta(\alpha+i \beta))=0\quad\tag{green curve}$$
where the red discrete points are the non-trivial zeta zeros.
Note $\Re(\zeta(\alpha+i \beta))=0$ (orange curve) and $\Im(\zeta(\alpha+i \beta))=0$ (green curve) are both symmetric about the real axis whereas $\Re(\zeta(\alpha+i \beta))=\Im(\zeta(\alpha+i \beta))$ (blue curve) is not.
[
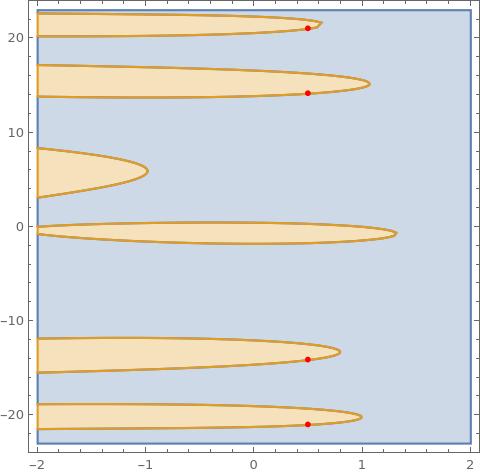
Here's a region plot of
$$\Re(\zeta(\alpha+i \beta))>\Im(\zeta(\alpha +i \beta))\quad\tag{blue region}$$
$$\Re(\zeta (\alpha +i \beta ))<\Im(\zeta (\alpha +i \beta ))\quad\tag{orange region}$$
where the red discrete points are the non-trivial zeta zeros.
Note the boundaries where these two regions meet correspond to the blue curves in the contour plots above.
-
$\begingroup$ And they all magically intersect at these red points. $\endgroup$– MrPieCommented Jan 21 at 3:30
-
$\begingroup$ these plots look like they indicate they only equal each other at the middle of the plane not even the roots. Or am I reading them wrong? $\endgroup$– MrPieCommented Jan 21 at 3:34
-
$\begingroup$ @free_lancer Of course since the nontrivial zeta zeros are the only points in the critical strip which satisfy all three conditions. $\endgroup$ Commented Jan 21 at 3:35
-
-
$\begingroup$ I'm not sure I understand your second and third comments, but the horizontal axis is the real part $\alpha$ and the vertical axis is the imaginary part $i \beta$. I combined a contour plot of the three curves specified in the answer above with a list plot of the zeta zeros which are shown as the red discrete dots. All of the zeta zeros appear on the vertical line corresponding to $\alpha=\frac{1}{2}$. $\endgroup$ Commented Jan 21 at 3:43