Consider $\Omega \subset \mathbb{R}^2$ a convex planar domain having positive curvature on the boundary except for a point $p \in \partial \Omega$ where the curvature vanishes.
I would like to know what happens in this case if I want to apply the Poincaré-Hopf Theorem, which requires a normal pointing outwards on the boundary.
In this case we have a 2-manifold, so we don't have to bother with the $(-1)^n$ term that appears in some generalizations of the theorem for manifolds, since $n = 2$ and our formula becomes that one from the link on wikipedia.
If one had a convex domain having positive curvature everywhere on the boundary, then $$ \sum_i \operatorname{Ind}_{p_i}v = 1 $$ since $\chi(\Omega) = 1.$ If there is a point where the curvature vanishes, $\Omega$ remains convex and this does not change that $\chi(\Omega) = 1.$
However I have not much knowledge on the proof of the Poincaré-Hopf Theorem and how the condition mentioned on the outward normal is crucial and how one could circumvent this and still conclude the formula for the index. A similar discussion is found in Poincare-Hopf theorem for polytopes? and there one of the answers seems to stress that the condition aforementioned is quite important.
Edit: the reference I have for the Poincaré-Hopf Theorem is the following one, which can be found in page 35 of the book Topology from the differentiable viewpoint by John Milnor: 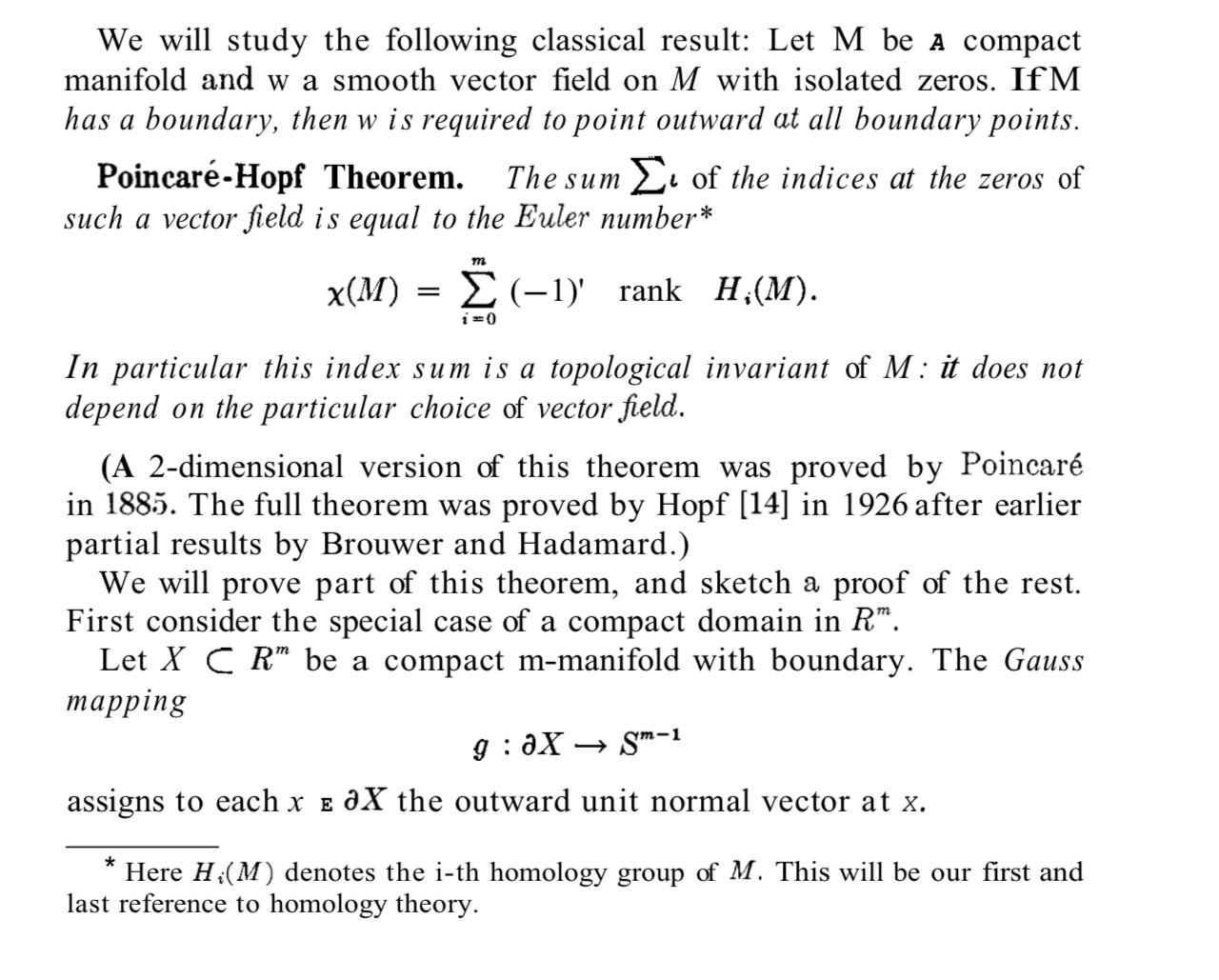
The theorem also works if the manifold is non-compact, which is my case ($\Omega$ is the domain of a PDE actually, so it is an open set). The requirement of the vector field $w$ to point outward everywhere on the boundary is equivalent to $\langle w,\nu \rangle < 0,$ where $\nu$ is the outward normal of the very boundary. At a boundary point where the curvature vanishes, so must $\nu$ vanish. Then how could $\langle w,\nu \rangle $ be anything other than zero there?
