Let $H(z)$ be a continuous solution of the problem $$ H(z)=\frac1{1-z}\int_z^1 \frac{2\zeta}{1+\zeta} H(\zeta^2)\,d\zeta,\ \ \ z\in[0,1);\ \ \ H(1)=1. $$ Is it true that $H(0)=1-\ln2$? The question is directly related to this one https://math.stackexchange.com/questions/4748129/asymptotics-of-sequence-of-rational-numbers .
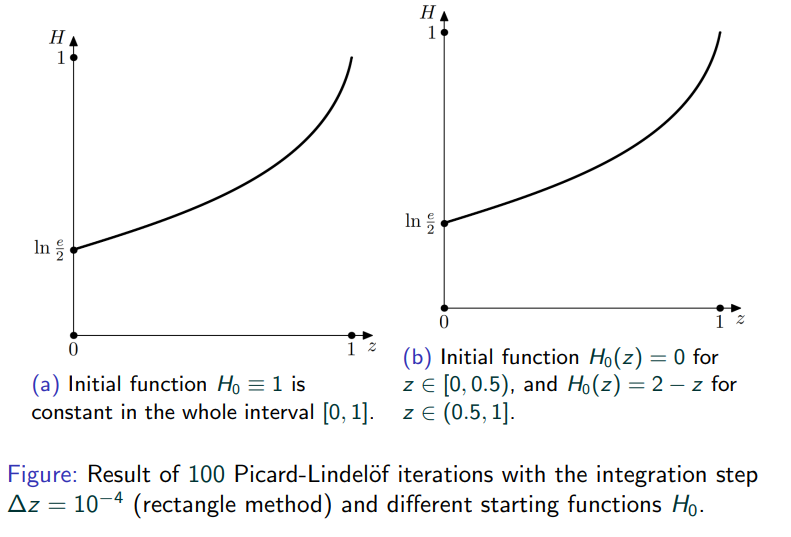
Small update: I did not check all the details, but, it seems that the existence of the solution of this equation can be proved by applying the standard arguments - it is a contraction mapping acting on continuous functions satisfying $H(1)=1$. Hence, the corresponding fixed point (the solution $H(z)$) exists. I already calculated this by using the Picard-Lindelöf scheme
$$
H_{n+1}(z)=\frac1{1-z}\int_z^1 \frac{2\zeta}{1+\zeta} H_n(\zeta^2) \, d\zeta,\ \ \ H_0(z)\equiv1.
$$
In fact, the iterations work well even for discontinuous functions, but the continuity at $z=1$ is required.