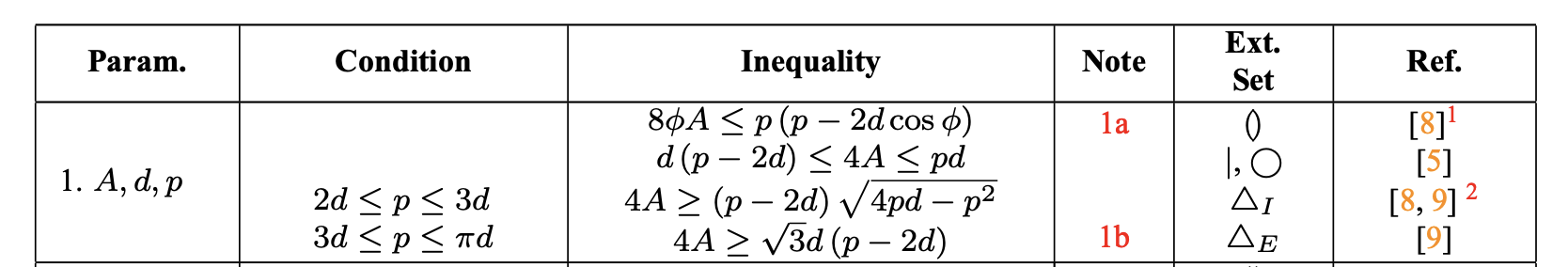2
$\begingroup$

The basic question is to find that planar convex region for which diameter is a minimum when area and perimeter are specified.

A partial answer is given here: http://nandacumar.blogspot.com/2012/11/maximizing-and-minimizing-diameter-ii.html which goes: If the specified perimeter is not more than that of the Reuleaux triangle with the specified area, then, the required planar convex region is a region of constant width. The following question remains, as was noted in the above-linked page:

Question: For specified area $A$ and perimeter $P$ where $P$ is greater than the perimeter of the Reuleaux triangle of area $A$, which planar convex shape minimizes diameter?

When the specified $P$ is steadily increased keeping $A$ fixed, will an ellipse become the answer at any stage?

Note: higher dimensional analogs to the questions could also be considered.

Improve this question
$\endgroup$
0

1 Answer 1

Reset to default
2
$\begingroup$

The 2000 paper Inequalities for Convex Sets, by Paul R. Scott and Poh Way Awyong, lists various inequalities on 2D convex bodies as described here; for your question you can see the state of the research at that time in the first of the three-inequality sections, with some extremal sets listed for their assorted bounds.

enter image description here

Improve this answer
$\endgroup$
1
  • $\begingroup$ Thanks for what appears to be a very comprehensive reference. $\endgroup$
    – Nandakumar R
    Commented Nov 27, 2023 at 10:24

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .