I'm computing the product map $$\mu_2 : CF(L_0,V)\otimes CF(V,L_1)\to CF(L_0,L_1)$$ in Seidel's exact triangle for this specific case:
This is a genus 2 surface, and I color-coded the three (Lagrangian) circles I'm interested in.
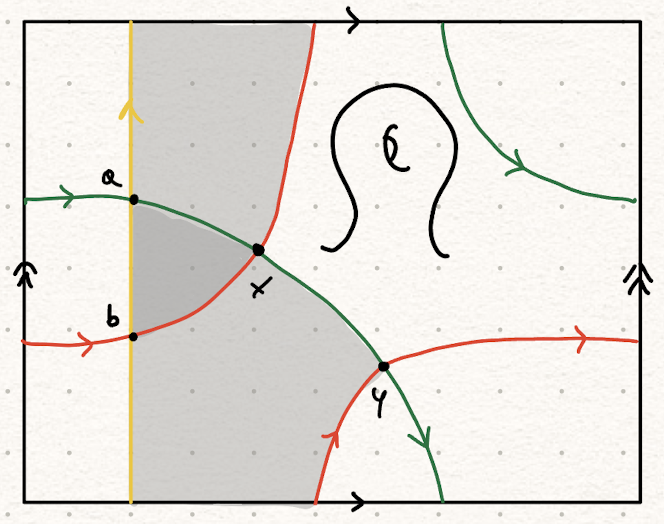
As far as I can see, there are 2 embedded triangles ($T_1,T_2$) with the correct orientation:
and a 3rd one (T3) which is not embedded but looks immersed nonetheless:
whose disk-like "nature" can be better seen by lifting to a double cover of the surface:
My problem with triangle T3 is that it looks rigid since it comes from a rigid one in the cover, but according to a classical theorem from FOOO, such triangles should be in natural bijection with bigons bounded by $L_0$ and $\tau_{V}L_1$ (I believe it's part of the proof that we have a long exact sequence for the Lagrangian surgery $V\# L_1$ - see Auroux's intro's to Fukaya cats page 29). For T1 and T2 their corresponding bigons are immediate to found, while for T3 I cannot.
Should T3 count for the map $\mu_2$? if not, why?