The real projective plane is the space of orientations for "nematic liquid crystals": these are materials (often found in your TV or computer screen!) composed of molecules shaped roughly like rods, which can point in any direction in 3D. However, they have no head or tail, so two antipodal orientations are identified. We can model nematic liquid crystals thus by a map from $U\subset \mathbb{R}^3$ to $\mathbb{RP}^2$.
The topology of the real projective plane thus comes into play when one thinks about "topological defects" in these materials. A topological defect is a sort of singularity, where in some tubular neighborhood of this defect the material is continuous, but at the points of the defect, there is a discontinuity. Furthermore, this defect is topological, in that it cannot be homotoped away locally.
With a bit of oversimplifying, $\pi_1(\mathbb{RP}^2)=\mathbb{Z}_2$ means that there is one nontrivial type of line defect (since $S^1$ surrounds a line) and $\pi_2(\mathbb{RP}^2)=\mathbb{Z}$ means that there are an infinite number of types of point defects in 3 dimensional nematic liquid crystals.
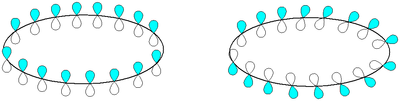
Here's a schematic image of a cross section of a line defect and a corresponding path on $\mathbb{RP}^2$ corresponding to a circuit around it. These are both from Jim Sethna's article "Order Parameters, Broken Symmetry, and Topology":


Here's an old photograph of droplets of nematic liquid crystal between crossed polarizers from the paper P. Poulin, H. Stark, T. C. Lubensky, and D. A. Weitz, Novel Colloidal Interactions in Anisotropic Fluids. J. Science (1997) vol. 275 page 1770.. I won't say too much about the colors, but they correspond roughly to the orientation of the molecule. The sharp points at the center of each droplet are one or more point defects, discontinuities in orientation. The dark brush-like structures coming out of each point are the regions where molecules are oriented in directions parallel to either of the polarizers - thus it's kind of like the inverse image of two different points on $\mathbb{RP}^2$.
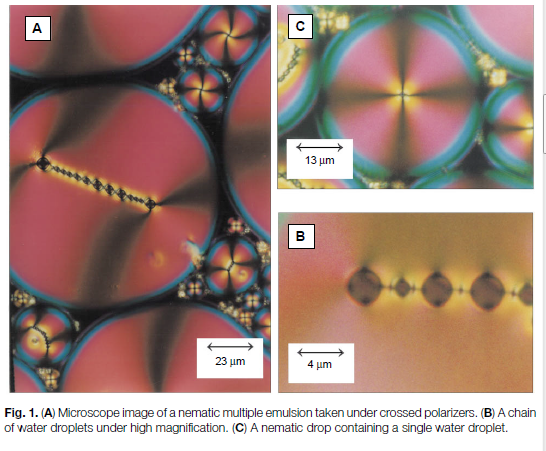
Roughly speaking, a homotopy class of a map from a 1- or 2-sphere to the projective plane being nontrivial, means that the defect cannot be smoothed away (otherwise there would be a homotopy to a constant).
This is part of a much bigger picture of course; and there are other nonorientable spaces that describe the order of materials. I've been vague above because all of this is explained quite beautifully in the article by N.D. Mermin,
The topological theory of defects in ordered media Rev. Mod. Phys. 51, 591–648 (1979). For a quicker introduction, the paper cited above "Order Parameters, Broken Symmetry, and Topology" by Jim Sethna (published in 1991 Lectures in Complex Systems, Eds. L. Nagel and D. Stein, Santa Fe Institute Studies in the Sciences of Complexity, Proc. Vol. XV, Addison-Wesley, 1992) covers the basics.
I love this stuff, so let me know if you have any questions and we can correspond further.