Suppose $(G_n)$ is a sequence of strongly connected directed graphs (without multiple edges) with $G_n$ having $n$ edges such that the adjacency matrix $A_n$ of $G_n$ is primitive, and let $(G_n’)$ be a sequence of subgraphs obtained by deleting a single vertex from $G_n$. Suppose also that the spectral radius of each $G_n$ is bounded below by $1+\epsilon$, and the degree of any vertex is bounded by some constant $d$ (with $d$, $\epsilon$ not depending on $n$). If we let $\rho(G)$ denote the spectral radius of a graph $G$, does it follow that $\rho(G_n) - \rho(G_n’) \rightarrow 0$ as $n \rightarrow \infty$? If so, is there some nice asymptotic for the convergence, say $\rho(G_n) - \rho(G_n’) = O(1/n)$?
It is straightforward that bounds on the spectral radius and degree are each necessary. For the $\epsilon$-condition, one can consider the directed cyclic graphs on $\{1,...,n\}$ with an additional self-loop at $1$; for the bounded vertex condition, one could consider a (two-sided) edge drawn between $1$ and $k$ for each $k \in \{2,...,n\}$.
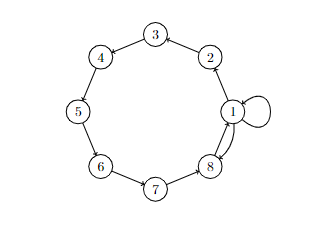
counterexample for $\epsilon$-condition, counterexample for $d$-condition
However, if we have that the spectral radii are bounded away from 1, it follows the "average" number of edges per vertex will be bounded away from 1, and via the degree bound, it follows that deleting a single vertex can only remove an asymptotically small portion of the edges.
It is well-known that $\rho(G_n) > \rho(G_n')$ for each $n$ (by the connectedness property). Additionally, when the graphs are undirected this result is straightforward. For example, it follows from Theorem 1 in this paper, since the size of the i-th component of the principal eigenvector for $G_n$ is proportional to the degree of the corresponding vertex. However, in the directed case I cannot find any results in the literature.
Unfortunately, I am not particularly familiar with the techniques and results in graph perturbation theory (this question came up when considering a certain family of dynamical systems), so I am not sure where to start.
EDIT: The answer is no: one can take any graph with spectral radius strictly greater than one and just attach an arbitrarily long loop. Here is an example where the degree of each vertex is bounded by 2.