I am interested in the affine version of the well-known Hessenberg's Theorem (saying that Pappian projective planes are Desarguesian).
First I introduce all necessary definitions.
Definition L. A linear space is a pair $(X,\mathcal L)$ consisting of a set $X$ and a family $\mathcal L$ of subsets of $X$ satisfying three axioms:
(L1) for any distinct points $x,y\in X$ there exists a unique line $L\in\mathcal L$ containing $x$ and $y$;
(L2) every set $L\in\mathcal L$ contains at least three points;
(L3) $X\notin\mathcal L$.
Elements of the family $\mathcal L$ are called lines.
For any distinct points $x,y\in X$ of a linear space $(X,\mathcal L)$, the unique line $L\in\mathcal L$ containing the points $x,y$ will be denoted by $\overline{xy}$.
Lines $L_1,\dots,L_n$ in a linear space are called concurrent if $\bigcap_{i=1}^n L_i$ is a singleton.
Definition. A linear space $(X,\mathcal L)$ is called
$\bullet$ a projective plane if any distinct lines in $X$ are concurrent;
$\bullet$ an affine plane if for every line $L\in\mathcal L$ and point $x\in X\setminus L$ there exists a unique line $L'\in\mathcal L$ such that $x\in L'$ and $L'\cap L=\emptyset$.
Definition P. A projective plane $(X,\mathcal L)$ is called
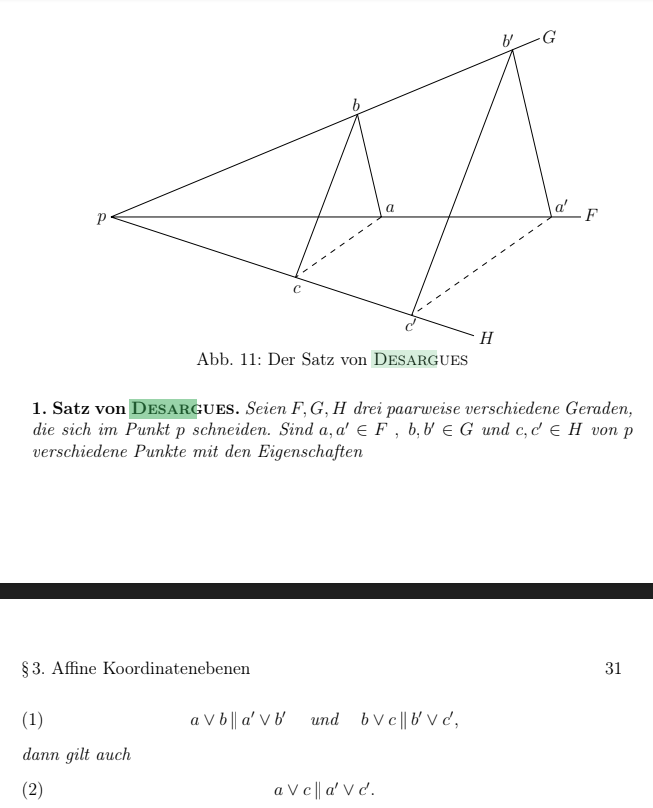
$\bullet$ Desarguesian if for every concurrent lines $A,B,C\in\mathcal L$ and points $a,a'\in A\setminus(B\cup C)$, $b,b'\in B\setminus(A\cup C)$, $c,c'\in C\setminus(A\cup B)$, the set $(\overline{ab}\cap\overline{a'b'})\cup(\overline{ac}\cap\overline{a'c'})\cup(\overline{bc} \cap \overline{b'c'})$ is contained in some line;
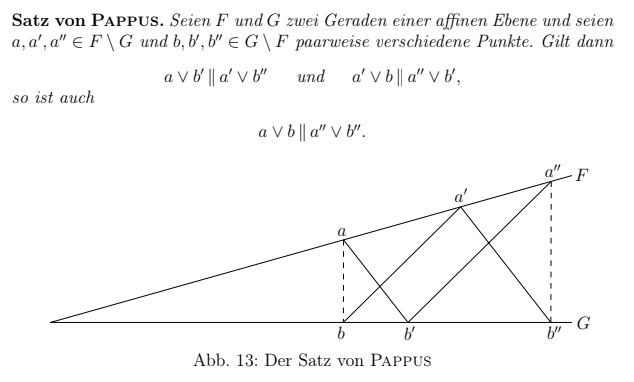
$\bullet$ Pappian if for every lines $L,L'\in\mathcal L$ and distinct points $a,b,c\in L\setminus L'$ and $a',b',c'\in L'\setminus L$, the set $(\overline{ab'}\cap\overline{a'b})\cup(\overline{ac'}\cap\overline{a'c})\cap(\overline{bc'}\cap\overline{b'c})$ is contained in some line.
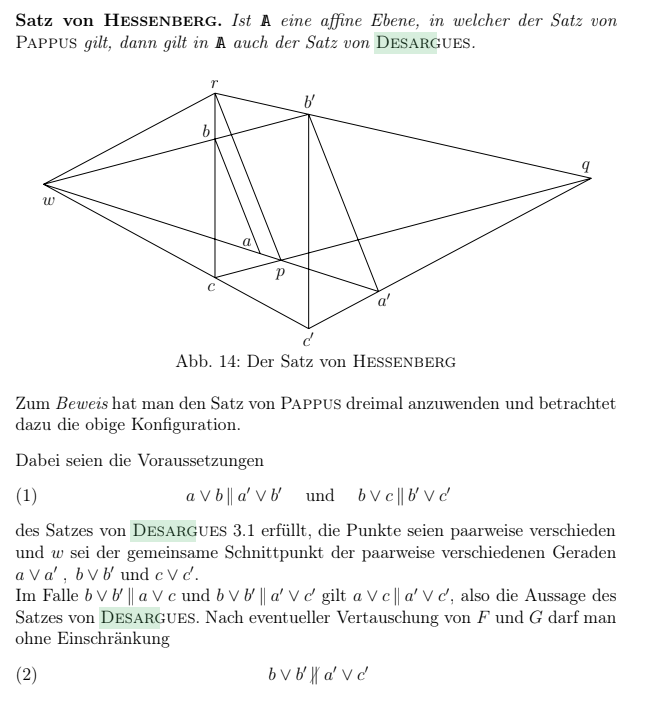
By the famous Hessenberg's Theorem, every Pappian projective plane is Desarguesian.
Now let us define affine counterparts of Desarguesian and Pappian projective planes. Given two lines $L,L'\in\mathcal L$ we write $L\parallel L'$ if $L=L'$ or $L\cap L'=\emptyset$.
Definition A. An affine plane $(X,\mathcal L)$ is called
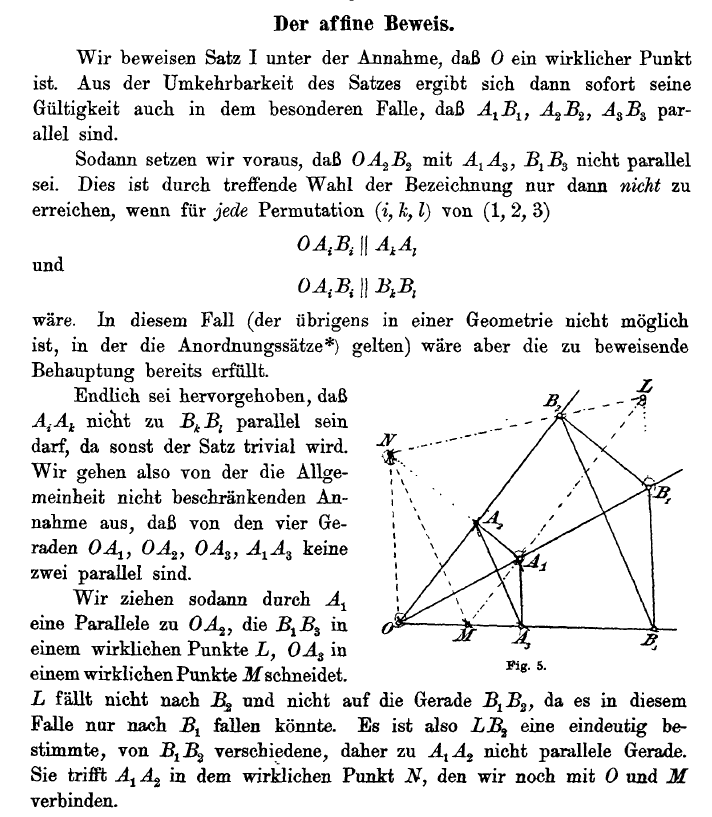
$\bullet$ Desarguesian if for every concurrent lines $A,B,C\in\mathcal L$ and points $a,a'\in A\setminus(B\cup C)$, $b,b'\in B\setminus(A\cup C)$, $c,c'\in C\setminus(A\cup B)$, if $\overline{ab}\parallel\overline{a'b'}$ and $\overline{bc}\parallel\overline{b'c'}$, then $\overline{ac}\parallel \overline{a'c'}$;
$\bullet$ Pappian if for every lines $L,L'\in\mathcal L$ and distinct points $a,b,c\in L\setminus L'$ and $a',b',c'\in L'\setminus L$, if $\overline{ab'}\parallel \overline{a'b}$ and $\overline{bc'}\parallel\overline{b'c}$, then $\overline{ac'}\parallel\overline{a'c}$.
Problem. Is every Pappian affine plane Desarguesian?
Remark. In the lecture notes of Adrien Deloro "Affine and Projective Geometry"
I found Theorem 6.2.1 which contains the proof of Hessenberg's Theorem and also mentions (unfortunately without a convincing proof) that the affine counterpart of the Hessenberg's Theorem also is true. So, what I would like to see is a correct and complete proof of the fact that Pappian affine planes are indeed Desarguesian. Is it published anywhere? If yes, then where exactly?