What is the cardinality of liners of rank 4? Is it always equal 27?

Question

Definition 1. A binary operation $\cdot:X\times X\to X$, $\cdot:(xy)\mapsto xy$, on a set $X$ will be called a line operation if $$xx=x,\quad xy=yx,\quad (xy)x=y$$ for every $x,y\in X$.

Remark 1. Every set $X$ endowed with a line operation is a commutative quasigroup: for every elements $a,b\in X$, the equation $ax=b$ has a unique solution $x=ab$.

Definition 2. A line operation $\cdot:X\times X\to X$ on a set $X$ is called planar if $$x(yz)=(xy)(xz)=z(y(xz)).$$

Example. Every linear space $X$ over a $3$-element field $F$ carries the planar line operation $\cdot:X\times X\to X$ assigning to every points $x,y\in X$ the unique point $xy\in X$ such that the set $\{x,y,xy\}$ coincides with the line $\{x+f(y-x):f\in F\}$ passing through the points $x,y$ in the $F$-linear space $X$.

Definition 3. A set $X$ endowed with a planar line operation $\cdot:X\times X\to X$ will be called a liner.

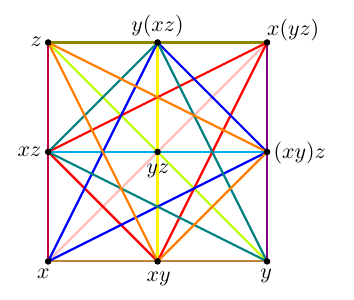
A subset $A\subseteq X$ of a liner $(X,\cdot)$ is called flat if $AA=\{xy:x,y\in A\}\subseteq A$. Flat subsets of liners will be called flats. Since the intersection of any family of flats is flat, for every set $B\subseteq X$, the intersection $\overline B$ of all flat subsets of $X$ that contain $B$ is a flat in $X$, called the flat hull of the set in $B$. For a subset $A$ of a liner $(X,\cdot)$ the rank $r(A)$ is the smallest cardinality of a set $B\subseteq X$ whose flat hull $\overline{B}$ contains the set $A$. Flats of rank 2 and 3 in $X$ are called lines and planes in $X$. It can be shown that every line $L$ in $X$ coincides with the set $\{x,y,x y\}$ for any distinct points $x,y\in L$ and every plane $P$ in $X$ coincides with the 9-element set $$\{x,y,z,xy,xz,yz,(yz)x,(xz)y,(xy)z\}$$for some points $x,y,z\in P$.

Question 1. What is the cardinality of liners of rank 4? Is it always equal to 27?

Question 2. What is the cardinality of the free liner with four generators? Is it infinite?

Question 3. Are liners known in the theory of quasigroups, maybe under a different name? Do they belong to some known and well-studied class of quasigroups?

Remark (added in Edit 10.10.2023): The answers to Questions 1--3 are (well-)known (to specialists). Question 1 has the negative answer: there exists a liner of cardinality 81. It was constructed by Hall in 1960. Question 2 also has (or at least seems to have) a negative answer: the cardinality of the free liner on 4 generators is 81. Question 3 has positive answer: liners indeed are known in the theory of quasigroups and have many different names (not only involutive quandles), in particular Hall Triple Systems. The necessary information on Hall Triple Systems can be found in Section 28 of Handbook of Combinatorial Designs. and also in this survey paper of Lucien Beneteau.

Answer 1

Every liner is an involutory Latin quandle, and we have a characterization of involutory Latin quandles, so we may simply restrict this characterization to liners.

A quandle is an algebra $(X,*,*^{-1})$ where $*,*^{-1}$ are binary operations that satisfy the identities $x*x=x,x*(y*z)=(x*y)*(x*z)$ and $x*^{-1}(x*y)=x*(x*^{-1}y)=y$. A quandle $(X,*,*^{-1})$ where the operations $*$ and $*^{-1}$ are equal is said to be an involutory quandle. If $(X,*,*^{-1})$ is a quandle, then for each $a\in X$, define an operation $R_a:X\rightarrow X$ by letting $R_a(x)=x*a$. If each function $R_a$ is a bijection, then we shall call the quandle $(X,*,*^{-1})$ a Latin quandle. We have a duality between between involutory Latin quandles and uniquely 2-divisible Bruck loops.

Recall that a loop is quasigroup $(X,*,/,\backslash)$ with an identity $1$ where $1*x=x*1=x$. A loop is said to have two sided inverses if for each $x\in X$, there is a (necessarily unique) $x^{-1}$ with $x*x^{-1}=1=x^{-1}*x$. A loop is said to have the automorphic inverse property if it satisfies the identity $(x*y)^{-1}=x^{-1}*y^{-1}$. A loop is said to be a left Bol loop if it satisfies the left Bol identity $x*(y*(x*z))=(x*(y*x))*z$. A left Bruck loop is a left Bol loop with the automorphic inverse property. We say that a binary operation is uniquely 2-divisible if the operation $x\mapsto x*x$ is a bijection.

A finite left Bruck loop is uniquely 2-divisible if and only if it is of odd order.

Theorem: (Kikkawa, Robinson) Let $X$ be a set, and let $e\in X$.

1. If $(X,*)$ is an involutory Latin quandle, then the structure $\Gamma(X,*)$ defined by $\Gamma(X,*)=(X,+)$ where $x+y=(x/e)*(e\backslash y)=(x/e)*(e*y)$ is a uniquely 2-divisible left Bruck loop with identity element $e$.

2. If $(X,+)$ is a uniquely 2-divisible left Bruck loop with identity element $e$, then define $\Delta(X,+)=(X,*)$ defined by $x*y=(x+x)-y=2x-y$ is an involutory Latin quandle.

3. The mappings $\Gamma,\Delta$ are inverses.

A proof of the above result can be found in [1].

By using this duality, the planars are in a one-to-one correspondence with the 2-divisible left Bruck loops with an identity element $e$ which satisfy the identity $(x+x)-((y+y)-z)=(z+z)-((y+y)-((x+x)-z))$ (which can be abbreviated as $2x-(2y-z)=2z-(2y-(2x-z))$) along with the identity $(x+x)-y=(y+y)-x$ (which is $2x-y=2y-x$).

[1]. Enumeration of involutory latin quandles, Bruck loops and commutative automorphic loops of odd prime power order. Izabella Stuhl, Petr Vojtěchovský. 2019