Lower bounding $|1+z+\cdots + z^{n-1}|$ when $z\approx 1$

Question

I am trying to lower bound $|1+z+\cdots + z^{n-1}|$ when $z$ is a complex number close to $1$ (and $n$ is sufficiently large). My main concern occurs in the case $z = 1 + it$, where $t$ is small. In these cases, the terms of the geometric series spiral around the origin, but because $|z| > 1$ these terms do not fully cancel with each other. Naive applications of the Triangle Inequality fail to capture the oscillation of the powers of $z$ around the origin and I'm having trouble accounting for that.

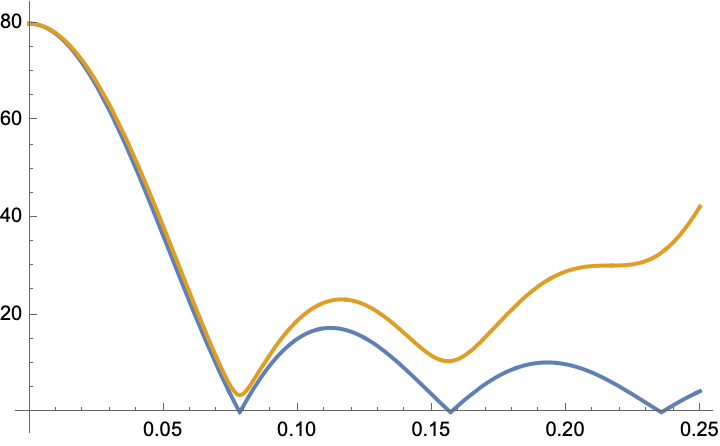
For example, it is tempting to write $$|1 + z + \cdots + z^{n-1}| = \frac{|z^n - 1|}{|z - 1|} \geq \frac{|z|^n - 1}{|z - 1|},$$ but this is too weak when $z$ is small, as the below graph shows
(orange: $t^{-1}|(1+it)^n - 1|$; blue: $t^{-1}(|1+it|^n-1)$, as a function of $t$ for $n=80$)

Are there any known bounds or techniques for dealing with lower bounds for sums of this form? I'm surprised I have not been able to find any yet, though perhaps I am not searching in the right places.

Answer 1

How about $t^{-1}|e^{int}-1|=t^{-1}\sqrt{2-2\cos nt}$? Here is the comparison plot for $n=80$.

Orange: $|1+z+z^2\cdots +z^{n-1}|$ with $z=1+it$ and $n=80$, as a function of $t$;
Blue $t^{-1}|e^{int}-1|$ for $n=80$.

To check that the orange curve indeed lies above the blue curve for small $t$, note that $\lim_{t\rightarrow 0}t^{-4}\bigl(|(1+it)^n-1|^2-|e^{int}-1|^2\bigr)=(6n-5)n^2/12>0$ for $n\geq 1$.

Answer 2

Just a note that Carlo's nice inequality $$ \left|\left(1+\frac{it}{n}\right)^n - 1\right| \geq |e^{it} - 1| \label{1}\tag{1}$$ in fact is valid for all real $t$ and natural numbers $n \geq 1$. For $n=1,2$ this is clear since $e^{it}$ is $1$-Lipschitz and $$\left|\left(1+\frac{it}{2}\right)^2 - 1\right| = \sqrt{t^2 + (t^2/4)^2} \geq |t| = |(1+it)-1|,$$ so suppose that $n \geq 3$. [Note that this calculation shows that the left-hand side of (1) is not always decreasing in $n$, despite converging to the right-hand side in the limit $n \to \infty$.]

Without loss of generality we may take $t$ to be positive. In the limit $n \to \infty$, $\big(1+\frac{it}{n}\big)^n$ converges to $e^{it}$. To try to take advantage of this approximation, we can write $\big(1+\frac{it}{n}\big)^n$ in polar coordinates as $re^{i(t-\varepsilon)}$, where $$ r := \left(1 + \frac{t^2}{n^2}\right)^{n/2}$$ and $$ \varepsilon := t - n \mathrm{arctan} \frac{t}{n}.$$ We would like to use the Taylor approximation $\mathrm{arctan} x \approx x - x^3/3$ for all $x$ (not necessarily close to zero). Indeed, on integrating the inequalities $1-x^2 \leq \frac{1}{1+x^2} \leq 1$ we have $x-\frac{x^3}{3} \leq \mathrm{arctan} x \leq x$ for all $x \geq 0$, thus $$ 0 \leq \varepsilon \leq \frac{t^3}{3n^2} \label{2}\tag{2}.$$ The inequality \eqref{1} squares to $$ r^2 + 1 - 2r \cos(t-\varepsilon) \geq 2 - 2 \cos t.$$ We can rearrange this as $$ (r-1)^2 + 2(r-1) (1 - \cos(t-\varepsilon)) \geq 2 (\cos(t-\varepsilon)-\cos(t)).\label{3} \tag{3}$$ When $t$ is large, $r-1$ is very large, and so we expect the first term on the left-hand side to dominate. Since $$ r-1 = \left(1 + \frac{t^2}{n^2}\right)^{n/2} - 1 \geq \frac{n}{2} \frac{t^2}{n^2} \label{4}\tag{4}$$ and $$ \cos(t-\varepsilon) - \cos(t) \leq \varepsilon \leq \frac{t^3}{3n^2} \label{5}\tag{5}$$ this inequality \eqref{3} is already satisfied (just using the first term on the LHS) if $$ \left(\frac{n}{2} \frac{t^2}{n^2}\right)^2 \geq 2 \frac{t^3}{3n^2}$$ or equivalently if $t \geq 8/3$. Thus we may assume $t < 8/3$. Note that $8/3$ lies between $\pi/2$ and $\pi$, and $\pi/2$ is an inflection point for cosine. In the range $\pi/2 \leq t < 8/3$, $\cos(t) \leq 0$ and we may estimate $$ 1 - \cos(t-\varepsilon) \geq 1 - \varepsilon\geq 1 - \frac{t^3}{3n^2}$$ by \eqref{2}, and so \eqref{3} will be obeyed (just using the second term on the LHS) if $$ 2 \frac{n}{2} \frac{t^2}{n^2} \left( 1 - \frac{t^3}{3n^2} \right) \geq 2 \frac{t^3}{3n^2}$$ which simplifies to $$ \frac{2}{3} t + \frac{t^3}{3n^2} \leq n$$ which one can easily verify for $n \geq 3$ and $t \leq 8/3$.

Finally we need to cover the range $0 < t < \pi/2$, which is the most delicate case (as already illustrated by the numerics). The left-hand side of \eqref{3} decays like $t^4$ as $t \to 0$, so, we need to make sure that our bound on the right-hand side does also; the previous $1$-Lipschitz estimate \eqref{5} will no longer suffice. We rewrite \eqref{2} slightly as $$ (r-1)^2 + 2(r-1) (1 - \cos(t)) \geq 2r (\cos(t-\varepsilon)-\cos(t))$$ and observe from the concavity of cosine in this region that $$ \cos(t-\varepsilon)-\cos(t) \leq \varepsilon \sin(t),$$ gaining us a crucial additional power of $t$ in the limit $t \to 0$ compared to \eqref{5}. Since $$ \frac{1-\cos(t)}{\sin(t)} = \tan\frac{t}{2} \geq \frac{t}{2}$$ it will thus suffice to establish the bound $$ 2(r-1) \frac{t}{2} \geq 2r \varepsilon.$$ From \eqref{2}, \eqref{4}, it would suffice to show that $$ 2 \frac{n}{2} \frac{t^2}{n^2} \frac{t}{2} \geq 2 r \frac{t^3}{3n^2}$$ which simplifies to $$ r \leq \frac{3}{4} n.$$ A key point here is that all the powers of $t$ have canceled out. Now we can bound $$ r \leq \left(1 + \frac{(\pi/2)^2}{n^2}\right)^{n/2} \leq \exp\left( \frac{(\pi/2)^2}{n^2} \frac{n}{2} \right) = \exp\left( \frac{\pi^2}{8n}\right)$$ and the claim easily follows for $n \geq 3$.

Answer 3

This is to complement the inequality $$|(1+it)^n-1|\ge b_1(n,t):=|e^{int}-1|,\tag{10}\label{10}$$ proved by Terry Tao for real $t$ and natural $n$, by the following inequality: $$|(1+it)^n-1|\ge b_2(n,t):=(1+t^2)^{n/2}-1\ge b_3(n,t):=nt^2/2 \tag{20}\label{20}$$ for real $t$ and real $n\ge0$.

For any fixed real $t\ne0$, the lower bound $b_2(n,t)$ on $|(1+it)^n-1|$ grows exponentially in $n$, whereas the lower bound $b_1(n,t)$ remains bounded by $2$. More generally, the lower bound $b_2(n,t)$ (and even the lower bound $b_3(n,t)$) will be better than $b_1(n,t)$ if $nt^2$ is large enough (say if $nt^2>4$).

To prove \eqref{2}, just note that $$|(1+it)^n-1|^2=1 - 2 c (1 + t^2)^{n/2} + (1 + t^2)^n\ge b_2(n,t)^2,$$ where $c:=\cos(n\arctan t)\le1$.

It is now also seen that, for any real $T\ge\tan\dfrac{2\pi}n$, there is some $t\in(0,T]$ such that the lower bound $b_2(n,t)$ on $|(1+it)^n-1|$ is exact, in the sense that $|(1+it)^n-1|=b_2(n,t)$. Actually, the lower bound $b_2(n,t)$ on $|(1+it)^n-1|$ is exact for all $t=\tan\dfrac{2\pi k}n$, where $k=0,1,\dots$.

---

Here are the graphs for $n=100$

• gold $$ \Big\{\Big(t,\dfrac{|(1+it)^n-1|}{b_1(n,t)}\Big)\colon0<t<0.6,b_1(n,t)\ge b_2(n,t)\Big\} $$ and
• blue $$ \Big\{\Big(t,\dfrac{|(1+it)^n-1|}{b_2(n,t)}\Big)\colon0<t<0.6,b_2(n,t)\ge b_1(n,t)\Big\} $$

Answer 4

You could set $z = 1+it=re^{i\theta}$ with $r=(1+t^2)^{1/2}$ and $\theta=\arctan t$ so $$|1 + z + \cdots + z^{n-1}| = \frac{|r^ne^{in\theta} - 1|}{|it|} = \frac{(r^{2n}-2r^n\cos(n\theta)+1)^{1/2}}{|t|}.$$ $$|1 + z + \cdots + z^{n-1}| = \frac{\sqrt{(1+t^2)^n-2(1+t^2)^{n/2}\cos(n\arctan t)+1}}{|t|}.$$