How to bulge out the curved edges of the stereographic tesseract?

Question

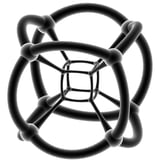
You probably already saw such a representation of the tesseract:

I did something similar on my blog for the truncated tesseract:

The vertices in 3D are the stereographic projections of the original 4D vertices. This point is clear. However I don't know how the varying radius of the bent tubular edges should be chosen. To get a bent 3D edge, I project the corresponding 4D edge on the 3-sphere and then I stereographically project the bent 4D edge. But how to choose the radii? The way I use on my blog consists in arbitrarily taking a radius "proportional" to the norm of the 3D point (e.g. I take $\log\bigl((1 + \lVert M \rVert)/4\bigr)/4$, because I empirically found this choice yields a pretty result). Is there a mathematical consideration that would justify to have a radius "proportional" to the norm of 3D point? What is the meaning of the value of the radius?

Schleimer and Segerman, the authors of the paper Sculptures in $S^3$ (see Figure 3) have a different approach and I don't understand it:

A better solution is to use tubular neighbourhoods in the intermediate $S^3$ geometry. For this we must parameterise the image of such a tube under stereographic projection. Here the circline property is very useful. The boundary of a tubular neighbourhood of a geodesic in $S^3$ can be made as a union of small circles in $\mathbb R^4$. (These circles lie in $S^3$, but are not great.) The small circles map to circlines in $\mathbb R^3$, which can be directly parameterised. Computer visualisation of stereographic projections of 4-polytopes, in this style, are beautifully rendered by the program Jenn3d [8]. In Figure 3 we show four views of a 3D print of the 24–cell, ….

Answer 1

One natural choice (if not necessarily the best choice) is to pick a small $\epsilon$, take the $\epsilon$-neighbourhood in the three-sphere, and stereographically project that to euclidean space. To actually do this requires a bit of spherical trig. Note also that there is no "need" to work in $S^3$. You can instead work with the spherical metric on $\mathbb{R}^3$.