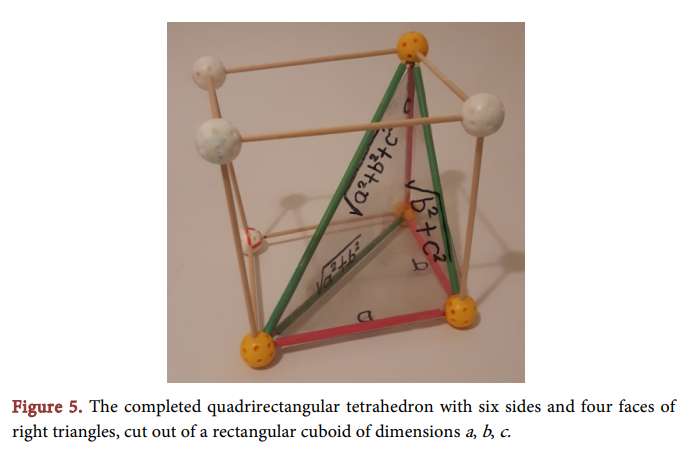
If such a pyramid exists, could someone provide the coordinates of its vertices?
-
$\begingroup$ Here’s a related Concept : en.m.wikipedia.org/wiki/Trirectangular_tetrahedron $\endgroup$– Sidharth GhoshalCommented Sep 26, 2023 at 16:00
-
$\begingroup$ See also math.stackexchange.com/questions/3214139/… $\endgroup$– Marco RipàCommented Sep 26, 2023 at 16:06
-
4$\begingroup$ "All four faces" seems to imply you mean a tetrahedron. If you had said "tetrahedron" rather than "pyramid", this would be clearer. $\endgroup$– Michael HardyCommented Sep 26, 2023 at 23:33
-
$\begingroup$ If three right angles are at one vertex, then you just have the corner of one octant of the usual $(x,y,z)$-Cartesian coordinate system. Are there points $(x>0,0,0),\,\, (0,y>0,0),\,\, (0,0,z>0)$ that are the vertices of a right triangle? No. Now the alternatives are: two right angles and one acute angle at one vertex, or at most one right angle at each vertex. $\endgroup$– Michael HardyCommented Sep 26, 2023 at 23:38
-
2$\begingroup$ en.wikipedia.org/wiki/Schl%C3%A4fli_orthoscheme $\endgroup$– Robin HoustonCommented Sep 27, 2023 at 0:34
3 Answers
If by pyramid, you mean the shape with a square base and four triangular sides, and you want the right angles all where the triangles meet, there is a solution, but it is degenerate.
Sample Vertices: Set A: (0,0,0), (1,0,0), (0,1,0), (-1,0,0), (0,-1,0) Set B: (0,0,0), (1,1,0), (1,-1,0), (-1,-1,0), (-1,1,0)
By degenerate, I mean that all of the vertices are in the same plane (z=0, in my example), and the shape has zero volume. It's flat.
It is obvious that this must be the case. You have four right angles meeting at a point. That's 360 degrees, exactly what you need for them to be in the same plane.
If the first point in my example A was (0,0,1), then all of the edges would be the same length (the square root of 2), so the triangles would all be equilateral--and all of their angles would be 60 degrees. The more you raise that point, the smaller the angles there get.
Giving this StackExchange one last try. In addition to the answer posted with triangular base, here is an example with a square base:
Use two 3-4-5 right triangles and two 3-5-sqrt(34) triangles.