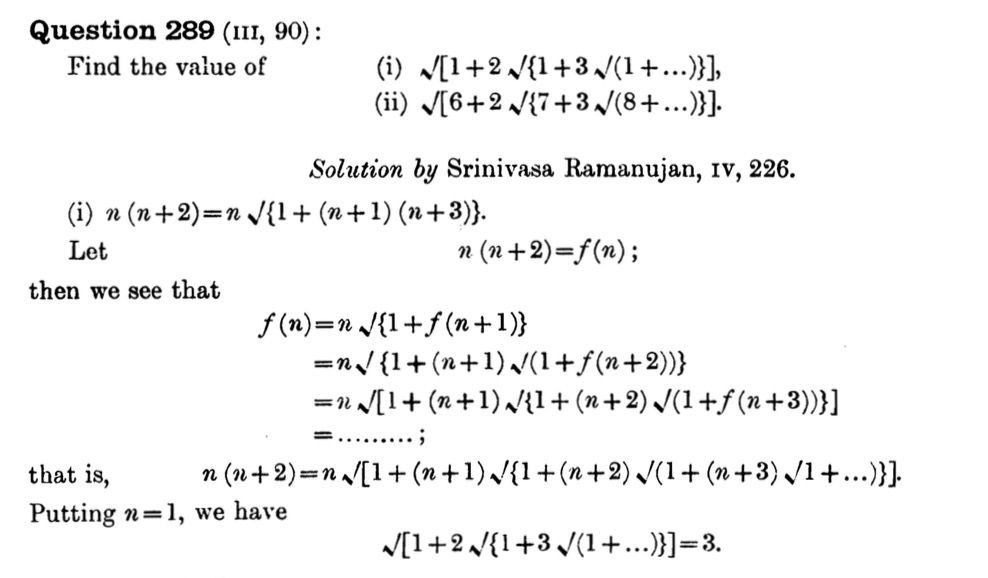Citation for the ill-posed Ramanujan's problem

Question

I've seen many times the following problem posed by Ramanujan: $$\sqrt{1+2{\sqrt{1+3{\sqrt{1+\cdots}}}}} = \mbox{?}$$

This problem is also mentioned on Ramanujan's Wikipedia page along with the proposed solution. The solution looks fine unless you try to formalize the original problem itself. What does '$\cdots$' exactly mean in the statement of the problem? There should be some hidden limit...

I've read some discussion about it (and completely forgot the source) saying that the original statement is meaningless since it allows for many solutions. For example, $$4 = \sqrt{16} = \sqrt{1 + 15} = \sqrt{1 + 2\sqrt{\frac{225}{4}}} = \sqrt{1 + 2\sqrt{1 + 3\sqrt{\frac{48841}{144}}}} = \cdots = \sqrt{1+2{\sqrt{1+3{\sqrt{1+\cdots}}}}}.$$ Who was the first to realize that this problem is ill-posed? Was it published somewhere?

Answer 1

The "incompleteness" of Ramanujan's solution is discussed in On Infinite Radicals by Aaron Herschfeld (1935). A convergence criterion is needed to arrive at the limit 3 (page 420).

Answer 2

I don't think that the problem is ill-posed. To me, the natural way to formalize the problem is to replace the "..." with 0: you don't write anything else. From this, you get

$$\sqrt{1+2}\approx 1.73$$

$$\sqrt{1+2\sqrt{1+3}}\approx 2.23$$

$$\sqrt{1+2\sqrt{1+3\sqrt{1+4}}}\approx 2.56$$

$$\sqrt{1+2\sqrt{1+3\sqrt{1+4\sqrt{1+5}}}}\approx 2.75505326133$$

$$...$$

$$\sqrt{1+2\sqrt{1+3\sqrt{1+4\sqrt{1+5\sqrt{1+7\sqrt{1+8\sqrt{1+9\sqrt{1+10}}}}}}}}\approx 2.998$$

$$...$$

Your example of placing arbitrary numbers in the square root seems to be clearly against the rules. Placing big numbers under the square root can clearly make the sum bigger. If your numbers are bounded then they should have increasingly little effect, and thus should not effect the value of the limit.