17
$\begingroup$

In Hartshorne's Algebraic Geometry Chapter IV, Section 6, he summarizes known results on the existence of smooth space curves of degree $d$ and genus $g$ for $g\le 12$ and $d \le 10$. He shows the following graph

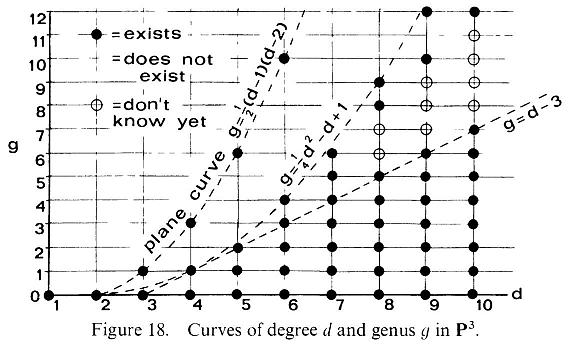
enter image description here

The book was published in the 70's so I was wondering if the existence of some of the unknown cases have already been proven. Are the white dots in the graph still white dots? I tried searching a bit but I do not see anything definite. But perhaps I am not searching correctly. Perhaps someone who is working on this topic could enlighten us.

Improve this question
$\endgroup$
1
  • 4
    $\begingroup$ This is a good question. I asked myself this already many times. In fact, a master student of mine recently checked curves on smooth cubic surfaces and found that some of the "don't know yet": $(d,g)\in \{(8,8),(9,7), (9,8),(10,8), (10,9), (10,10)\}$ actually exist. But this is with very classical tools so I would guess it should be known to experts. $\endgroup$
    – Jérémy Blanc
    Commented Sep 7, 2023 at 19:23

1 Answer 1

Reset to default
9
$\begingroup$

$\newcommand\P{\mathbb P}$Some time ago someone posted an answer to this question and promptly deleted it. I thought that the answer was worth writing. I waited a bit to see if a revised version of this answer would be posted but this never happened. I am not sure why it was deleted as I couldn't find anything wrong with the answer. I will repost the answer with credits to that person (whom I unfortunately do not know):

The paper

On Degrees and Genera of Curves on Smooth Quartic Surfaces in $\P^3$, Mori, Nagoya Math. Journal, Vol 96, p.127-132, 1984.

proves that the curves in the graph associated with the empty "don't know yet" circles do exist. The curves that Mori finds lie on a smooth quartic surface and the condition for this to occur is given in his Theorem 1:

Let $k$ be an algebraically closed field of characteristic $0$ and $d>0$ and $g\ge 0$ be integers. Then there is a non-singular curve $C$ of degree $d$ and genus $g$ on a non-singular quartic surface $X$ in $\P^3$ iff

  • $g=d^2/8 +1$ or
  • $g<d/8$ and $(d,g)\ne (5,3)$

Mori's paper also cite a work of Gruson and Peskin, written in French, where a similar thing was proven except that $X$ is considered to be a singular quartic surface instead. Still it seems that not everything under the curve $g=\frac 14 d^2-d+1$ is known.

Improve this answer
$\endgroup$
1
  • 3
    $\begingroup$ For the benefit of those without sufficient reputation to view deleted answers: there was a similar answer by user inkspot, but with a sketch of a proof instead of a reference (inkspot was not aware of a published proof, but did attribute the result to Mori). Jose Capco added the reference in the comments, and inkspot replied "Thank you. It would be much better to give that reference as an answer so I will delete mine." $\endgroup$
    – R. van Dobben de Bruyn
    Commented Sep 15, 2023 at 19:55

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .