I'm currently self-studying both Von Neumann Set Theory (not ZFC but rather axiomatic set theory with the undefined notion of class) and First-Order Logic.
I've been self-studying the following textbooks for each respectively.
- A Book of Set Theory by Charles C Pinter
- Mathematical Logic by Chiswell
Unfortunately, both implicitly assume a consistent formation of the other leading to potentially circular definitions.
I would like to understand each field of math so that no circular definitions exist.
For example,
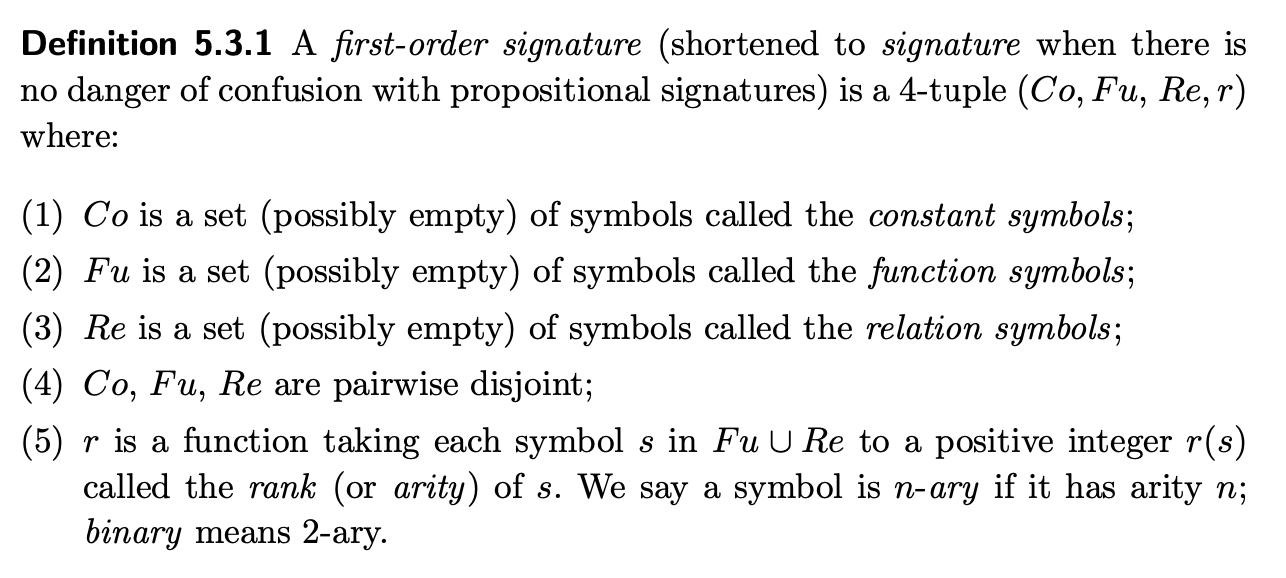
The definition of First-Order Signature $\sigma$ relies upon the definition of Set and consequently Class among other things (which I'll ignore for the sake of argument).
But at this point, one cannot assume there exists a single Class and consequently a single Set. Thus, we cannot assert the existence of even a single variable or constant symbol.
For that, we need an Axiom from Set Theory. For example, the Axiom of Empty Set, which asserts the existence of an "empty set" put precisely as
$$\exists x \forall Y(Y \notin x)$$
However, this assumes we can use variables $x, Y$ in first-order logic but per my previous point, we cannot assert the existence of even a single variable.
Can you please help me unpack this and potentially other circular definitions? Additional resource recommendations are welcome.