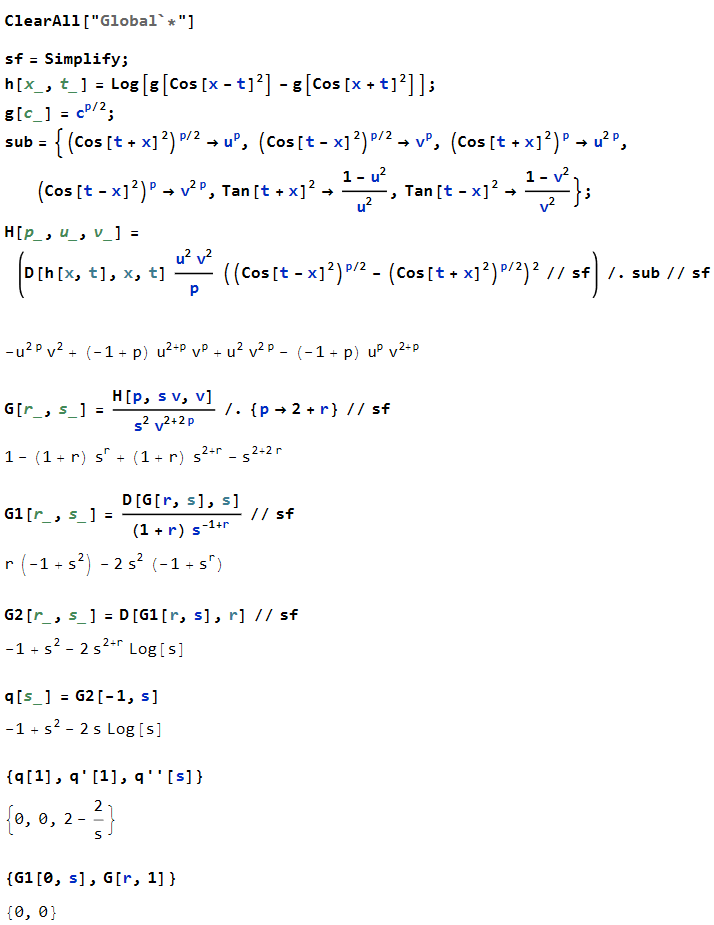Monotone likelihood ratio of densities based on power function

Question

Given $p,\phi,\theta \in \mathbb{R}$ such that $p>2$ and $0 \le \phi,\theta\le \pi/2$ define the density function:

$$f(\phi;\theta) = \mbox{$\Large\frac{1}{p B\big(\hspace{-1pt}\frac{3}{2},\frac{p+1}{2}\hspace{-1pt}\big)}$}\Big(|\cos(\phi-\theta)|^p - |\cos(\phi+\theta)|^p \Big)\hspace{0.5pt} \frac{\sin(2\phi)}{\sin(2\theta)}$$

Show that $f(\phi;\theta)$ has a monotone likelihood ratio. I.e., for $0\le\theta_1 < \theta_2\le\pi/2$, the function:

$$ h(\phi) = \frac{|\cos(\phi-\theta_2)|^p - |\cos(\phi+\theta_2)|^p }{|\cos(\phi-\theta_1)|^p - |\cos(\phi+\theta_1)|^p }$$

is increasing on $[0,\pi/2]$. Equivalently, show that:

$$ \frac{\partial}{\partial \phi} \frac{\partial}{\partial \theta}\hspace{2pt} \log f(\phi;\theta) > 0,\quad 0 \le \phi,\theta\le \pi/2$$

For $1\le p < 2$, the likelihood ratio is decreasing, and the derivative inequality is reversed. But the second partial derivative is $-\infty$ for $\phi=\theta$, which I think will still imply the MLRP, just an infinite slope in the likelihood ratio at one point.

The answer can be for the $1 \le p \le 2$ or $p>2$ case.

This result is important to prove uniqueness of stable optima in the unmixing and deconvolution of linear mixtures of independent random variables with strongly sub- and super-gaussian densities, using variation diminishing property of MLR densities. The result can be proved for $p=4$ by simplifying the derivative expression. This corresponds to using kurtosis as the the cost function, and the uniqueness result is already known in this case. For $p=1$, the likelihood ratio is non-increasing, constant around $\phi=0$ and $\phi=\pi/2$.

Answer 1

This conjecture is true.

Indeed, we have to show that for $x$ and $t$ in $(0,\pi/2)$ we have
\begin{equation} h_2(p;x,t):=\partial_x\partial_t\,\ln(|\cos(x-t)|^p-|\cos(x+t)|^p) \end{equation} is $\ge0$ if $p\ge2$ and $\le0$ if $p\in(1,2]$. (Note that for all $x$ and $t$ in $(0,\pi/2)$ we have $\cos^2(x-t)-\cos^2(x+t)=\sin2x\,\sin2t>0$, so that $h_2(g;x,t)$ is well defined.)

Note that \begin{equation} \begin{aligned} & h_2(p;x,t)\frac{u^2 v^2}p\,(|\cos(x-t)|^p-|\cos(x+t)|^p)^2 \\ & =H(p,u,v):=(p-1) u^{p+2} v^p-v^2 u^{2 p}-(p-1) u^p v^{p+2}+u^2 v^{2 p} \\ & =s^2 v^{2 + 2 p} G(r,s), \end{aligned} \end{equation} where \begin{equation} G(r,s):=(r+1) s^{r+2}-s^{2 r+2}-(r+1) s^r+1, \end{equation} $u:=|\cos(x+t)|$, $v:=|\cos(x-t)|$, $s:=u/v$, and $r:=p-2$, so that $0<u<v\le1$, $s\in(0,1)$, $r\in(-1,0]$ when $p\in(1,2]$ and $r\ge0$ when $p\ge2$.

So, it suffices to prove that, for $s\in(0,1)$,
\begin{equation} G(r,s) \left\{ \begin{aligned} \overset{\text{(?)}}\le0&\text{ if }r\in(-1,0], \\ \overset{\text{(?)}}\ge0&\text{ if }r\ge0. \end{aligned} \right. \tag{10}\label{10} \end{equation}

To prove this, let \begin{equation} G_1(r,s):=\frac{\partial_s G(r,s)}{(1 + r) s^{r-1}} =r (s^2-1)-2 s^2 (s^r-1). \end{equation} Then \begin{equation} G_2(r,s):=\partial_r G_1(r,s)=-2 s^{r+2} \ln s+s^2-1, \end{equation} which clearly decreases in real $r$ for each $s$ ($s\in(0,1)$). So, \begin{equation} G_2(r,s)\le G_2(-1,s)=-2 s \ln s+s^2-1=:q(s). \end{equation} We have $q(1)=0=q'(1)$ and $q''(s)=2-2/s<0$ (as $s\in(0,1)$). So, $q<0$ in $(0,1)$ and hence $G_2(r,s)<0$ (for the relevant $r,s$). So, $G_1(r,s)$ is decreasing in $r$. Also, $G_1(0,s)=0$. So, $G_1(r,s)>0$ for $r\in(-1,0)$ and $G_1(r,s)<0$ for $r>0$.

So, $G(r,s)$ is increasing in $s\in(0,1)$ for each $r\in(-1,0)$ and $G(r,s)$ is decreasing in $s\in(0,1)$ for each $r>0$. Also, $G(r,1)=0$.

Thus, \eqref{10} follows. $\quad\Box$

---

Below are the symbolic calculations, in Mathematica, of $H(p,u,v)$, $G(r,s)$, $G_1(r,s)$, $G_2(r,s)$, $q(s),q(1),q'(1),q''(s)$, $G_1(0,s)$, and $G(r,1)$: