I want to draw the Desargues configuration $10_3$ in LaTeX using the standard picture environment, which allows only lines with the slopes $n:m$ where $\max\{|n|,|m|\}\le 6$. Is it possible? If not, then what is the smallest number $s$ for which there exists a drawing of the Desargues configuration $10_3$ in which all lines have slopes $n:m$ with $\max\{|n|,|m|\}\le s$? And for this smallest $s$, what is the smallest number $c$ for which there exists a drawing of the Desargues configuration $10_3$ in which all lines have slopes $n:m$ with $\max\{|n|,|m|\}\le s$ and all points have integer coordinates $(x,y)$ with $\max\{|x|,|y|\}\le c$?
$\begingroup$
$\endgroup$
4
-
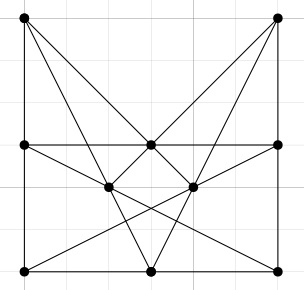
1$\begingroup$ Is this image OK? $\endgroup$– Alex RavskyCommented Jul 28, 2023 at 16:29
-
1$\begingroup$ Perhaps you'll get more replies on TeX SE: tex.stackexchange.com $\endgroup$– Max Lonysa MullerCommented Jul 28, 2023 at 16:42
-
$\begingroup$ @AlexRavsky Yes, Sasha, it is perfect: uses only lines with slopes $(n,m)$ where $\max\{|n|,|m|\}\le 2$, which is probably the best possible. And the coordinates are also small. Very good. Please write down this as an answer and I will accept. $\endgroup$– Taras BanakhCommented Jul 28, 2023 at 16:49
-
1$\begingroup$ Done........... $\endgroup$– Alex RavskyCommented Jul 28, 2023 at 17:31
Add a comment
|
1 Answer
$\begingroup$
$\endgroup$
1
This example shows that $s\le 2$ and for this $s$, $c\le 3$. Note that we are lucky with the latter, because there is a configuration for which every combinatorially equivalent realization has at least one irrational number as one of its coordinates.
-
2$\begingroup$ Such a simple and beautiful drawing of the classical Desargue configuration! It is strange that Google does not find it is the Internet... $\endgroup$ Commented Jul 28, 2023 at 18:12