0
$\begingroup$

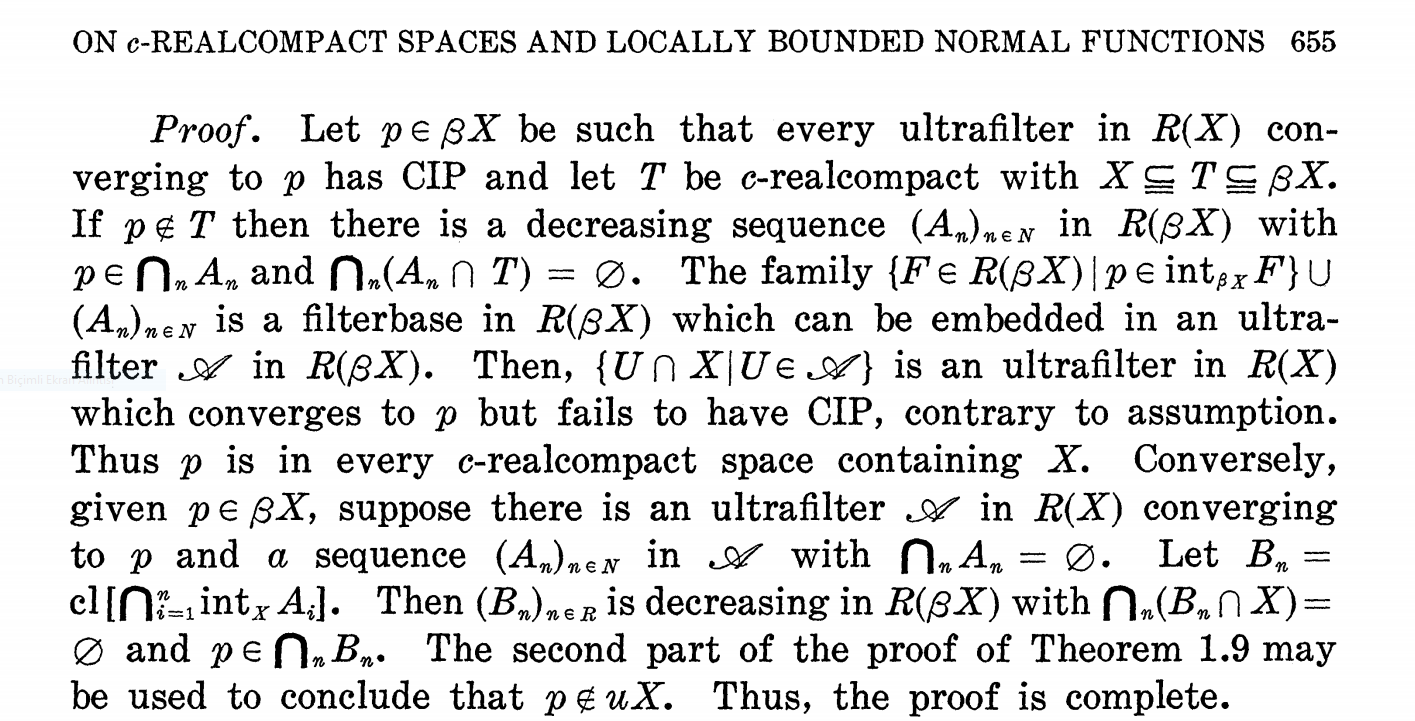
K. Hardy and R. G. Wood assert that the family in line 4 is a filterbase. I couldn't show it.

enter image description here

Improve this question
$\endgroup$

1 Answer 1

Reset to default
1
$\begingroup$

The first part of the family, $\{F:p\in\mathrm{int}_{\beta X}F\}$ is a family of neighbourhoods of $p$. Each $A_n$ is the closure of its interior (the $R$ suggests we have regular closed sets here). Take finitely many $F$s and finitely many $A_n$s. Among the latter there is a smallest set: the one with the largest index, $A_m$ say. The intersection, $I$, of the $F$s is a neighbourhood of $p$, so the interior of $I$ intersects the interior of $A_m$. That shows that the whole finite family has an intersection with non-empty interior. So the family has the finite intersection property. That is enough to make the proof go through. But it is not necessarily a filterbase: the intersection of an $F$ and an $A_n$ need not contain another $F$ nor another $A_m$.

Improve this answer
$\endgroup$
7
  • $\begingroup$ Actually, I tried to show that it was a filterbasis, but it wasn't. Actually, isn't it obvious that this family satisfies the finite intersection property? Both $F$'s and $A_n$'s contain $p$ so its intersection is not empty. Why are you emphasizing that it has an intersection with non-empty interior? Is there something I can't see? $\endgroup$
    – Mehmet Onat
    Commented Jun 16, 2023 at 21:55
  • $\begingroup$ i guess its for a filterbase of regular closed sets $\endgroup$
    – Mehmet Onat
    Commented Jun 17, 2023 at 6:19
  • 1
    $\begingroup$ The next line extends the family to an ultrafilter in the algebra of regular open sets; in that algebra "non-zero intersection" means that the interiors intersect. Here it is not given that $p$ is in the interior of all $A_n$. $\endgroup$
    – KP Hart
    Commented Jun 17, 2023 at 9:56
  • $\begingroup$ In the above proof, why $B_n=cl[\bigcap_{i=1}^{n}int_X A_{i}]$ is in $R(\beta X)$? $\endgroup$
    – Mehmet Onat
    Commented Sep 12, 2023 at 13:08
  • 1
    $\begingroup$ There is an open set $O$ in $\beta X$ such that $O\cap X=\bigcap_{i=1}^n\operatorname{int}_XA_i$. And $O$ and $O\cap X$ have the same closure in $\beta X$. $\endgroup$
    – KP Hart
    Commented Sep 16, 2023 at 10:56

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .