Does anyone recognize the following theorem on probability distributions?

Question

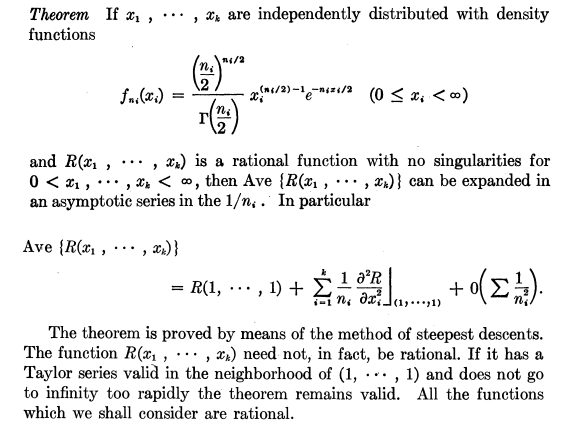
I am reading article [1] of Paul Meier, and on page 7, the following probability distribution function is given:

Theorem: If $x_1, \dots, x_k$ are independently distributed with density functions: $$f_{n_i}(x_i) = \left(\frac{n_i}{2}\right)^{\frac{n_i}{2}} \frac{x_i^{\frac{n_i}{2}-1}e^{-\frac{n_ix_i}{2}}}{\Gamma\left(\frac{n_i}{2}\right)}$$ for $0 \leq x_i < \infty$ and $R(x_1,\dots,x_k)$ is a rational function with no singularities for $0 < x_1,\dots,x_k < \infty$, then $\text{Ave}\{R(x_1,\dots,x_k)\}$ can be expanded in an asymptotic series in $\frac{1}{n_i}$ . In particular:

$$\text{Ave}\{R(x_1,\dots,x_k)\} = R(1,\dots,1) + \sum_{i=1}^k \frac{1}{n_i} \left.\frac{\partial^2 R}{\partial x_i^2}\right|_{(1,\dots,1)} + O\left(\sum \frac{1}{n_i^2}\right)$$

My Question: It looks like in this theorem, the probability distribution $f_{n_i}(x_i)$ is a Chi-Square Distribution - but does anyone know any more information about this theorem? Does it have a name?

I have been trying to find more information about it to learn where it comes from, why it is useful and why it is true (i.e. proof).

Thanks!

Note: Screenshot of the paper in case I transcribed it incorrectly :

Reference:

[1] Meier, Paul. “Variance of a Weighted Mean.” Biometrics, vol. 9, no. 1, 1953, pp. 59–73. JSTOR, https://doi.org/10.2307/3001633.

Answer 1

Q: Does anyone know any more information about this theorem? Does it have a name? Why is it true?

This result is simply referred to as "Meier's theorem" in the literature. It follows from the property that for large $n$ the chi-square distribution tends to a narrow Gaussian, $$f_{n}(x)\mapsto \sqrt{\frac{n}{4\pi}}e^{-(n/4)(x-1)^2}.$$ Now you can expand the function $R$ in a Taylor series around the peak of the Gaussian, $$R(x_1,x_2,\ldots x_k)=R(1,1,\ldots 1)+\sum_{i=1}^k \left.(x_i-1)\frac{\partial R}{\partial x_i}\right|_{(1,\dots,1)}+\frac{1}{2}\sum_{i=1}^k (x_i-1)^2 \left.\frac{\partial^2 R}{\partial x_i^2}\right|_{(1,\dots,1)}+{\cal O}(x-1)^3,$$ and perform the Gaussian average, $$\mathbb{E}[R]=R(1,1,\ldots 1)+\sum_{i=1}^k \left.\frac{1}{n_i}\frac{\partial^2 R}{\partial x_i^2}\right|_{(1,\dots,1)}+{\cal O}(n^{-2}).$$