Decomposition of a tensor product of representations of $\mathrm{GL}_l(\mathbb{C})$ and decomposition of Littlewood-Richardson numbers?

Question

For a positive integer $m$, denote $T(m)=\{(\lambda_1,\dots,\lambda_m)\in \mathbb{Z}^m:\lambda_1\ge \lambda_2\ge\dots \ge\lambda_m\}$ and $T^+(m)=\{ (\lambda_1,\dots,\lambda_m)\in \mathbb{Z}^m:\lambda_1\ge \lambda_2\ge\dots \ge\lambda_m\ge 0\}$. For $\lambda=(\lambda_1,\dots,\lambda_m)\in T(m)$, denote $|\lambda|=\lambda_1+\dots+\lambda_m$. Then an element $\lambda$ in $T^+(m)$ can be viewed as a partition of $|\lambda|$. For an element $\lambda\in T(m)$, denote by $\Psi_\lambda$ the irreducible representation of $\mathrm{GL}_m(\mathbb{C})$ with maximal weight $\lambda$. Note that if $\lambda=(\lambda_1,\dots,\lambda_m)$, then $\lambda^+:=(\lambda_1-\lambda_m,\lambda_2-\lambda_m,\dots,\lambda_{m-1}-\lambda_m,0)\in T^+(m)$ and $\Psi_{\lambda}=\Psi_{\lambda^+}\otimes D_{\lambda_m}$, where $D$ denotes the determinant and $D_{\lambda_m}=\det^{\lambda_m}$. Moreover, if we denote $\lambda^*=(-\lambda_m,\dots,-\lambda_1)$, then it is known that the dual of $\Psi_{\lambda}$ is $\Psi_{\lambda^*}$. Here we follow the notations of Fulton-Harris' book "Representation theory: a first course".

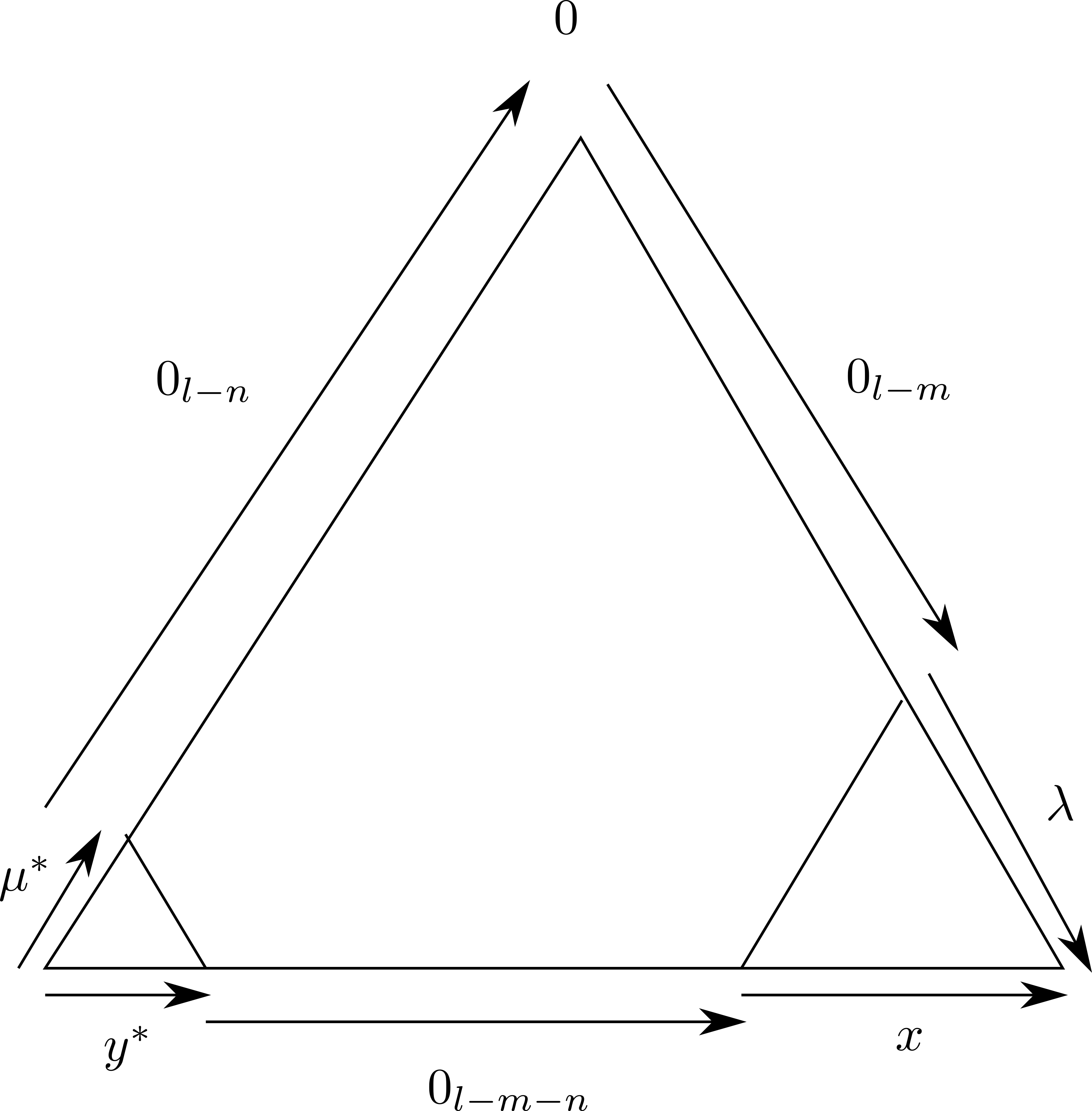
Now let $l,m,n$ be positive integers such that $m+n<l$ and $n\le m$. Let $\lambda\in T^+(m),\mu\in T^+(n)$ and we consider the decomposition $$\Psi_{(\lambda,0_{l-m})}\otimes \Psi_{(0_{l-n},\mu^*)}$$ as a representation of $\mathrm{GL}_l(\mathbb{C})$.

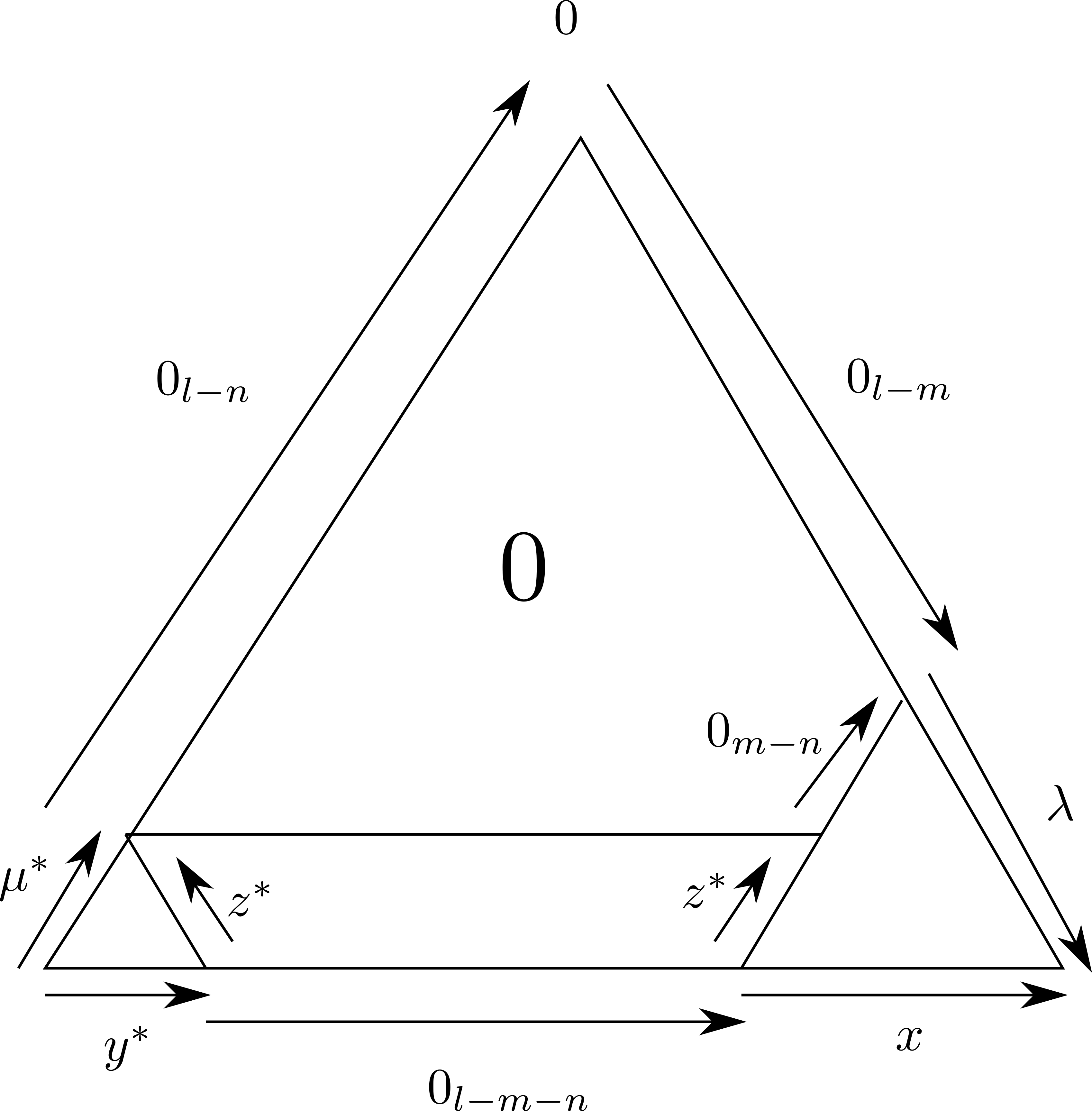
Question: Is the following decomposition true $$\Psi_{(\lambda,0_{l-m})}\otimes \Psi_{(0_{l-n},\mu^*)}=\bigoplus_{x\in T^+(m),y,z\in T^+(n)}N_{xz\lambda}N_{yz\mu}\Psi_{(x,0,y^*)}?$$ Here $N_{xz\lambda}$ is the Littlewood-Richardson number, which is usually written as $c_{xz}^\lambda$ in other literatures.

Note that, we can write $\Psi_{(0_{l-n},\mu^*)}=\Psi_{\tilde\mu}\otimes D_{-\mu_1}$ with $\widetilde \mu=(\mu_1,\dots,\mu_1,\mu_1-\mu_n,\dots,\mu_1-\mu_2,0)$ with $(l-n)$'s $\mu_1$, then by Littlewood-Richardson rule, we can decompose the left side as \begin{align*} \Psi_{(\lambda,0_{l-m})}\otimes \Psi_{(0_{l-n}, \mu^*)}&=\Psi_{(\lambda,0_{l-m})}\otimes \Psi_{\tilde\mu}\otimes D_{-\mu_1}\\ &=\bigoplus_{\nu}N_{\tilde \mu \lambda \nu} \Psi_{\nu} \otimes D_{-\mu_1}. \end{align*} It is not hard to see that if $N_{\tilde \mu \lambda \nu}\ne 0$, then $\nu$ must be of the form $(\nu_1,\dots,\nu_l)$ with $\nu_{m+1}=\dots=\nu_{l-n-1}=\mu_1$, which means that $ \Psi_{\nu}\otimes D_{-\mu_1} $ is of the form $\Psi_{(x,0,y^*)}$ with $x\in T^+(m)$ and $T^+(n)$. Thus the above question is the same as the following

Question': Is the following identity true $$N_{\tilde \mu \lambda \nu}=\sum_{z\in T^+(n)}N_{xz\lambda}N_{yz\mu},$$ for any $\lambda,x\in T^+(m),\mu,y\in T^+(n)$ (one can add the condition $|\lambda|-|\mu|=|x|-|y|$)? Here $\tilde \mu=(\mu_1,\dots,\mu_1,\mu_1-\mu_n,\dots,\mu_1-\mu_2,0)$, and $\nu=(x,0,y^*)+(\mu_1,\dots,\mu_1).$

I checked several small examples, and it seems that the above questions have positive answer. I believe they should be true in general. I am wondering if this is a well-known result. Any comments and references will be appreciated. Thanks in advance.

Edit: Some small typos were fixed. I did not expect this question will draw the attention of Professor Terry Tao. I really appreciate Professor Tao for his time to answer this question. Thanks.

Answer 1

This identity can be deduced from the hive model of Littlewood-Richardson coefficients, which Allen Knutson and I introduced in

Knutson, Allen; Tao, Terence, The honeycomb model of (\text{GL}_n(\mathbb C)) tensor products. I: Proof of the saturation conjecture, J. Am. Math. Soc. 12, No. 4, 1055-1090 (1999). ZBL0944.05097.

The quantity $N_{\tilde \mu \lambda \nu}$ is the number of (integer-valued) hives with boundary data

where the arrows indicate the values of successive differences of hive values (to be consistent with your conventions one has to reverse the order of all the weights, but I won't indicate that explicitly), and the 0 at the top means that we have normalized the top hive value to be zero. The two interior line segments have no significance at present, other than to indicate (via the two equilateral triangles that they border) that $\mu, y$ have the same length, and $\lambda, x$ have the same length.

Because of the concavity properties of hives, we can fill in a lot of the entries here, and the hive must take the following form

for some non-negative weight $z$ (in particular, the hive vanishes completely in the upper trapezoid, and horizontally constant on the lower trapezoid). Conversely, for any such $z$ and any hives filling in the bottom left and bottom right triangles, there is a unique extension to a hive in the large triangle. This gives your desired combinatorial identity.

One can also establish this identity using other combinatorial models of the Littlewood-Richardson rule, such as honeycombs or Young tableaux, though I haven't worked out how the argument would work in those models.