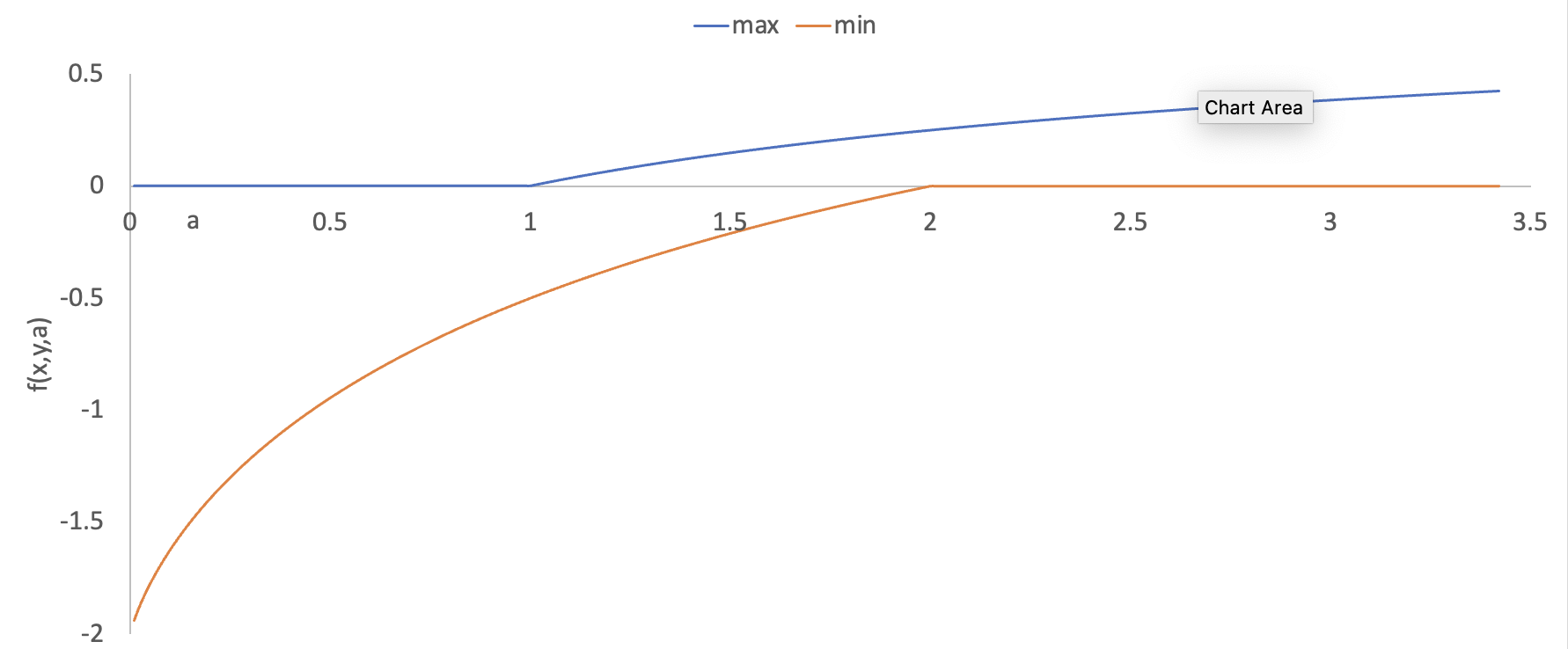
As Iosif observed, you have $-2 \leq c_a \leq C_a \leq 2$ for any $a$.
Slightly improved upper bounds
When $a < 1$
The expression is symmetric in $x$ and $y$, so we can WLOG assume $y \geq x$. Let $\beta = y^a / x^a$ and $\beta^* = y^a / (y^a - x^a)$ its Holder conjugate. Then Young's inequality states
$$\tag{*} x y^\beta \leq \frac{x^{\beta^*}}{\beta^*} + \frac{y^{\beta^2}}{\beta} $$
and
$$\tag{**} x^{\beta^{-1}} y \leq \frac{x}{\beta} + \frac{y^{\beta^*}}{\beta^*} $$
Using that $a < 1 \leq \beta,\beta^*$ and that $x,y \leq 1$, we therefore conclude
$$ xy^\beta + y x^{\beta^{-1}} \leq (x^a + y^a) \left( \frac{1}{\beta} + \frac{1}{\beta^*} \right) = x^a + y^a $$
and hence $f(x,y,a) \leq 0$ for all $a < 1$. Taking the limit as $x,y \to 0$ for any fixed $a$ shows that this estimate is sharp. This proves that the best $C_a$ when $a < 1$ is $C_a = 0$.
When $a \geq 1$
Expressions (*) and (**) still hold in general, so the same argument proves that
$$ x y^\beta + y x^{\beta^{-1}} \leq x + y \implies f(x,y,a) \leq x - x^a + y - y^a $$
This expression can be optimized using single variable calculus to obtain the upper bound
$$ f(x,y,a) \leq 2 \left[ \left( \frac1a\right)^{\frac1{a-1}} - \left( \frac1a \right)^{\frac{a}{a-1}} \right] < 2 $$
(Note that this is a worse bound than was proposed in my comments to the OP.)
Sharp lower bounds
When $a \geq 2$
Again, since the expression is symmetric in $x,y$, we can assume WLOG that $y \leq x$; write $y = \lambda x$ for $\lambda \in (0,1]$. Our function
$$ f = x^{1+\lambda^a} \lambda^{\lambda^a} + x^{1 + \lambda^{-a}} \lambda - x^a (1 + \lambda^a) $$
Observe that
$$ (1+\lambda^a)^{-1} + (1 + \lambda^{-a})^{-1} = 1 $$
and so we can apply Young's inequality (writing the first two terms as $A^{1+ \lambda^a} / (1 + \lambda^a)$ and $B^{1+\lambda^{-a}} / (1 + \lambda^{-a})$ respectively) to find that
$$ \tag{***} f \geq AB - x^a(1+\lambda^a) = x^2 \lambda^{\frac{(2-a)\lambda^a}{1+\lambda^a}} (1+\lambda^a) - x^a(1+\lambda^a)$$
Note that when $a \geq 2$, we have $\ln(\lambda) \cdot \frac{(2-a)\lambda^a}{1+\lambda^a} \geq 0$ for all $\lambda \in (0,1]$, and hence we have
$$ f \geq (x^2 - x^a)(1 + \lambda^a) \geq 0$$
This shows the sharp lower bound when $a \geq 2$ is $c_a = 0$. (The sharpness comes from considering what happens as we take $x$ and $y$ to both approach $0$.)
When $a < 2$
It is again convenient to rewrite in terms of $\beta = \lambda^a$, so we have (using (***) and replacing $\lambda$ by $\beta$)
$$ f \geq x^2 \beta^{\frac{2-a}{a} \frac{\beta}{1+\beta}} (1+\beta) - x^a (1+\beta) $$
Optimize first in $x$ for a fixed $\beta$, we find that the minimum of RHS is attained when $x^{a-2} = \frac2a \beta^{\frac{2-a}{a} \frac{\beta}{1+\beta}}$. Reinserting we see that, for $\beta \in (0,1]$, we have
$$ f \geq \left[ \left(\frac{a}{2}\right)^{\frac{2}{2-a}} - \left(\frac{a}{2}\right)^{\frac{a}{2-a}}\right] \beta^{-\frac{\beta}{1+\beta}}(1+\beta) $$
The function $\beta^{-\frac{\beta}{1+\beta}}(1+\beta)$ attains its maximum at $\beta = 1$, where it takes the value $2$, and hence we have
$$f \geq 2\left[ \left(\frac{a}{2}\right)^{\frac{2}{2-a}} - \left(\frac{a}{2}\right)^{\frac{a}{2-a}}\right] $$
This shows that when $a < 2$, we can bound $c_a \geq 2\left[ \left(\frac{a}{2}\right)^{\frac{2}{2-a}} - \left(\frac{a}{2}\right)^{\frac{a}{2-a}}\right]$. Now, as observed in my previous comment, if you evaluate $f$ along the diagonal $f(x,x,a)$ this becomes a single-variable optimization problem, which can be solved by calculus. And one can check therefore that this lower bound is in fact attained for a well-chosen $x$ (and $y = x$), showing that it is sharp.