What structure is preserved by pseudo-homeomorphisms of pseudo-Euclidean spaces?

Question

Let us recall that for integer numbers $t,s\ge 0$ the pseudo-Euclidean space $\mathbb R^{t,s}$ is the vector space $\mathbb R^{t+s}$ endowed with the quadratic form $q_{t,s}:\mathbb R^{t+s}\to\mathbb R$ assigning to every vector $x=(x)_{i\in t+s}$ the real number $$q_{t,s}(x)=\sum_{i\in t}x_i^2-\sum_{j\in s}x_{t+j}^2.$$ The pseudo-Euclidean space $\mathbb R^{1,3}$, called the Minkowski spacetime, plays an important role in the Einstein Relativity Theory.

For every $x\in\mathbb R^{t,s}$ and $\varepsilon>0$, let $$B_{t,s}(x,\varepsilon):=\{y\in\mathbb R^{t,s}:|q_{t,s}(x-y)|<\varepsilon^2\}$$be the pseudo-ball of radius $\varepsilon$ around $x$.

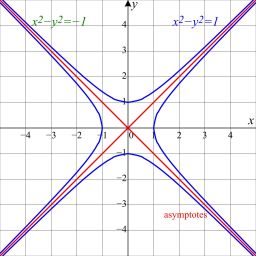
In the pseudo-Euclidean space $\mathbb R^{1,1}$ the pseudo-ball of radius one around zero is the set of points between the hyperbolas:

A function $f:\mathbb R^{t,s}\to \mathbb R^{t,s}$ is defined to be pseudo-continuous if $$\forall x\in\mathbb R^{t,s}\;\forall\varepsilon>0\;\exists \delta>0\;f[B_{t,s}(x,\delta)]\subseteq B_{t,s}(f(x),\varepsilon).$$

A function $f:\mathbb R^{t,s}\to \mathbb R^{t,s}$ is defined to be a pseudo-homeomorphism of the pseudo-Euclidean space $\mathbb R^{t,s}$ if $f$ is bijective and the functions $f$ and $f^{-1}$ are pseudo-continuous.

The following natural problems are motivated by the famous Erlangen Program of Felix Klein.

Problem 1. Describe the structure of individual pseudo-homeomorphisms of the pseudo-Euclidean spaces $\mathbb R^{t,s}$ and the structure of the group of pseudo-homeomorphisms of $\mathbb R^{t,s}$ (at least in the simplest non-trivial case of the pseudo-Euclidean space $\mathbb R^{1,1}$).

Problem 2. What structure of the pseudo-Euclidean space $\mathbb R^{t,s}$ is preserved by pseudo-homeomorphisms (so that a bijective function $f:\mathbb R^{t,s}\to\mathbb R^{t,s}$ is a pseudo-homeomorphism if and only if it preserves this structure)?

Remark. The notion of pseudo-continuity is not topological: no topology on $\mathbb R^{t,s}$ exists in which the continuity of functions is equivalent to the pseudo-continuity. So, the hypothetical structure on $\mathbb R^{t,s}$ preserved by pseudo-homeomorphisms (which can be called the pseudo-topology) should be something else, different from a topology.

Answer 1

Solution to problem 1 and 2 in the $s=t=1$ case

In the $(1,1)$ case, change the quadratic form to $(x,y)\mapsto xy$ instead of $(x,y)\mapsto x^2 - y^2$. This is merely a change of coordinates.

Theorem: A function $f:\mathbb R^{1,1}\to \mathbb R^{1,1}$ is "pseudocontinuous" if and only if:

$$f(x,y)\equiv(g(x),h(y)),$$ or $$f(x,y)\equiv(h(y),g(x)),$$ for any pair of continuous functions $g,h:\mathbb R\to\mathbb R$. In particular, the group of pseudo-automorphisms is the semidirect product $(\operatorname{Aut}(\mathbb R)\times \operatorname{Aut}(\mathbb R))\rtimes C_2$, where $\operatorname{Aut}(\mathbb R)$ is the set of automorphisms of $\mathbb R$ as a topological space.

Why? I list the sequence of lemmas which one should prove:

Lemma 1: A "cross" (that is, the union of a line parallel to the $x$ axis with a line parallel to the $y$ axis) necessarily gets mapped to another cross by any pseudocontinuous function $f$.

Lemma 2: A horizontal line or vertical line gets mapped to a horizontal or vertical line by pseudocontinuous $f$.

Lemma 3: For fixed $x$, the function $y \mapsto f(x,y)$ is continuous. The same is true for $x \mapsto f(x,y)$ for fixed $y$.

Lemma 4: The function $f$ is continuous everywhere.

Theorem: The above statement.