Problem. Let $G$ be a connected Lie group and $H$ is a closed subgroup of $G$ such that the homogeneous space $G/H$ is contractible. Is $G/H$ homeomorphic to a Euclidean space $\mathbb R^n$ for some $n\in\omega$?
-
2$\begingroup$ I believe the answer is yes and is Wu-Chung Hsiang era topology, i.e. some paper from the 60's (maybe early 70's). But I don't know a precise reference for you. They likely also know it is diffeomorphic to the euclidean space. One of the theorems back then was homogenous spaces do not come with exotic smooth structures. $\endgroup$– Ryan BudneyCommented Mar 29, 2023 at 5:56
-
$\begingroup$ Yes. Szenthe proved this for locally compact topological groups. See acta.bibl.u-szeged.hu/14497 or the epilogue of a survey by Halverson and Repovs arxiv.org/abs/0811.0886. $\endgroup$– Shijie GuCommented Mar 29, 2023 at 6:53
-
$\begingroup$ @ShijieGu Thank you for the links to these papers. But they discuss only local structure of homogeneous spaces and show that they are topological manifolds. But this is not sufficient to answer my question because contractible manifolds need not be homeomorphic to Euclidean spaces. $\endgroup$– Taras BanakhCommented Mar 29, 2023 at 8:17
-
2$\begingroup$ @TarasBanakh You are right. It seems Szenthe proved this without the assumption of the connectedness. With the connectedness, the group is almost connected. By a Theorem (1.2) of Antonyan (arxiv.org/pdf/1104.1820v1.pdf), H is maximal compact. Then Malcev–Iwasawa theorem or Theorem 32.5 in Stroppel's book (ems.press/books/etb/15) shows that G/H is homeomorphic to a Euclidean space. $\endgroup$– Shijie GuCommented Mar 29, 2023 at 9:30
-
1$\begingroup$ @ShijieGu Thank you for the comment. Following the suggestion of Dusan Repovs I have written an email to you several minutes ago. If you want you can copy your comment as an answer and I will accept it (in order to close this question as answered). What about the other my question:mathoverflow.net/q/443695/61536 ? $\endgroup$– Taras BanakhCommented Mar 29, 2023 at 10:03
2 Answers
The main references would be Theorem 1.2 of Sergey Antonyan's paper and Theorem 32.5 in Markus Stroppel's book.
Topological group $G$ is called almost connected if the factor group $G/G_0$ of $G$ modulo the connected component $G_0$ of the identity is compact.
Theorem 1 (Antonyan). Let $G$ be a locally compact almost connected group. Then a compact subgroup $H \subset G$ is maximal compact if and only if the coset space $G/H$ is contractible.
Since $G$ is connected and that $G/H$ is contractible, Theorem 1 implies that $H$ is maximal compact. Then the following theorem (Theorem 32.5 in Stroppel's book), which is due to Malcev and Iwasawa, will finish the proof.
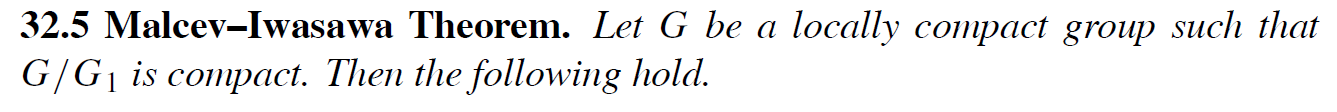

It might be worth mentioning that a generalization can be obtained if one drops the connectedness hypothesis. The following theorem is due to Szenthe or see Theorem 7.5 in a survey by Halverson and Repovs.
Theorem (Szenthe). Let $G$ be a locally compact almost connected topological group. Then for any closed subgroup $H \subset G$, the coset space $G/H$ is a disjoint union of topological manifolds if and only if it is locally contractible.
-
2$\begingroup$ Just a comment: Szenthe's proof turned out to contain a serious gap, but the theorem is correct. Complete proofs were given later by Antonyan and Dobrowolski [Antonyan, Sergey; Dobrowolski, Tadeusz, Locally contractible coset spaces. Forum Math. 27 (2015), no. 4, 2157–2175. ] and by Hofmann an myself [Hofmann, Karl H.; Kramer, Linus, Transitive actions of locally compact groups on locally contractible spaces. J. Reine Angew. Math. 702 (2015), 227–243.]. $\endgroup$– LinusCommented Apr 11, 2023 at 11:59
-
$\begingroup$ Antonyan's theorem is not applicable here, because $H$ is not assumed to be compact. So this does not answer the Problem. $\endgroup$– LinusCommented Apr 12, 2023 at 9:04
-
$\begingroup$ @Linus Thank you, Prof. Kramer. Yes, my argument doesn't work without assuming that H is compact. Your answer is the correct one. $\endgroup$ Commented Apr 12, 2023 at 12:19
I don't have an answer. Note that $H$ need not be compact.
For example, $G$ could be the universal covering of $SL_2\mathbb R$, and
$H$ could be a $1$-dimensional closed subgroup.
Here are some more thoughts. Since $X=G/H$ is contractible, $H\hookrightarrow G$ is a homotopy equivalence. In particular, $H$ is connected. Let $K$ be a maximal compact subgroup of $H$. Then $K\hookrightarrow H$ is also a homotopy equivalence. Now let $L$ be a maximal compact subgroup of $G$ containing $K$. Then $L\hookrightarrow G$ is another homotopy equivalence, and hence $K\hookrightarrow L$ is a homotopy equivalence. This implies that $K=L$. So we have a bundle $H/K\to G/K \to G/H$. By Iwasawa's theorem (mentioned in Shijie Gu's answer above) the fibre $H/K$ and the total space $G/K$ are homeomorphic to $\mathbb R^m$ and $\mathbb R^n$, respectively. Since $X$ is contractible, the bundle is trivial and thus $\mathbb R^n\times X\cong \mathbb R^m$.
Edit. The answer to the Problem is 'yes', and this is due to Mostow [Mostow, G. D., Covariant fiberings of Klein spaces. II., Amer. J. Math. 84 (1962), 466–474.] Mostow proves the following. Let $G$ be a Lie group with finitely many components and let $H$ be a closed subgroup, also with finitely many components. Let $K$ a maximal compact subgroup of $H$, and $L$ a maximal compact subgroup in $G$ containing $K$. Then there exist closed submanifolds $E,F\subseteq G$, diffeomorphic to $\mathbb R^k$ and $\mathbb R^\ell$, respectively, such that the maps $K\times E\to KE=H$ and $L\times E\times F\to LEF=G$ are diffeomorphisms, and such that $F$ is invariant under conjugation by $K$. It follows that $G/H$ is diffeomorphic to $L\times_KF$ (which is the total space of a vector bundle over $L/K$). In our situation, where $K=L$, this means that $G/H$ is diffeomeorphic to $F$.
(I found this in the book by Gorbatsevich, Onishchik and Vinberg, 'Foundations of Lie theory and Lie transformation groups'.)
