What is $(C, D, \delta, \gamma)$ and $(C, \delta; D, \gamma)$ Desarguesian?

Question

A projective plane is $(C, \gamma)$-Desarguesian if for any 2 triangles $A_1 B_1 C_1, A_2 B_2 C_2$ in perspective from $C$ (which means $C \in A_1 A_2, B_1 B_2, C_1 C_2$) such that $A_1 B_1 \cap A_2 B_2, A_1 C_1 \cap A_2 C_2$ lie on $\gamma,$ so does $B_1 C_1 \cap B_2 C_2.$

Zickert's Projektive Ebenen (1955) defines $(C, D, \delta, \gamma)$-Desarguian on page 76 in the following way:

Macht man nun eine der Geraden $\alpha_{ik}$ oder $\beta_{ik}$ sowie einen der Punkte $A_i, A_k$ bzw. $B_i, B_k$ zu Festelementen mit den Werten $\delta,D,$ so entsteht nach dem eben Bewiesenen nur eine Spezialisierung, die als Desarguesscher $(C, D, \delta, \gamma)$-Satz bezeiehnet werden soll.

I translated this as such:

If you fix one of the lines $\alpha_{ik}$ or $\beta_{ik}$ and one of the Points $A_i, A_k$ or $B_i, B_k$ as $​​\delta,D$ respectively, according to what has just been proved, only one specialization arises, which should be called Desargues' $(C, D, \delta, \gamma)$ theorem

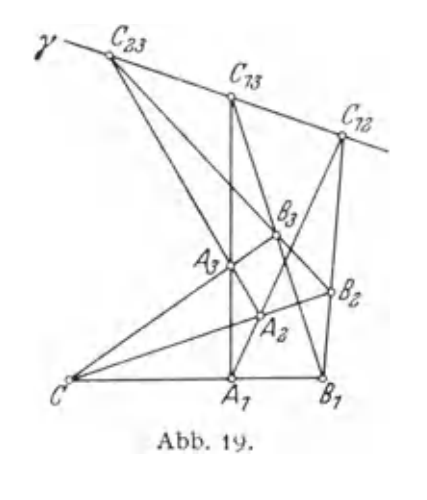
$\alpha_{ik}, \beta_{ik}$ is the line $A_i A_k, B_i B_k$ respectively. Here is the diagram:

As I see it, a projective plane is $(C, D, \delta, \gamma)$-Desarguian if for any 2 triangles $A_1 B_1 C_1, A_2 B_2 C_2$ in perspective from $C$ such that $A_1 B_1 \cap A_2 B_2, A_1 C_1 \cap A_2 C_2$ lie on $\gamma$ and $A_1 = D, A_1 A_k = \delta,$ we have $B_1 C_1 \cap B_2 C_2 \in \gamma.$ In other words, only $(C, \gamma)$ Desarguesian for a special case of the configuration determined by fixing one side of one of the triangles. Is this correct?

Now the author proceeds to define $(C, \delta; D, \gamma)$ Desarguian, which I don't get.

Soll nun ein solches Paar zusammengehoriger Variabler zu Festelementen mit den Werten $C',\gamma'$ gemacht werden, so ergibt das nach S. 74/75 also nur zwei verschiedene Spezialisierungen, wenn wieder die Wahl von $C_{23}$ als Festelement ausscheidet. Die folgende Verabredung macht es jedoch moglich, fUr beide nur eine einzige Bezeichnung zu verwenden. Aus der Voraussetzung des $(C,\gamma)$-Satzes folgt namlich $A_1 \not\in \gamma, C \not\in \beta_{23}, C_{12} \in \gamma, C \in \gamma_3.$ Es erscheint daher sinnvoll, von $C',\gamma'$ entweder $$(5) \ \ C \not\in \gamma', C' \not\in \gamma$$ oder $$(6) \ \ C \in \gamma', C' \in \gamma$$ zu verlangen. Je nachdem, ob (5) oder (6) erfiillt ist, soll dann unter dem Desarguesschen $(C,\gamma; C',\gamma')$-Satz die Spezialisierung mit $C' = A_1, \gamma' = \beta_{23}$ oder die mit $C' = C_{12}, \gamma' = \gamma_3$ verstanden werden.

I translated this as follows:

If I now fix such a pair of related variables to $C',\gamma',$ then according to p.74-5 this results in only two different specializations, if again the choice of $C_{23}$ is fixed. However, the following agreement makes it possible for both to use a single designation. From the assumption of the $(C,\gamma)$-theorem it follows that $A_1 \not\in \gamma, C \not\in \beta_{23}, C_{12} \in \gamma, C \in \gamma_3.$ It therefore makes sense to require from $C', \gamma'$ that either $$(5) \ \ C \not\in \gamma', C' \not\in \gamma$$ oder $$(6) \ \ C \in \gamma', C' \in \gamma.$$ Depending on whether (5) or (6) is fulfilled, under the Desargues $(C, \gamma; C', \gamma')$ theorem the specialization can be understood with $C' = A_1, \gamma' = \beta_{23}$ or with $C' = C_{12}, \gamma' = \gamma_3.$

This is the 1st time in the book the notation $(C, \delta; D, \gamma)$ is used, and yet the phrasing makes it seem like it's already been defined. Perhaps I didn't translate correctly, but I don't see how this is a definition.