Is it consistent that the additivity of Lebesgue null sets is greater than $\frak h$?

Question

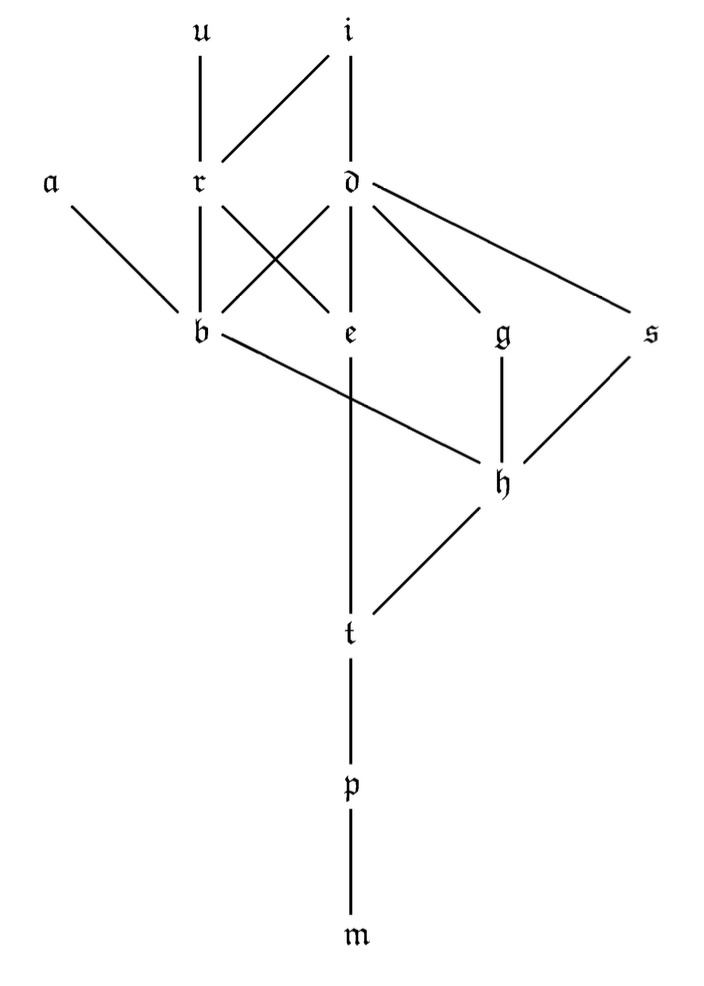
This question concerns combinatorial cardinals of the continuum. Some of these are listed in the following diagram, from Blass's survey on the topic.

There are some additional cardinals, related to analysis of the real line. The smallest of these is add(Null), the minimal number of Lebesgue null susbests of the real line whose union is not Lebesgue null.

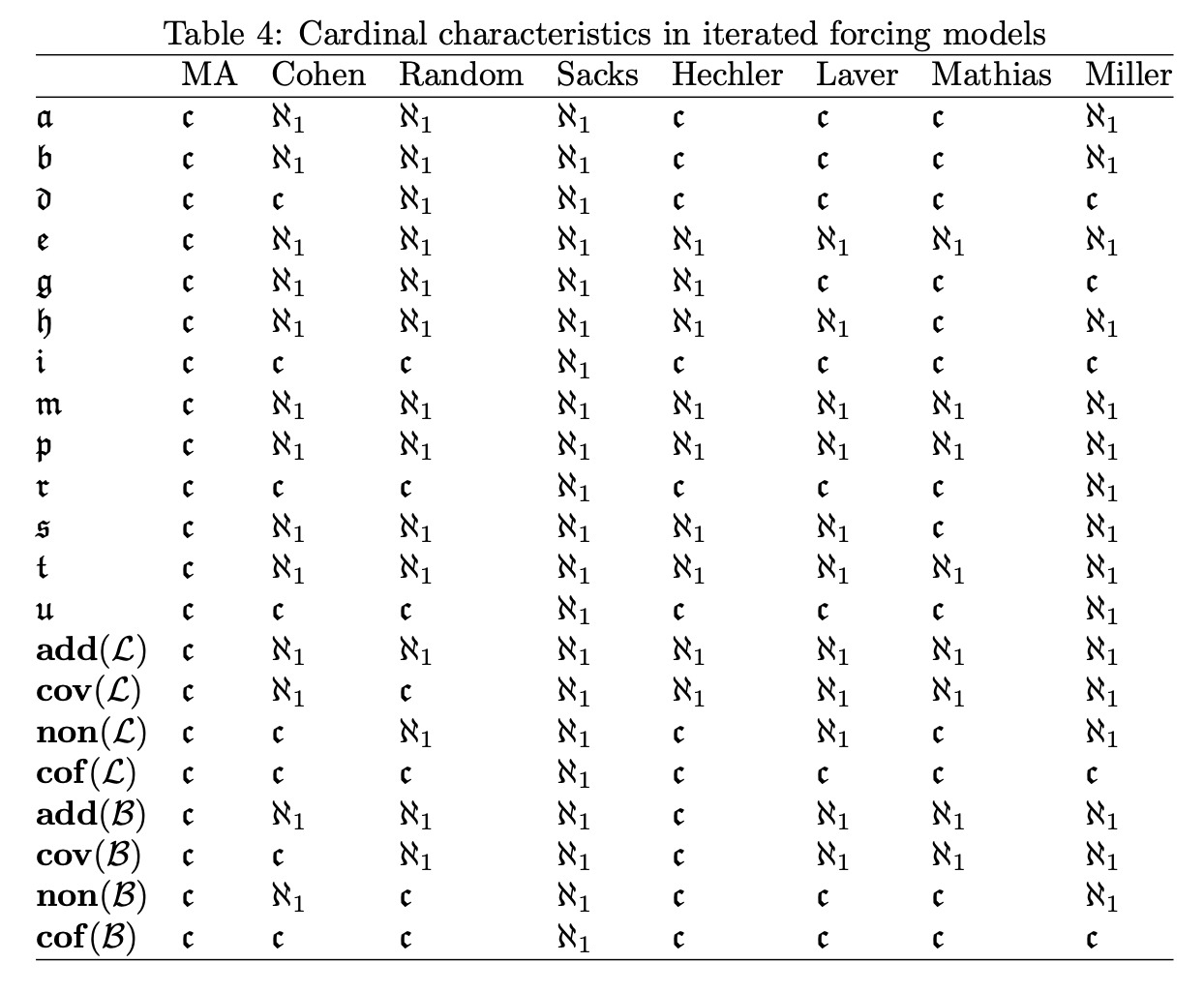
As can be seen in the following table from Blass's survey, in the Mathias model we have add(Null)$<\frak h$. None of these models has $\frak h<$add(Null).

Question: Is it consistent that $\frak h<$add(Null)?

Answer 1

Yes, this is consistent. In fact, it is consistent that $\mathrm{add}(\mathcal N) > \mathfrak{s}$. This was proved by Ihoda and Shelah in

Ihoda, Jaime I.; Shelah, Saharon, Souslin forcing, J. Symb. Log. 53, No. 4, 1188-1207 (1988). ZBL0673.03039.

The paper describes (I think for the first time?) the notion of Souslin forcing, a term used to describe notions of forcing that are definable in some very nice way. They then prove that it is consistent to have $\mathfrak{s} = \aleph_1$, while at the same time Martin's Axiom holds for "nice enough" ccc posets. The Amoeba forcing is "nice enough" for this forcing axiom to apply to it, and this implies $\mathrm{add}(\mathcal N) > \aleph_1$ in this model.