In knot theory, what is this link property and how to detect it: "linkings between components separate nicely"

Question

The following could be made more general (see below), but let's focus on a link $L$ that consists of three components (closed curves) $\gamma_1,\gamma_2,\gamma_3\subset\Bbb R^3$. Call $L$ a necklace if there are 3-balls $B_1,B_2,B_3\subset\Bbb R^3$ so that

1. $B_1\cap B_2\cap B_3=\varnothing$,
2. $\gamma_i\subset B_i$, and
3. $\gamma_i$ has at most two intersections with $\partial B_j$ for all $j\not=i$.

The picture on the left gives an example for a necklace and the corresponding 3-balls.

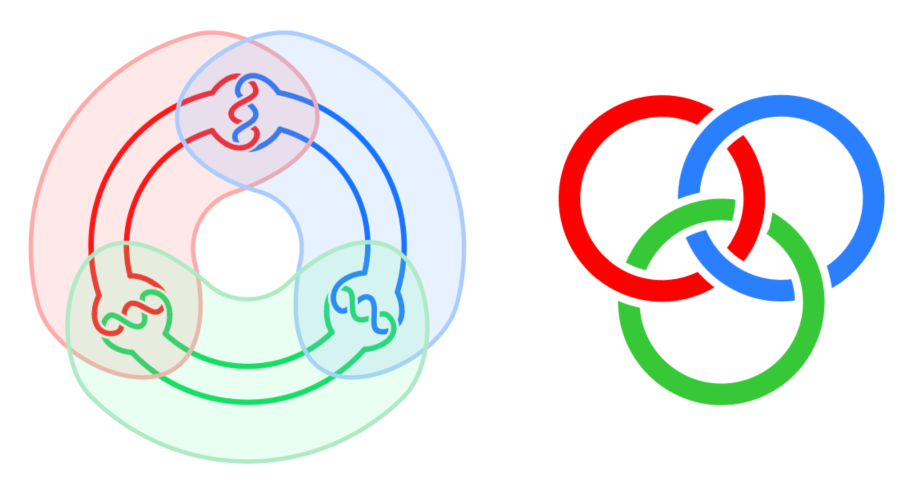
I believe that Borromean rings (on the right), or more generally Brunnian links, are examples of links that are not necklaces.

Questions:

1. Has this concept (or a generalization thereof) been looked at before and what are potential keywords to look it up?
2. Is there a link invariant that can provide an obstruction to being a necklace?

---

Further notes

• One can of course generalize this to more than three components $\gamma_1,...,\gamma_n\subset\Bbb R^3$, and then look at the graph (or better, the simplicial complex) of intersections between the corresponding balls $B_i$ and classify links according to that.

• Being a necklaces should be stronger than being Brunnian, since one does not need that deletion of a single component already unlinks the other two.

Answer 1

Nice question--I'm not sure if this already has a name.

Here is one way to show that a link is not a necklace, that applies to the Borromean rings. Suppose that the link has components $(R,G,B)$, and that the linking number between any two is $0$. (Orient the components any way you like). Then the triple linking number is defined by making $R= \partial S_R$ so that $S_R$ is disjoint from $G$ and $B$, etc. Then the triple linking (aka a Milnor $\bar\mu$ invariant) is defined by counting the intersections of $S_R$, $S_G$, and $S_B$. So it's $\pm 1$ for the Borromean rings.

I claim that the triple linking number is $0$ for a necklace where the pairwise linking numbers vanish.

This follows by showing that we can find a Seifert surface for each component (missing the other components) so that $S_R \cap S_G$ lies in a the ball $B_{RG}$ that contains the Red-Green tangle in your picture, etc. This implies that the 3-way intersection $S_R \cap S_G \cap S_B$ is trivial.

These surfaces are assembled from partial Seifert surface lying in the balls $B_{RG}$ (etc) together with bands joining those balls. Consider the Red-Green tangle in the ball $B_{RG}$. Choose an initial surface with boundary the red portion together with an arc on $\partial B_{RG}$ where the band in your picture goes from $B_{RG}$ to $B_{BR}$. The green arc hits this algebraically 0 times, so you can add tubes to this surface so it's disjoint from the green arc. Now do the same to make a green surface missing the red arc. The intersection of those partial Seifert surfaces lies in $B_{RG}$.

If you join all of these surfaces by bands, then you'll get the desired surfaces.

Probably you can make similar arguments with higher versions of the triple linking number (other $\bar\mu$ invariants, maybe using Cochran's intersections interpretation).