0
$\begingroup$

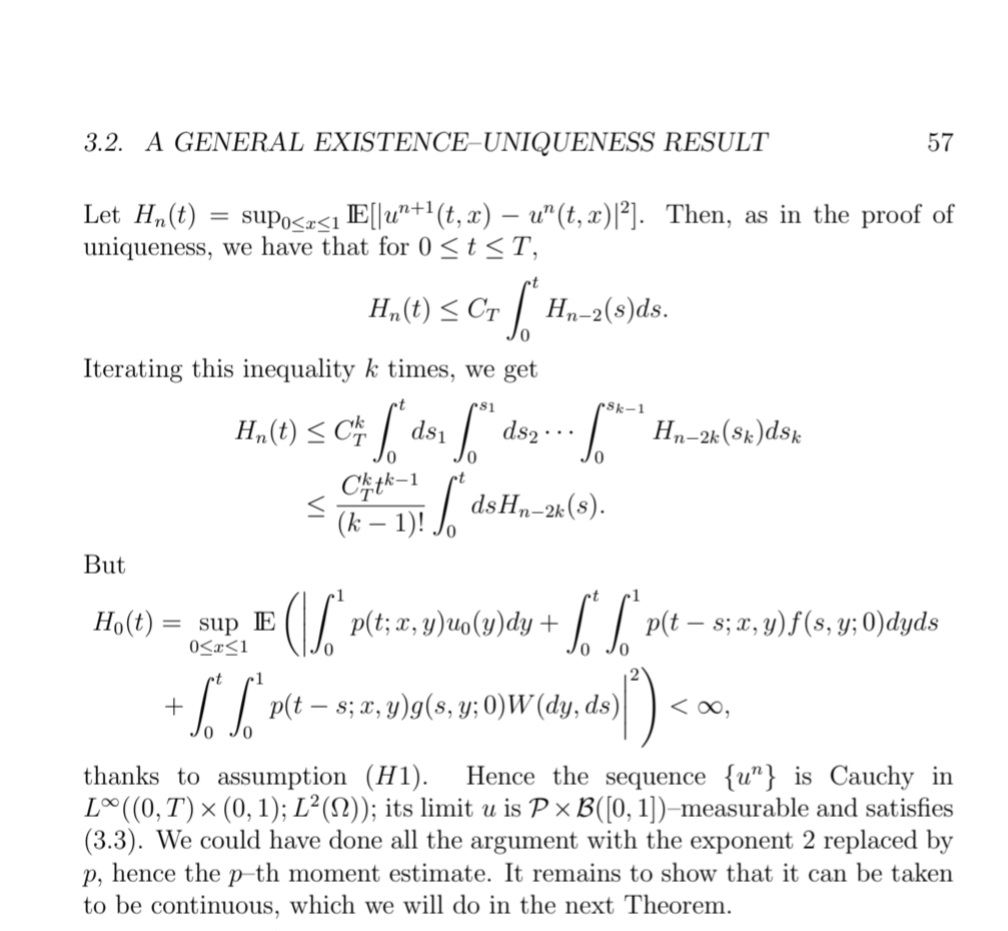
Consider the following theorem (picture below) taken from Pardoux's lecture notes: Stochastic partial differential equations available at scholar google: https://scholar.google.ca/scholar?q=etienne+pardoux+lecture+note+stochastic+partial+differential+equations&hl=en&as_sdt=0&as_vis=1&oi=scholart#d=gs_qabs&t=1674356056002&u=%23p%3DE86Iye_EWKsJ

For the existence he's using Picard iteration to provide a proof.

I have questions: supposing that $u_0$ is predictable ($\mathcal{P} \otimes \mathcal{B}([0,1])$-measurable) how can we prove by induction that $u_n$ is predictable? Also how to deduce that its limit $u$ is predictable? Why $\int_0^1p(t,x,y)u_0(y)dy$ is well defined and predictable? enter image description here enter image description here

Improve this question
$\endgroup$
5
  • $\begingroup$ the $u_{n}$ is defined in terms of integrals up to time $t$ so yes they are adapted to the filtration. $\endgroup$
    – Thomas Kojar
    Commented Jan 22, 2023 at 4:47
  • $\begingroup$ Why are they predictable? $\endgroup$
    – mathex
    Commented Jan 22, 2023 at 5:16
  • $\begingroup$ because they are also continuous as integrals (any left-continuous adapted is predictable) and u0 assumed to be predictable. math.stackexchange.com/questions/352907/… $\endgroup$
    – Thomas Kojar
    Commented Jan 22, 2023 at 5:29
  • $\begingroup$ Here predictable means $\mathcal{P} \otimes \mathcal{B}([0,1])$-measurable used in SPDE, not the one used in Ito integral $\endgroup$
    – mathex
    Commented Jan 22, 2023 at 5:47
  • $\begingroup$ sure and as they mention in those notes, the filtration they consider is with respect to time t. $\endgroup$
    – Thomas Kojar
    Commented Jan 22, 2023 at 5:51

0

Reset to default

You must log in to answer this question.