3
$\begingroup$

Let $a>b>0$.

Suppose we want to minimize $$ f(x)=(x-a)^2+(1/x-b)^2, $$ over $x>0$.

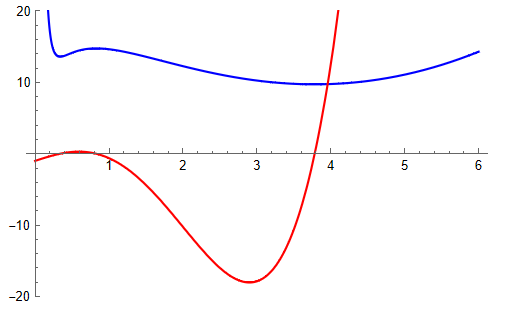
Equating $f'(x)=0$ leads to the quartic equation $$ g(x)=x^4-ax^3+bx-1=0. \tag{1} $$

Question:

Is the smallest positive real root of equation $(1)$ always the minimizer of $f$?

Since $g(0)<0$ and $\lim_{x \to -\infty} g(x)=\lim_{x \to \infty} g(x)=\infty$, there always exist two real solutions, one positive and one negative.

Thus, there can be either one positive root (e.g. when $a=b=1$), or three positive roots (e.g. $a=3,b=4$).

In some numerical examples I tried, the smallest (positive) root was indeed the minimizer, and I wonder whether this is always the case.

Improve this question
$\endgroup$
2
  • $\begingroup$ There is a quartic formula, analogous to (but considerably more complicated than) the quadratic formula. $\endgroup$
    – Gerry Myerson
    Commented Jan 12, 2023 at 18:44
  • 1
    $\begingroup$ Thanks. I have now reformulated the question to be more precise. $\endgroup$
    – Asaf Shachar
    Commented Jan 12, 2023 at 20:35

1 Answer 1

Reset to default
3
$\begingroup$

No.

E.g., take $a=4$ and $b=27/8$. Then the positive roots of $g$ are $x_1\approx0.338$, $x_2\approx0.826$, $x_3\approx3.78$, and $f(x_1)\approx13.6$ and $f(x_3)\approx9.72$, so that $x_1$ is not a global minimizer of $f$ on $(0,\infty)$.

For an illustration, here are graphs of $f$ (blue) and $g$ (red):

enter image description here

Improve this answer
$\endgroup$
3
  • $\begingroup$ Thanks. I don't think $g$ has only one or two positive roots. $g$ can have $3$ positive roots, e.g. when $a=3,b=4$, as I mentioned in the question. So unfortunately, I don't think that your argument works as is, unless I am missing something. Indeed, if the roots of $g$ are $u<v<w$, then $u,w$ are both local minimizers of $f$, and we need to determine which one is the global minimizer. If I am not mistaken, $g$ can have either one positive root or three, so it's the case of three roots is the real one we should handle. $\endgroup$
    – Asaf Shachar
    Commented Jan 13, 2023 at 10:53
  • $\begingroup$ (If there are only two roots then $g$ tends to $-\infty$, at $x \to \infty$, no?). An example for three roots you can see plotted here: wolframalpha.com/input?i=plot+x%5E4-3x%5E3%2B4x-1 $\endgroup$
    – Asaf Shachar
    Commented Jan 13, 2023 at 10:53
  • $\begingroup$ @AsafShachar : Oops! I miscounted the roots; will have to learn to count to $3$. Now this is corrected, but the answer turns out to be negative. $\endgroup$
    – Iosif Pinelis
    Commented Jan 13, 2023 at 16:12

Not the answer you're looking for? Browse other questions tagged .