Are there general principles that allow us to easily determine whether coins in simple arrangements in a frame can move?

Question

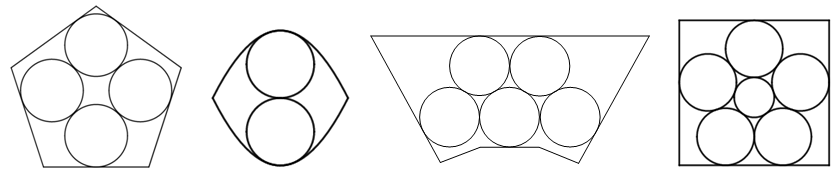
Circular coins in a frame may all be stuck in their positions; for example:

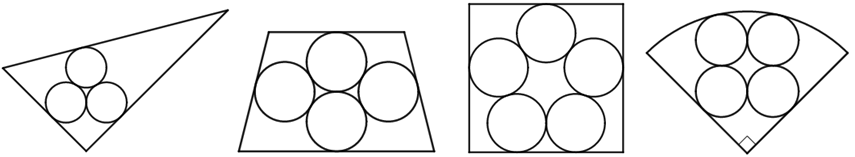
Another possibility is that they can all move simultaneously; I claim the following examples:

It is not always obvious that the coins can move, and it can be quite tedious, or nearly impossible, to show algebraically that the coins can move. We can use animations (example1, example2, example3), but that's not quite rigorous.

Are there general principles that allow us to easily determine whether coins in simple arrangements in a frame can all move?

Here are some of my attempts at formulating such general principles.

• Earlier I conjectured that if circular coins of any sizes are in a convex polygonal frame, with each coin touching exactly one edge, then all the coins can move. A counter-example, using coins of different sizes, was given.
• Then I proposed a second conjecture that was like the first one, except it required the coins to be equal size. A counter-example was given.
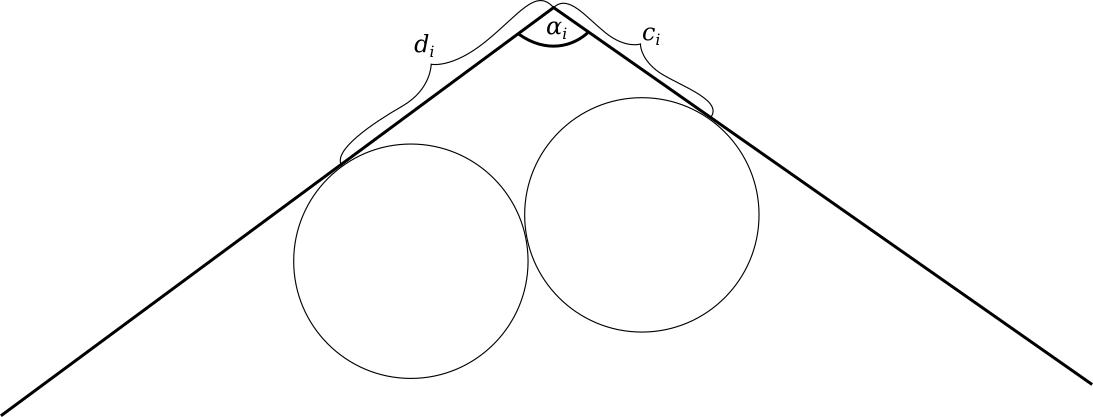
• My latest idea is to add the condition that the coins must be in a ring (i.e. every coin touches exactly two other coins), but I would not be surprised if there's yet another counter-example.

Anyway, I open to floor to general principles that are useful in determining if coins in simple arrangements in a frame can move.

(I have no strict definition of "simple arrangements". Let's say, just a few coins, and just a few sides and/or "natural" curves like circular arcs, parabolas, etc. I exclude arrangements in which a coin can move without disturbing others, and arrangements in which the coins can rotate as a single rigid body about a fixed point.)

Answer 1

With a ring configuration in a convex polygon and no coin touching two walls, they can always move.

Without loss of generality, assume that each wall has a coin on it. (Walls not touched by any coin have no impact to small movements and can thus be omitted, extending the polygon.) It is sufficient to consider movements along the walls. (If other movements are possible, the problem is trivial.)

The situation thus boils down to a chain of touching-points of the following appearance:

Proposition 1: $c_i$ and $d_i$ are in a strict-monotonically decreasing relationship to each other.
This is actually not true in general: specifically, for acute $\alpha_i$ we can have the situation where both coins move simultaneously further into the corner. This case can however be disregarded, because it immediately provides a solution, namely just move these two coins.

Lemma 2: $L_i := c_i+d_i$ is concave (considered as a function of either $c_i$ or $d_i$), regardless of $\alpha_i$.
The proof is left as an exercise.

Furthermore, in the degenerate case $\alpha_i=180^\circ$, $L_i$ is constant and thus still (non-strictly) concave; this covers the case that multiple coins touch the same wall.

Now consider the chain of coins, numbered from $i=0$ to $i=n-1$, to stay connected. $d_i + c_{i+1}$ is constant (namely the length of that side of the polygon); together with proposition 1 this implies that the movement of all the coins together can be parameterised equivalently by any of the $c_i$.

If I say “all coins”, we're treating the $i=0$-coin as split in two halves here, each of them touching only one other coin and still staying tangential to the wall. The distance between the halves of this split coin, considered as a function of moving the whole chain, is a constant (the polygon circumference) minus a sum of concave functions by lemma 2 (the $L_i$ contributions, using the fact that a concave function composed with a monotonically increasing one is still concave), and is therefore a convex function. Consequently, it is possible to move the whole chain in at least one direction without pressing the two halves of the $i=0$ coin into each other. Or equivalently, all coins (no splitting) can move in at least one direction without pressing two coins into each other.

---

I am almost sure that the condition of the polygon to be convex can be dropped: although $L_i$ fails to be concave at an impinging vertex, the other corners have to be all the more acute in this case and have therefore more strongly concave $L_i$. But it is more elaborate to show that the whole sum is concave then.