Space-time trajectory that cannot be straightened and its braid form

Question

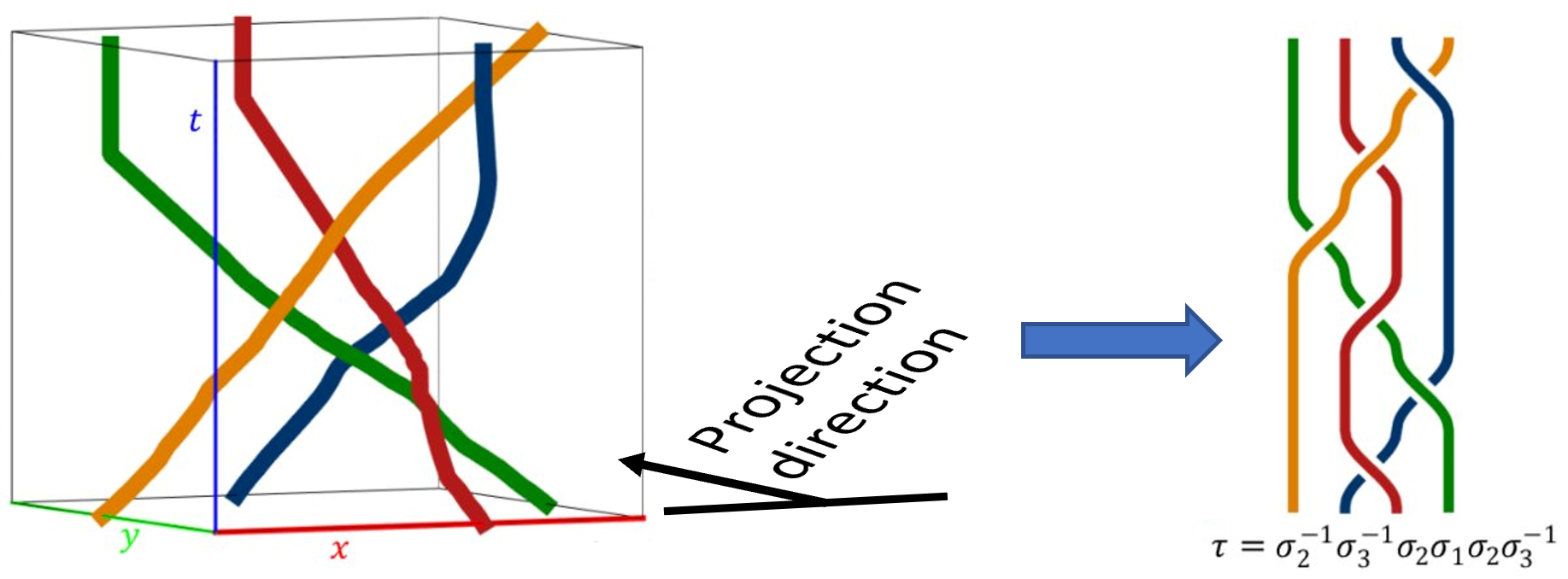
Considering we have the space-time trajectory of multiple particles (or any objects) in the X-Y-Time coordination system. Given a projection direction, we can obtain the braid form of the space-time trajectory. The figure below illustrates this idea (taken from the paper 'Social Momentum: A Framework for Legible Navigation in Dynamic Multi-Agent Environments' by C Mavrogiannis, etal).

Now, image the trajectory are strings and we can pull them tight while keeping their starting and ending positions unchanged (it is like applying elementary moves on the trajectories), we may or may not be able to straighten all the strings. By straightening the strings I mean making the string a straight line between the starting and ending points. Not able to straighten the strings means that some strings is in contact with at least one other strings. The radius (thickness) of string is neglected.

My first question is, for a space-time trajectory that cannot be straightened, does there always exist a a projection direction parallel to the X-Y plane, such that the corresponding braid form also cannot be straightened? The braid form is a standard form where the starting and ending point are evenly spaced. Braid cannot be straightened meaning that its strings cannot be all straight no matter how many elementary moves and reidemeister moves applied to it.

My second question is, given a braid that cannot be straightened, does there exist a point in time $t_{start}<t_1\leq t_{end}$, such that the braid formed during time $t_{start}$ to $t_{1}$ consists of some sub 2-braid or 3-braid that can be closed to form non-trivial knot/link? Sub 2-braid and 3-braid are braids formed when we only pick the particular 2 or 3 strings from the braid.

The intuition for the second question is, when a bent string in a braid is formed, it gets the bend from first interacting with either one or two other strings. Hence, if we extract the part of the braid from the start to the time when the first bend is formed, the 2 or 3 strings that interacted together should form a non-trivial knot/link.

The questions are long and maybe a bit difficult to understand at first. Really appreciate any input on any questions. Feel free to clarify anything as well. Thank you for reading!

EDIT: to give examples for the second question, the left braid in the figure below is straightenable, while the right braid is not. The right braid closes to be a non-trivial knot.

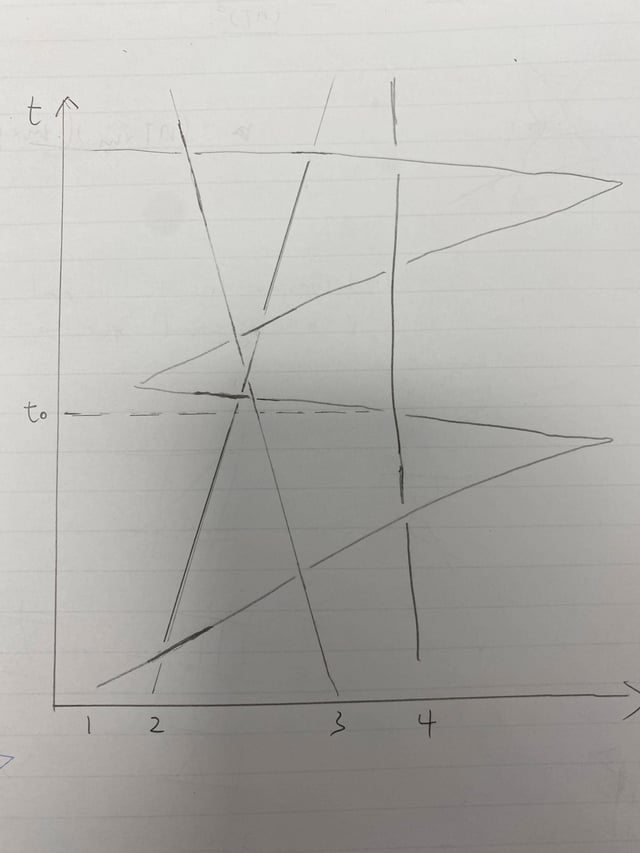
I have also taken a look at the Brunnian 4-braid, which is not straightenable, and it has a sub 2-braid formed from string 1 and 4 which gives a non-trivial knot at time $t_0$.

Answer 1

The answer to your second question is "no". Non-trivial braids can have braid closures yielding trivial knots (and links). As an example of this, consider the braid $\beta = \sigma_1 \sigma_2 \sigma_3$. To place this in three space, we use coordinates in $\mathbb{R}^3$. So, let $a = (0, 0, 0)$, $b = (1, 0, 0)$, $c = (2, 0, 0)$, and $d = (3, 0, 0)$. Let $a' = (0, 0, 1)$, $b' = (1, 0, 1)$, $c' = (2, 0, 1)$, and $d' = (3, 0, 1)$. We use these points as the upper and lower ends of the strands. Thus, the given braid in three-space cannot be straightened in three-space, as the four lines would all lie in a plane, and thus cross. The closure of any sub-braid, truncated at any height, of the usual projection (into the $xz$ plane) is an unlink.

Note that if you are willing to isotope the braid before truncation then the answer to the second question becomes "yes" in an uninteresting way. Thus is because a braid $\beta$ is isotopic to the braid $\beta \gamma \gamma^{-1}$ for any $\gamma$. You could then truncate the height to obtain $\beta \gamma$ which will have a non-trivial closure, if you so desire.

---

Note that truncating at an arbitrary (generic?) height and then taking a closure does not really make sense... To compose, or to close, braids we need the endpoints to be at predetermined locations. With $n$ strands, it is traditional to take the points $(i, 0, 0)$ (and $(i, 0, 1)$) as $i$ ranges from zero to $n-1$. If you really want to deal with physical braids whose endpoints are elsewhere, then I think that the algebra will need much more care.